In conservation laws, quantities are said to be “conserved,” and the conservation laws that result can be considered the most fundamental principles of mechanics. In mechanics, examples of conserved quantities are energy, momentum, and angular momentum. The laws of conservation are exact for an isolated system.
The Law of Conservation of Matter
The law of conservation of matter or principle of matter conservation states that the mass of an object or collection of objects never changes over time, no matter how the constituent parts rearrange themselves.
The mass can neither be created nor destroyed.
The law requires that during any nuclear reaction, radioactive decay, or chemical reaction in an isolated system, the total mass of the reactants or starting materials must be equal to the mass of the products.
See also: Law of Conservation of Matter
Law of Conservation of Energy
The law of energy conservation is one of the basic laws of physics, along with the conservation of mass and the conservation of momentum. The law of conservation of energy states that energy can change from one form into another, but it cannot be created or destroyed. Or the general definition is:
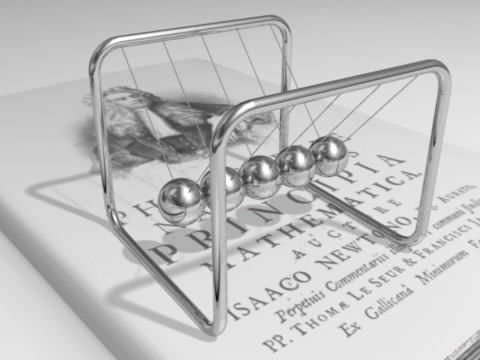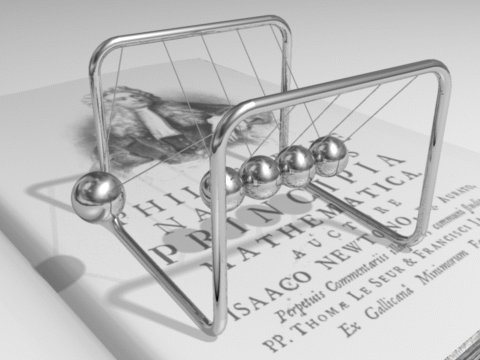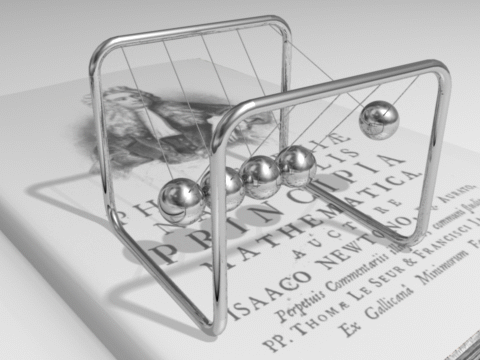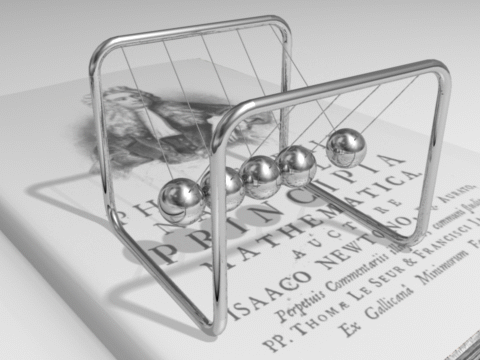
The total energy of an isolated system remains constant over time.
Energy can be defined as the capacity for doing work. It may exist in various forms and may be transformed from one type of energy to another in hundreds of ways.
See also: Law of Conservation of Energy.
The concept of energy conservation is widely used in many fields. In this article, the following fields are discussed:
- Conservation of Mechanical Energy
- Conservation of Energy in Fluid Mechanics
- Conservation of Energy in Thermodynamics
- Conservation of Energy in Electrical Circuits
- Conservation of Energy in Chemical Reactions
- Conservation of Energy in Special Relativity Theory
- Conservation of Energy in Nuclear Reactions
Law of Conservation of Mass-Energy – Mass-Energy Equivalence
In the special theory of relativity, certain types of matter may be created or destroyed. Still, the mass and energy associated with such matter remain unchanged in quantity in all of these processes. It was found the rest mass of an atomic nucleus is measurably smaller than the sum of the rest masses of its constituent protons, neutrons, and electrons. Mass was no longer considered unchangeable in the closed system. The difference is a measure of the nuclear binding energy which holds the nucleus together. According to the Einstein relationship (E = mc2), this binding energy is proportional to this mass difference, known as the mass defect.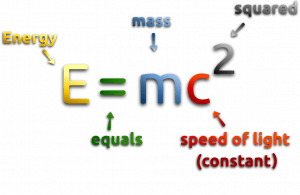
Law of Conservation of Momentum
In general, the law of conservation of momentum or principle of momentum conservation states that the momentum of an isolated system is a constant. The vector sum of the momenta (momentum is equal to the mass of an object multiplied by its velocity) of all the objects of a system cannot be changed by interactions within the system. In classical mechanics, this law is implied by Newton’s laws. This principle is a direct consequence of Newton’s third law.
See also: Law of Conservation of Momentum
Laws of Conservation in Nuclear Reactions
In analyzing nuclear reactions, we apply the many conservation laws. Nuclear reactions are subject to classical conservation laws for the charge, momentum, angular momentum, and energy (including rest energies). Not anticipated by classical physics, other conservation laws are electric charge, lepton number, and baryon number. Certain of these laws are obeyed under all circumstances. Others are not. We have accepted the conservation of energy and momentum. In all the examples given, we assume that the number of protons and neutrons are separately conserved. We shall find circumstances and conditions in which this rule is not true. Where we are considering non-relativistic nuclear reactions, it is essentially true. However, we shall find that these principles must be extended when we consider relativistic nuclear energies or those involving weak interactions.