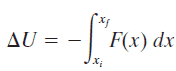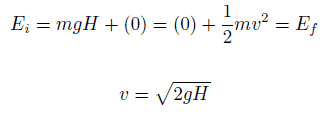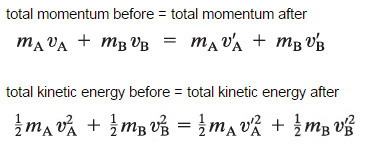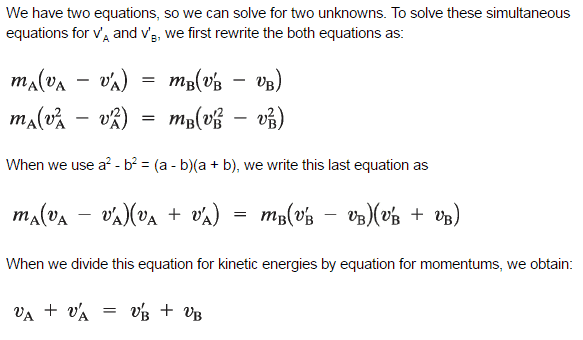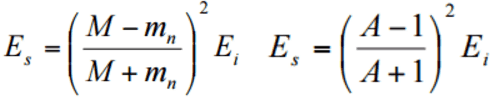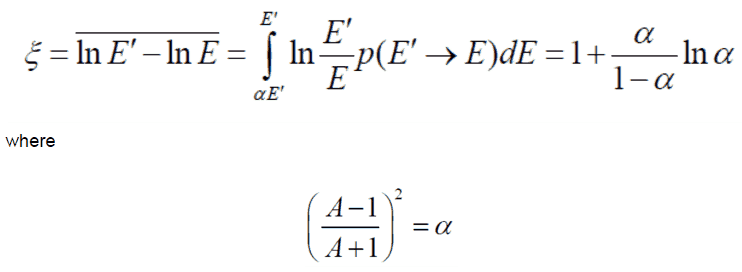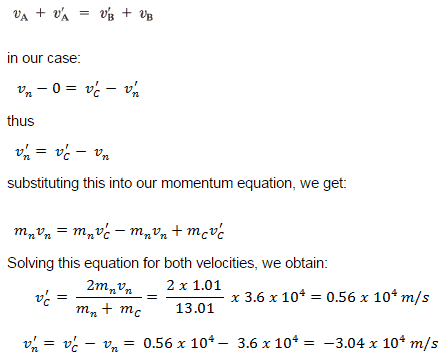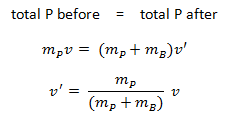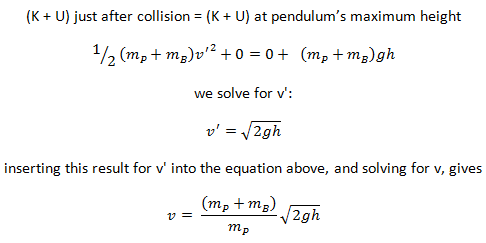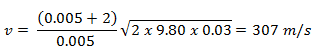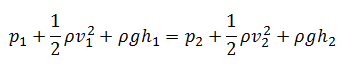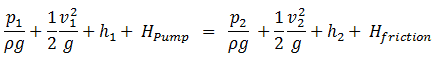Or the general definition is:
The total energy of an isolated system remains constant over time.
Energy can be defined as the capacity for doing work. It may exist in various forms and be transformed from one type of energy to another in hundreds of ways.
For example, burning gasoline to power cars is an energy conversion process we rely on. The chemical energy in gasoline is converted to thermal energy, then converted to mechanical energy that makes the car move. The mechanical energy has been converted to kinetic energy. When we use the brakes to stop a car, that kinetic energy is converted by friction back to heat or thermal energy.
A consequence of the law of conservation of energy is that a perpetual motion machine of the first kind, which produces work without the input of energy, cannot exist.
The concept of energy conservation is widely used in many fields. In this article, the following fields are discussed:
- Conservation of Mechanical Energy
- Conservation of Energy in Fluid Mechanics
- Conservation of Energy in Thermodynamics
- Conservation of Energy in Electrical Circuits
- Conservation of Energy in Chemical Reactions
- Conservation of Energy in Special Relativity Theory
- Conservation of Energy in Nuclear Reactions
Law of Conservation of Energy in Classical Physics
Conservation of Mechanical Energy
First, the principle of the Conservation of Mechanical Energy was stated:
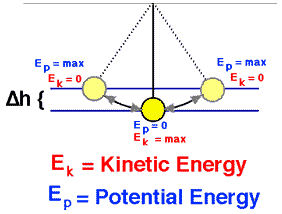
The total mechanical energy (defined as the sum of its potential and kinetic energies) of a particle being acted on by only conservative forces is constant.
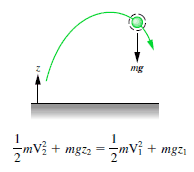An isolated system is one in which no external force causes energy changes. If only conservative forces act on an object and U is the potential energy function for the total conservative force, then:
Emech = U + K
The potential energy, U, depends on the position of an object subjected to a conservative force.
It is defined as the object’s ability to do work and is increased as the object is moved in the opposite direction of the force.
The potential energy associated with a system consisting of Earth and a nearby particle is gravitational potential energy.
The kinetic energy, K, depends on the speed of an object and is the ability of a moving object to do work on other objects when it collides with them.
K = ½ mv2
The definition mentioned above (Emech = U + K) assumes that the system is free of friction and other non-conservative forces. The difference between a conservative and a non-conservative force is that when a conservative force moves an object from one point to another, the work done by the conservative force is independent of the path.
In any real situation, frictional forces and other non-conservative forces are present. Still, their effects on the system are so small that the principle of conservation of mechanical energy can be used as a fair approximation in many cases. For example, the frictional force is a non-conservative force because it acts to reduce the mechanical energy in a system.
Note that non-conservative forces do not always reduce mechanical energy. A non-conservative force changes the mechanical energy. Some forces increase the total mechanical energy, like the force provided by a motor or engine, which is also a non-conservative force.
Assume a pendulum (ball of mass m suspended on a string of length L that we have pulled up so that the ball is a height H < L above its lowest point on the arc of its stretched string motion. The pendulum is subjected to the conservative gravitational force where frictional forces like air drag and friction at the pivot are negligible.
We release it from rest. How fast is it going at the bottom?
The pendulum reaches the greatest kinetic energy and least potential energy when in the vertical position because it will have the greatest speed and be nearest the Earth at this point. On the other hand, it will have its least kinetic energy and greatest potential energy at the extreme positions of its swing because it has zero speed and is farthest from Earth at these points.
If the amplitude is limited to small swings, the period T of a simple pendulum, the time is taken for a complete cycle, is:
where L is the length of the pendulum and g is the local acceleration of gravity. For small swings, the period of swing is approximately the same for different size swings. That is, the period is independent of amplitude.
The Law of Conservation of Energy – Non-conservative Forces
We now take into account non-conservative forces such as friction since they are important in real situations. For example, consider the pendulum again, but this time let us include air resistance. The pendulum will slow down because of friction. In this and other natural processes, the mechanical energy (sum of the kinetic and potential energies) does not remain constant but decreases. Because frictional forces reduce the mechanical energy (but not the total energy), they are called non-conservative forces (or dissipative forces). But in the nineteenth century, it was demonstrated the total energy is conserved in any process. In the case of the pendulum, its initial kinetic energy is all transformed into thermal energy.
For each type of force, conservative or non-conservative, it has always been found possible to define a type of energy that corresponds to the work done by such a force. And it has been found experimentally that the total energy E always remains constant. The general law of conservation of energy can be stated as follows:
The total energy E of a system (the sum of its mechanical energy and its internal energies, including thermal energy) can change only by amounts of energy transferred to or from the system.
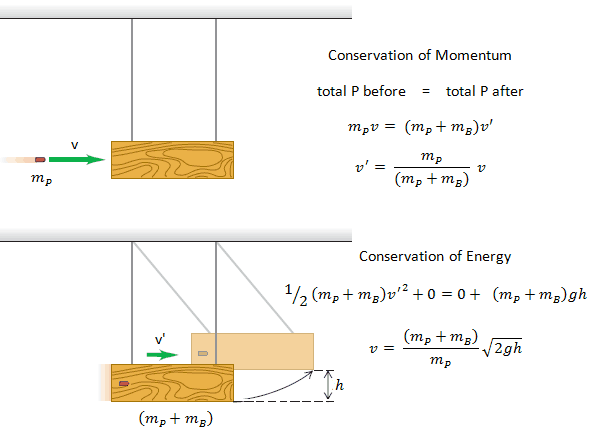
Conservation of Momentum and Energy in Collisions
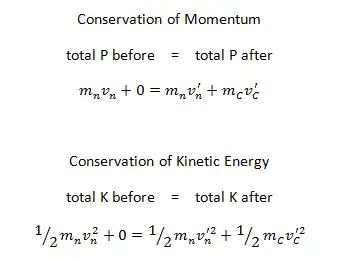
The use of the conservation laws for momentum and energy is also very important in particle collisions. This is a very powerful rule because it can allow us to determine the results of a collision without knowing the details of the collision. The law of conservation of momentum states that the total momentum is conserved in the collision of two objects such as billiard balls. The assumption of conservation of momentum and the conservation of kinetic energy makes possible the calculation of the final velocities in two-body collisions. At this point, we have to distinguish between two types of collisions:
- Elastic collisions
- Inelastic collisions
Elastic Collisions
A perfectly elastic collision is defined as one in which there is no net conversion of kinetic energy into other forms (such as heat or noise). For the brief moment during which the two objects are in contact, some (or all) of the energy is stored momentarily in the form of elastic potential energy. But if we compare the total kinetic energy just before the collision with the total kinetic energy just after the collision, they are found to be the same. We say that the total kinetic energy is conserved.
- Large-scale interactions between satellites and planets are perfectly elastic, like the slingshot type gravitational interactions (also known as a planetary swing-by or a gravity-assist maneuver).
- Collisions between very hard spheres may be nearly elastic, so it is useful to calculate the limiting case of an elastic collision.
- Collisions in ideal gases approach perfectly elastic collisions, as do scattering interactions of sub-atomic particles, deflected by the electromagnetic force.
- Rutherford scattering is the elastic scattering of charged particles also by the electromagnetic force.
- A neutron-nucleus scattering reaction may also be elastic, but in this case, the neutron is deflected by a strong nuclear force.
The relative speed of the two objects after the collision has the same magnitude (but opposite direction) as before the collision, no matter what the masses are.
It is known the fission neutrons are of importance in any chain-reacting system. All neutrons produced by fission are born as fast neutrons with high kinetic energy. Before such neutrons can efficiently cause additional fissions, they must be slowed down by collisions with nuclei in the reactor’s moderator. The probability of the fission U-235 becomes very large at the thermal energies of slow neutrons. This fact implies an increase in the multiplication factor of the reactor (i.e., lower fuel enrichment is needed to sustain a chain reaction).
The neutrons released during fission with an average energy of 2 MeV in a reactor on average undergo many collisions (elastic or inelastic) before they are absorbed. During the scattering reaction, a fraction of the neutron’s kinetic energy is transferred to the nucleus. It is possible to derive the following equation for the mass of target or moderator nucleus (M), the energy of incident neutron (Ei), and the energy of scattered neutron (Es) using the laws of conservation of momentum and energy and the analogy of collisions of billiard balls for elastic scattering.
where A is the atomic mass number, in case of the hydrogen (A = 1) as the target nucleus, the incident neutron can be completely stopped. But this works when the direction of the neutron is completely reversed (i.e., scattered at 180°). In reality, the direction of scattering ranges from 0 to 180 °, and the energy transferred also ranges from 0% to maximum. Therefore, the average energy of the scattered neutron is taken as the average of energies with scattering angles 0 and 180°.
Moreover, it is useful to work with logarithmic quantities. Therefore one defines the logarithmic energy decrement per collision (ξ) as a key material constant describing energy transfers during a neutron slowing down. ξ is not dependent on energy, only on A and is defined as follows:
For heavy target nuclei, ξ may be approximated by the following formula:
From these equations, it is easy to determine the number of collisions required to slow down a neutron from, for example from 2 MeV to 1 eV.
Example: Determine the number of collisions required for thermalization for the 2 MeV neutrons in the carbon.
ξCARBON = 0.158
N(2MeV → 1eV) = ln 2⋅106/ξ =14.5/0.158 = 92
For a mixture of isotopes:
What are the velocities of the neutron and carbon nucleus after the collision?
Solution:
This is an elastic head-on collision of two objects with unequal masses. We have to use the conservation laws of momentum and kinetic energy and apply them to our system of two particles.
We can solve this system of equations or use the equation derived in the previous section. This equation stated that the relative speed of the two objects after the collision has the same magnitude (but opposite direction) as before the collision, no matter what the masses are.
The minus sign for v’ tells us that the neutron scatters the back of the carbon nucleus because the carbon nucleus is significantly heavier. On the other hand, its speed is less than its initial speed. This process is known as neutron moderation, and it significantly depends on the mass of moderator nuclei.
Inelastic Collisions
An inelastic collision is when part of the kinetic energy is changed to some other form of energy in the collision. Any macroscopic collision between objects will convert some kinetic energy into internal energy and other forms of energy, so no large-scale impacts are perfectly elastic. For example, in collisions of common bodies, such as two cars, some energy is always transferred from kinetic energy to other forms of energy, such as thermal energy or energy of sound. The inelastic collision of two bodies always involves a loss in the kinetic energy of the system. The greatest loss occurs if the bodies stick together, in which case the collision is called a completely inelastic collision. Thus, the system’s kinetic energy is not conserved, while the total energy is conserved as required by the general principle of conservation of energy. Momentum is conserved in inelastic collisions, but one cannot track the kinetic energy through the collision since some of it is converted to other forms of energy.
In nuclear physics, an inelastic collision is when the incoming particle causes the nucleus to strike to become excited or break up. Deep inelastic scattering is a method of probing the structure of subatomic particles in much the same way as Rutherford probed the inside of the atom (see Rutherford scattering).
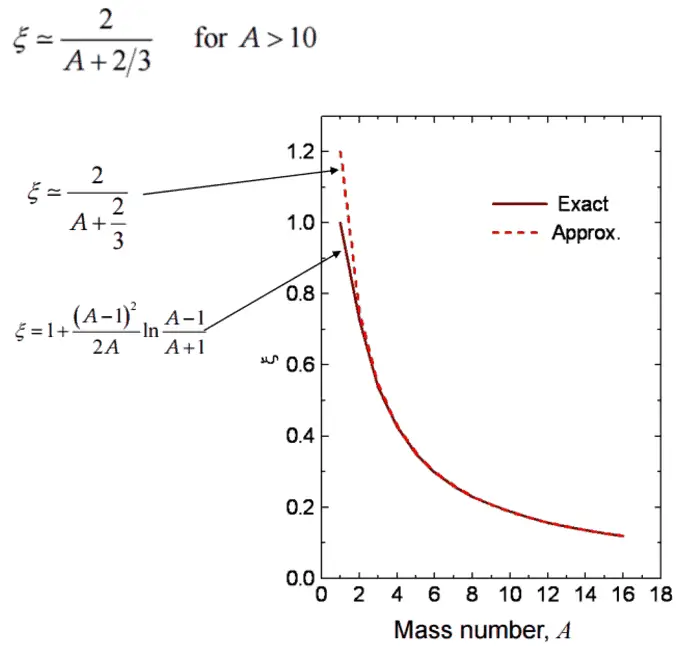
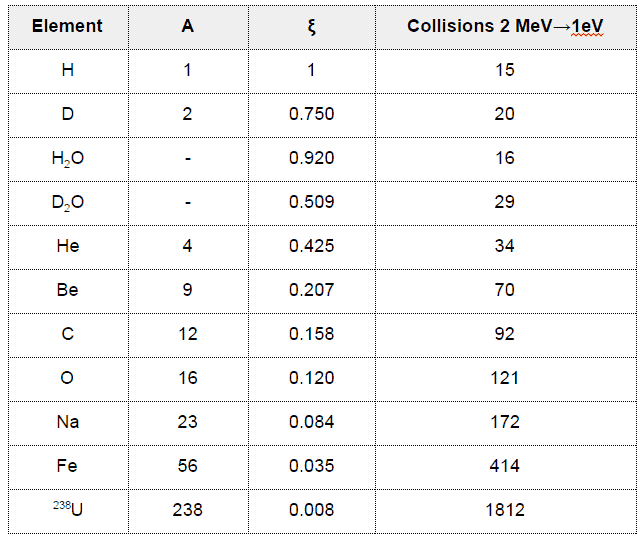
In nuclear reactors, inelastic collisions are of importance in the neutron moderation process. An inelastic scattering plays an important role in slowing down neutrons, especially at high energies and by heavy nuclei. Inelastic scattering occurs above threshold energy. This threshold energy is higher than the energy of the first excited state of the target nucleus (due to the laws of conservation), and it is given by the following formula:
Et = ((A+1)/A)* ε1
where Et is the inelastic threshold energy, and ε1 is the energy of the first excited state. Therefore, especially scattering data of 238U, a major component of nuclear fuel in commercial power reactors, is one of the most important data in the neutron transport calculations in the reactor core.
A ballistic pendulum is a device for measuring the velocity of a projectile, such as a bullet. The ballistic pendulum is a kind of “transformer,” exchanging the high speed of a light object (the bullet) for the low speed of a massive object (the block). When a bullet is fired into the block, its momentum is transferred to the block. The bullet’s momentum can be determined from the amplitude of the pendulum swing.
When the bullet is embedding itself in the block, it occurs so quickly that the block does not move appreciably. The supporting strings remain nearly vertical, so negligible external horizontal force acts on the bullet–block system, and the horizontal component of momentum is conserved. However, mechanical energy is not conserved during this stage because a non-conservative force does work (the force of friction between bullet and block).
In the second stage, the bullet and block move together. The only forces acting on this system are gravity (a conservative force) and string tensions (which do not work). Thus, as the block swings, mechanical energy is conserved. Momentum is not conserved during this stage because there is a net external force (the forces of gravity and string tension don’t cancel when the strings are inclined).
Equations governing the ballistic pendulum
In the first stage, momentum is conserved, and therefore:
where v is the initial velocity of the projectile of mass mP. v’ is the velocity of the block and embedded projectile (both of mass mP + mB) just after the collision, before they have moved significantly.
In the second stage, mechanical energy is conserved. We choose y = 0 when the pendulum hangs vertically and then y = h when the block and embedded projectile system reach their maximum height. The system swings up and comes to rest for an instant at a height y, where its kinetic energy is zero, and the potential energy is (mP + mB)gh. Thus we write the law of conservation of energy:
which is the initial velocity of the projectile and our final result.
When we use some realistic numbers:
- mP = 5 g
- mB = 2 kg
- h = 3 cm
- v = ?
then we have:
Conservation of Energy in Fluid Mechanics – Bernoulli’s Principle
The law of conservation of energy can also be used in the analysis of flowing fluids.
Bernoulli’s equation can be considered a statement of the conservation of energy principle appropriate for flowing fluids. It is one of the most important/useful equations in fluid mechanics. It puts into a relation pressure and velocity in an inviscid incompressible flow. The general energy equation is simplified to:
This equation is the most famous in fluid dynamics. Bernoulli’s equation describes the qualitative behavior flowing fluid that is usually labeled with Bernoulli’s effect. This effect causes the lowering of fluid pressure in regions where the flow velocity is increased. This lowering of pressure in a constriction of a flow path may seem counterintuitive but seems less so when you consider the pressure to be energy density. In the high-velocity flow through the constriction, kinetic energy must increase at the expense of pressure energy. The dimensions of terms in the equation are kinetic energy per unit volume. The equation above assumes that no non-conservative forces (e.g.,, friction forces) act on the fluid. This is a very strong assumption.
Extended Bernoulli’s equation
Bernoulli’s equation can be modified to take into account gains and losses of the head caused by external forces and non-conservative forces. The resulting equation referred to as the extended Bernoulli’s equation is useful in solving most fluid flow problems. The following equation is one form of the extended Bernoulli equation.
where:
- h = height above reference level (m)
- v = average velocity of fluid (m/s)
- p = pressure of fluid (Pa)
- Hpump = head added by pump (m)
- Hfriction = head loss due to fluid friction (m)
- g = acceleration due to gravity (m/s2)
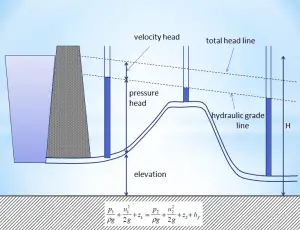
The head loss (or the pressure loss) due to fluid friction (Hfriction) represents the energy used in overcoming friction caused by the pipe walls. The head loss that occurs in pipes is dependent on the flow velocity, pipe diameter, and length, and a friction factor based on the roughness of the pipe and the Reynolds number of the flow. A piping system containing many pipe fittings and joints, tube convergence, divergence, turns, surface roughness, and other physical properties will also increase the head loss of a hydraulic system.
Although the head loss represents a loss of energy, it does not represent a loss of total energy of the fluid. The total energy of the fluid is conserved as a consequence of the law of conservation of energy. In reality, the head loss due to friction results in an equivalent increase in the internal energy (increase in temperature) of the fluid.
This phenomenon can also be seen in the case of reactor coolant pumps. Generally, reactor coolant pumps are very powerful, they can consume up to 6 MW each, and therefore they can be used for heating the primary coolant before a reactor startup, for example, from 30°C at cold zero power (CZP) to 290°C at hot zero power (HZP).
Conservation of Energy in Thermodynamics – The First Law of Thermodynamics
In thermodynamics, the concept of energy is broadened to account for other observed changes. The principle of conservation of energy is extended to include a wide variety of ways systems interact with their surroundings. The only ways the energy of a closed system can be changed are through the transfer of energy by work or by heat. Further, based on the experiments of Joule and others, a fundamental aspect of the energy concept is that energy is conserved. This principle is known as the first law of thermodynamics. The first law of thermodynamics can be written in various forms:
In words:
Equation form:
∆Eint = Q – W
where Eint represents the internal energy of the material, which depends only on the material’s state (temperature, pressure, and volume), Q is the net heat added to the system, and W is the net work done by the system. We must be careful and consistent in following the sign conventions for Q and W. Because W in the equation is the work done by the system, then if work is done on the system, W will be negative, and Eint will increase.
Similarly, Q is positive for heat added to the system, so Q is negative if heat leaves the system. This tells us the following: The internal energy of a system tends to increase if heat is absorbed by the system or if positive work is done on the system. Conversely, the internal energy tends to decrease if heat is lost by the system or if negative work is done on the system. It must be added Q and W are path-dependent, while Eint is path-independent.
Differential form:
dEint = dQ – dW
The internal energy Eint of a system tends to increase if energy is added as heat Q and tends to decrease if energy is lost as work W is done by the system.
Open System – Closed System – Isolated System
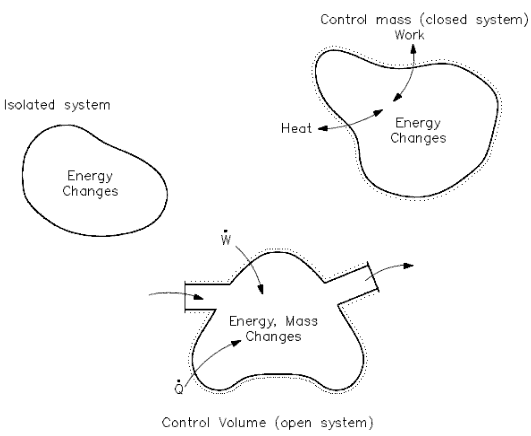Heat and/or work can be directed into or out of the control volume. But, for convenience and as a standard convention, the net energy exchange is presented here, with the net heat exchange assumed to be into the system and the network assumed to be out of the system. If no mass crosses the boundary, but work and/or heat do, the system is referred to as a “closed” system. If mass, work, and heat do not cross the boundary (that is, the only energy exchanges taking place are within the system), the system is referred to as an isolated system. Isolated and closed systems are nothing more than specialized cases of the open system.
Conservation of Energy in Electrical Circuits
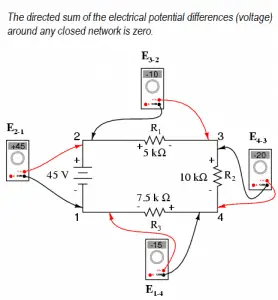Kirchhoff’s voltage law (KVL)
The law of conservation of energy can also be used in the analysis of electrical circuits. In the analysis of electrical circuits, the principle of energy conservation provides the basis for the law, known as Kirchhoff’s voltage law (or Kirchhoff’s second law), after German physicist Gustav Robert Kirchhoff.
Kirchhoff’s voltage law states:
The algebraic sum of the voltages (drops or rises) encountered in traversing any circuit loop in a specified direction must be zero.
The algebraic sum of the voltages (drops or rises) encountered in traversing any circuit loop in a specified direction must be zero.
Simply, the voltage changes around any closed loop must sum to zero. The sum of the voltage rises is equal to the sum of the voltage drops in a loop. No matter what path you take through an electric circuit, if you return to your starting point, you must measure the same voltage, constraining the net change around the loop to be zero.
Since voltage is electric potential energy per unit charge, the voltage law can be a consequence of the conservation of energy. This rule is equivalent to saying that each point on a mountain has only one elevation above sea level. If you start from any point and return to it after walking around the mountain, the algebraic sum of the elevation changes that you encounter must be zero.
The voltage law has great practical utility in the analysis of electric circuits. It is used in conjunction with the current law in many circuit analysis tasks.
Conservation of Energy in Chemical Reactions
The concept of energy conservation is also widely used in chemistry. Chemical reactions are determined by the laws of thermodynamics. In thermodynamics, the internal energy of a system is the energy contained within the system, excluding the kinetic energy of motion of the system as a whole and the potential energy of the system as a whole due to external force fields.
In thermodynamics, the internal energy includes the translational kinetic energy of the molecules (in the case of gases), the kinetic energy due to rotation of the molecules relative to their centers of mass, and the kinetic energy associated with vibrational motions within the molecules.
In chemical reactions, energy is stored in the chemical bonds between the atoms that make up the molecules. Energy storage on the atomic level includes energy associated with electron orbital states. Whether a chemical reaction absorbs or releases energy, there is no overall change in the amount of energy during the reaction. That’s because of the law of conservation of energy, which states that:
Energy cannot be created or destroyed. Energy may change form during a chemical reaction.
For example, energy may change from chemical energy to heat energy when gas burns in a furnace. The exact amount of energy remains after the reaction as before. This is true of all chemical reactions.
In an endothermic reaction, the products have more stored chemical energy than the reactants. In an exothermic reaction, the opposite is true. The products have less stored chemical energy than the reactants. The excess energy is generally released to the surroundings when the reaction occurs.
Example: Combustion of Hydrogen
Consider the combustion of hydrogen in air. In a flame of pure hydrogen gas burning in the air, the hydrogen (H2) reacts with oxygen (O2) to form water (H2O) and releases energy.
Energetically, the process can require the energy to dissociate the H2 and O2, but then the bonding of the H2O returns the system to a bound state with negative potential. It is more negative than the bound states of the reactants, and the formation of the two water molecules is, therefore, an exothermic reaction, which releases 5.7 eV of energy.
2H2(g) + O2(g) → 2H2O(g)
The energy balance before and after the reaction can be illustrated schematically with the state in which all atoms are free taken as the reference for energy.
Law of Conservation of Mass-Energy – Mass-Energy Equivalence
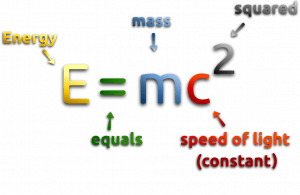At the beginning of the 20th century, the notion of mass underwent a radical revision. The mass lost its absoluteness. One of the striking results of Einstein’s theory of relativity is that mass and energy are equivalent and convertible one into the other. Equivalence of the mass and energy is described by Einstein’s famous formula E = mc2. In other words, energy equals mass multiplied by the speed of light squared. Because the speed of light is a very large number, the formula implies that any small amount of matter contains a very large amount of energy. The mass of an object was seen as equivalent to energy, interconvertible with energy, and increasing significantly at exceedingly high speeds near that of light. The total energy of an object was understood to comprise its rest mass and its increase of mass caused by an increase in kinetic energy.
In the special theory of relativity, certain types of matter may be created or destroyed. Still, the mass and energy associated with such matter remain unchanged in quantity in all of these processes. It was found the rest mass of an atomic nucleus is measurably smaller than the sum of the rest masses of its constituent protons, neutrons, and electrons. Mass was no longer considered unchangeable in the closed system. The difference is a measure of the nuclear binding energy which holds the nucleus together. According to the Einstein relationship (E = mc2), this binding energy is proportional to this mass difference, known as the mass defect.
Source: hyperphysics.phy-astr.gsu.edu
During the nuclear splitting or nuclear fusion, some of the mass of the nucleus gets converted into huge amounts of energy. Thus this mass is removed from the total mass of the original particles, and the mass is missing in the resulting nucleus. The nuclear binding energies are enormous, and they are a million times greater than the electron binding energies of atoms.
Generally, in both chemical and nuclear reactions, some conversion between rest mass and energy occurs so that the products generally have smaller or greater mass than the reactants. Therefore the new conservation principle is the conservation of mass energy.
See also: Energy Release from Fission
Conservation of Energy in Nuclear Reactions
In analyzing nuclear reactions, we have to apply the general law of conservation of mass energy. According to this law, mass and energy are equivalent and convertible one into the other. It is one of the striking results of Einstein’s theory of relativity. This equivalence of mass and energy is described by Einstein’s famous formula E = mc2.
Generally, in both chemical and nuclear reactions, some conversion between rest mass and energy occurs so that the products generally have smaller or greater mass than the reactants. In general, the total (relativistic) energy must be conserved. The “missing” rest mass must therefore reappear as kinetic energy released in the reaction. The difference is a measure of the nuclear binding energy which holds the nucleus together.
The nuclear binding energies are enormous, and they are a million times greater than the electron binding energies of atoms.
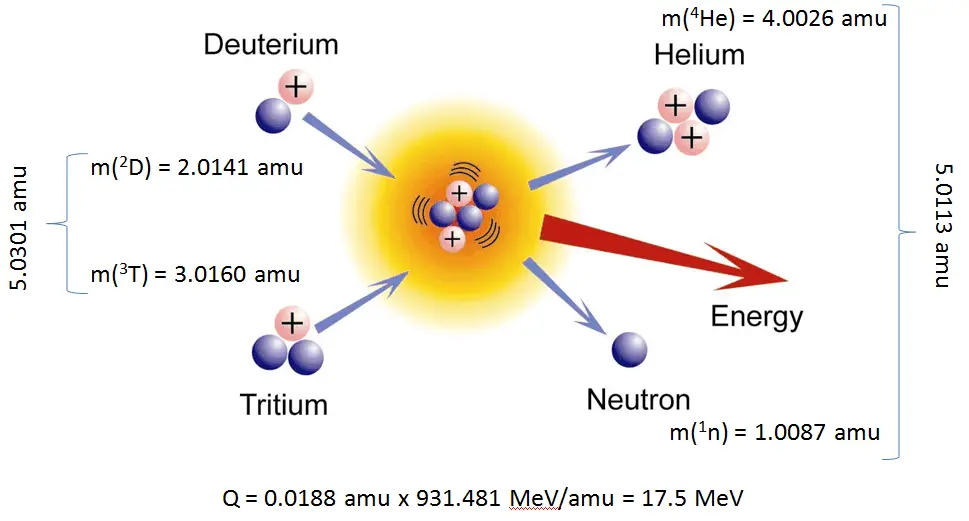
The energetics of nuclear reactions is determined by the Q-value of that reaction. The Q-value of the reaction is defined as the difference between the sum of the masses of the initial reactants and the sum of the masses of the final products in energy units (usually in MeV).
Consider a typical reaction in which the projectile a and target A place to two products, B and b. This can also be expressed in the notation we have used so far, a + A → B + b, or even in a more compact notation, A(a,b)B.
See also: E=mc2
The Q-value of this reaction is given by:
Q = [ma + mA – (mb + mB)]c2
which is the same as the excess kinetic energy of the final products:
Q = Tfinal – Tinitial
= Tb + TB – (Ta + TA)
For reactions in which there is an increase in the kinetic energy of the products, Q is positive. The positive Q reactions are said to be exothermic (or exergic). There is a net release of energy since the kinetic energy of the final state is greater than the kinetic energy of the initial state.
For reactions in which there is a decrease in the kinetic energy of the products, Q is negative. The negative Q reactions are endothermic (or endoergic), and they require net energy input.
See also: Q-value Calculator.
The DT fusion reaction of deuterium and tritium is particularly interesting because of its potential of providing energy for the future. Calculate the reaction Q-value.
3T (d, n) 4He
The atom masses of the reactants and products are:
m(3T) = 3.0160 amu
m(2D) = 2.0141 amu
m(1n) = 1.0087 amu
m(4He) = 4.0026 amu
Using the mass-energy equivalence, we get the Q-value of this reaction as:
Q = {(3.0160+2.0141) [amu] – (1.0087+4.0026) [amu]} x 931.481 [MeV/amu]
= 0.0188 x 931.481 = 17.5 MeV
A high-energy photon (gamma-ray) can, under certain conditions, eject a neutron from a nucleus. It occurs when its energy exceeds the binding energy of the neutron in the nucleus. Most nuclei have binding energies above 6 MeV, above the energy of most gamma rays from fission. On the other hand, few nuclei with sufficiently low binding energy are of practical interest. These are 2D, 9Be, 6Li, 7Li, and 13C. As can be seen from the table, the lowest threshold have 9Be with 1.666 MeV and 2D with 2.226 MeV.
threshold energies.
In the case of deuterium, neutrons can be produced by the interaction of gamma rays (with a minimum energy of 2.22 MeV) with deuterium:
The reaction Q-value is calculated below:
The atom masses of the reactant and products are:
m(2D) = 2.01363 amu
m(1n) = 1.00866 amu
m(1H) = 1.00728 amu
Using the mass-energy equivalence, we get the Q-value of this reaction as:
Q = {2.01363 [amu] – (1.00866+1.00728) [amu]} x 931.481 [MeV/amu]
= -0.00231 x 931.481 = -2.15 MeV
- The kinetic energy of the products
- Emission of gamma rays. Gamma rays are emitted by unstable nuclei in their transition from a high-energy state to a lower state known as gamma decay.
- Metastable state. Some energy may remain in the nucleus as a metastable energy level.
A small amount of energy may also emerge in the form of X-rays. Generally, products of nuclear reactions may have different atomic numbers, and thus the configuration of their electron shells is different in comparison with reactants. As the electrons rearrange themselves and drop to lower energy levels, internal transition X-rays (X-rays with precisely defined emission lines) may be emitted.
Energy Conservation in Nuclear Fission
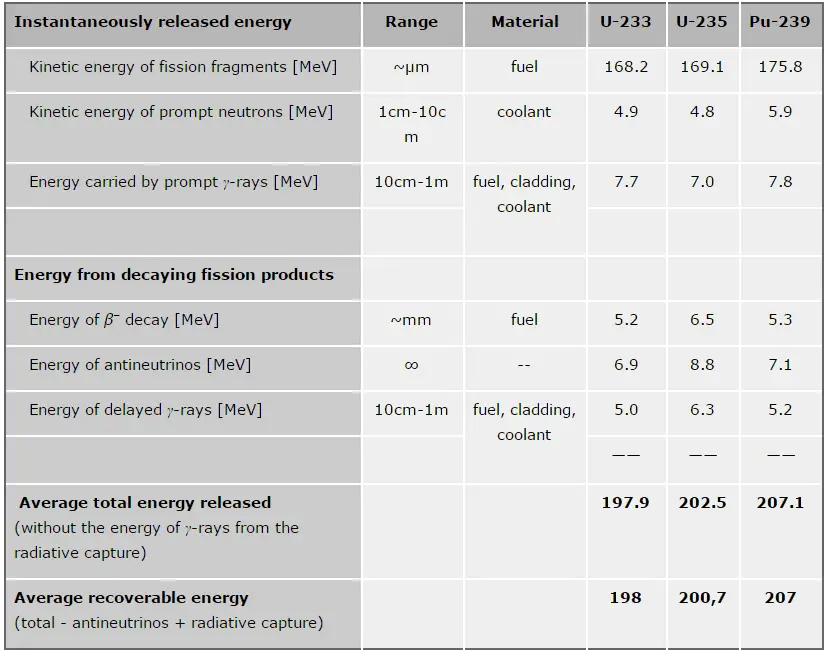In general, nuclear fission results in the release of enormous quantities of energy. The amount of energy depends strongly on the nucleus to be fissioned and also depends strongly on the kinetic energy of an incident neutron. It is necessary to identify the individual components of this energy precisely to calculate the power of a reactor. At first, it is important to distinguish between the total energy released and the energy that can be recovered in a reactor.
The total energy released in fission can be calculated from binding energies of the initial target nucleus to be fissioned and binding energies of fission products. But not all the total energy can be recovered in a reactor. For example, about 10 MeV is released in the form of neutrinos (in fact, antineutrinos). Since the neutrinos are weakly interacting (with an extremely low cross-section of any interaction), they do not contribute to the energy that can be recovered in a reactor.
See also: Energy Release from Fission.
Energy Conservation in Beta Decay – Discovery of the Neutrino
Beta-decay (β-decay) is a radioactive decay in which a beta particle and a respective neutrino are emitted from an atomic nucleus. Beta radiation consists of high-energy beta particles. High-speed electrons or positrons are emitted during beta decay. By beta decay emission, a neutron is transformed into a proton by the emission of an electron. Conversely, a proton is converted into a neutron by the emission of a positron, thus changing the nuclide type.
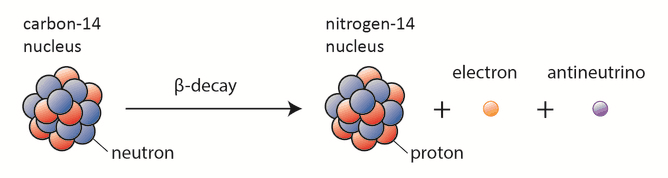
Discovery of the Neutrino
The study of beta decay provided the first physical evidence for the existence of the neutrino. The discovery of the neutrino is based on the law of conservation of energy during the process of beta decay.
The resulting particle (alpha particle or photon) has a narrow energy distribution in both alpha and gamma decay. The particle carries the energy from the difference between the initial and final nuclear states. For example, in the case of alpha decay, when a parent nucleus breaks down spontaneously to yield a daughter nucleus and an alpha particle, the sum of the mass of the two products does not quite equal the mass of the original nucleus (see Mass Defect). As a result of the law of conservation of energy, this difference appears in the form of the kinetic energy of the alpha particle. Since the same particles appear as products at every breakdown of a particular parent nucleus, the mass difference should always be the same, and the kinetic energy of the alpha particles should also always be the same. In other words, the beam of alpha particles should be monoenergetic.
It was expected that the same considerations would hold for a parent nucleus breaking down to a daughter nucleus and a beta particle. Because only the electron and the recoiling daughter nucleus were observed beta decay, the process was initially assumed to be a two-body process, very much like alpha decay. It would seem reasonable to suppose that the beta particles would also form a monoenergetic beam.
To demonstrate the energetics of two-body beta decay, consider the beta decay in which an electron is emitted and the parent nucleus rest. Conservation of energy requires:
Since the electron is a much lighter particle, it was expected that it would carry away most of the released energy, which would have a unique value Te-.
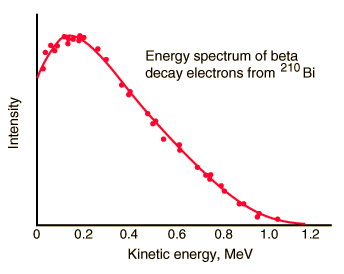
But the reality was different. However, the spectrum of beta particles measured by Lise Meitner and Otto Hahn in 1911 and by Jean Danysz in 1913 showed multiple lines on a diffuse background. Moreover, virtually all of the emitted beta particles have energies below predicted by energy conservation in two-body decays. The electrons emitted in beta decay have a continuous rather than a discrete spectrum that appeared to contradict conservation of energy, under the then-current assumption that beta decay is the simple emission of an electron from a nucleus. When this was first observed, it appeared to threaten the survival of one of the most important conservation laws in physics!
To account for this energy release, Pauli proposed (in 1931) that there was emitted in the decay process another particle, later named by Fermi the neutrino. It was clear, this article must be highly penetrating and that the conservation of electric charge requires the neutrino to be electrically neutral. This would explain why it was so hard to detect this particle. The term neutrino comes from Italian, meaning “little neutral one”, and neutrinos are denoted by the Greek letter ν (nu). In the process of beta decay, the neutrino carries the missing energy, and also, in this process, the law of conservation of energy remains valid.