Article Summary & FAQs
What is the law of conservation of matter?
The law of conservation of matter is a general law in physics and chemistry applicable for any system closed to all transfers of matter and energy. According to this law, the mass of an object or collection of objects never changes over time, no matter how the constituent parts rearrange themselves. In other words, the mass can neither be created nor destroyed.
Key Facts
- The concept of mass conservation is widely used in many fields such as physics, chemistry, mechanics, and fluid dynamics.
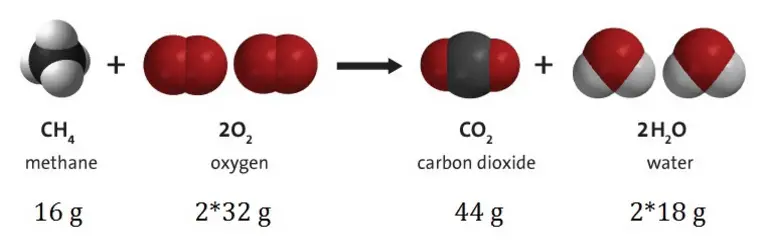
- In chemistry, the calculation of the amount of reactant and products in a chemical reaction, or stoichiometry, is founded on the principle of conservation of mass.
- In fluid dynamics, this law is represented by the continuity equation, which states that all mass flow rates into a control volume are equal to all mass flow rates out of the control volume plus the rate of change of mass within the control volume.
- In the special theory of relativity, certain types of matter may be created or destroyed. Still, the mass and energy associated with such matter remain unchanged in quantity in all of these processes.
The Law of Conservation of Matter – Conservation of Mass
The law of conservation of matter or principle of matter conservation states that the mass of an object or collection of objects never changes over time, no matter how the constituent parts rearrange themselves.
The mass can neither be created nor destroyed.
The law requires that during any nuclear reaction, radioactive decay or chemical reaction in an isolated system, the total mass of the reactants or starting materials must be equal to the mass of the products.
Historically, already the ancient Greeks proposed the idea that the total amount of matter in the universe is constant. Mikhail Lomonosov first outlined the principle of conservation of mass in 1748. However, the law of conservation of matter (or the principle of mass/matter conservation) as a fundamental principle of physics was discovered by Antoine Lavoisier in the late 18th century. It was of great importance in progressing from alchemy to modern chemistry. Before this discovery, there were questions like:
- Why a piece of wood weighs less after afterburning?
- Can a matter or some of its part disappear?
In the case of burned wood, the problem was the measurement of the weight of released gases. Measurements of the weight of released gases were complicated because of the buoyancy effect of the Earth’s atmosphere on the weight of gases. Once understood, the conservation of matter was crucial in progress from alchemy to the modern natural science of chemistry.
ṁin = ṁout
(ρAv)in = (ρAv)out
____________________________
Pressurized water reactors are cooled and moderated by high-pressure liquid water (e.g.,, 16MPa). At this pressure, water boils at approximately 350°C (662°F). The inlet temperature of the water is about 290°C (⍴ ~ 720 kg/m3). The water (coolant) is heated in the reactor core to approximately 325°C (⍴ ~ 654 kg/m3) as the water flows through the core.
The primary circuit of a typical PWR is divided into 4 independent loops (piping diameter ~ 700mm). Each loop comprises a steam generator and one main coolant pump. Inside the reactor pressure vessel (RPV), the coolant first flows down outside the reactor core (through the downcomer). The flow is reversed up through the core from the bottom of the pressure vessel, where the coolant temperature increases as it passes through the fuel rods and the assemblies formed by them.
Calculate:
- the primary piping volumetric flow rate (m3/s),
- the primary piping flow velocity (m/s),
- the core inlet flow velocity (m/s),
- the core outlet flow velocity (m/s)
when
- the mass flow rate in the hot leg of primary piping is equal to 4648 kg/s,
- Reactor core flow cross-section is equal to 5m2,
- Primary piping flow cross-section (single loop) is equal to 0.38 m2
Results:
Cold leg volumetric flow rate:
Qcold = ṁ / ⍴ = 4648 / 720 = 6.46 m3/s = 23240 m3/hod
Cold leg flow velocity:
A1 = π.d2 / 4
vcold = Qcold / A1 = 6.46 / (3.14 x 0.72 / 4) = 6.46 / 0.38 = 17 m/s
Hot leg volumetric flow rate:
Qhot = ṁ / ⍴ = 4648 / 654 = 7.11 m3/s = 25585 m3/hod
Hot leg flow velocity:
A = π.d2 / 4
vhot = Qhot / A1 = 7.11 / (3.14 x 0.72 / 4) = 7.11 / 0.38 = 18,7 m/s
or according to the continuity equation:
⍴1 . A1 . v1 = ⍴2 . A2 . v2
vhot = vcold . ⍴cold / ⍴hot = 17 x 720 / 654 = 18.7 m/s
Core inlet flow velocity:
Acore = 5m2
Apiping = 4 x A1 = 4 x 0.38 = 1.52 m2
⍴inlet = ⍴cold
according to the continuity equation:
⍴inlet . Acore . vinlet = ⍴cold . Apiping . vcold
vinlet = vcold . Apiping / Acore = 17 x 1.52 / 5 = 5.17 m/s
Core outlet flow velocity:
⍴inlet = ⍴cold
⍴outlet = ⍴hot
according to the continuity equation:
⍴outlet . Acore . voutlet = ⍴inlet . Acore . vinlet
voutlet = vinlet . ⍴inlet / ⍴outlet = 5.17 x 720 / 654 = 5.69 m/s
The Law of Conservation of Matter in Special Relativity Theory
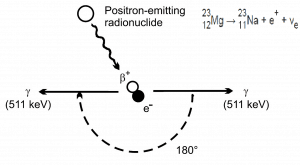
At the beginning of the 20th century, the notion of mass underwent a radical revision. The mass lost its absoluteness. One of the striking results of Einstein’s theory of relativity is that mass and energy are equivalent and convertible one into the other. Equivalence of the mass and energy is described by Einstein’s famous formula E = mc2. In other words, energy equals mass multiplied by the speed of light squared. Because the speed of light is very large, the formula implies that any small amount of matter contains a very large amount of energy. The mass of an object was seen as equivalent to energy, interconvertible with energy, and increasing significantly at exceedingly high speeds near that of light. The total energy was understood to comprise its rest mass and its increase of mass caused by increased kinetic energy.
In the special theory of relativity, certain types of matter may be created or destroyed. Still, the mass and energy associated with such matter remain unchanged in quantity in all of these processes. It was found the rest mass of an atomic nucleus is measurably smaller than the sum of the rest masses of its constituent protons, neutrons, and electrons. Mass was no longer considered unchangeable in the closed system. The difference is a measure of the nuclear binding energy which holds the nucleus together. According to the Einstein relationship (E = mc2), this binding energy is proportional to this mass difference, known as the mass defect.
Source: hyperphysics.phy-astr.gsu.edu
During the nuclear splitting or nuclear fusion, some of the mass of the nucleus gets converted into huge amounts of energy. Thus this mass is removed from the total mass of the original particles, and the mass is missing in the resulting nucleus. The nuclear binding energies are enormous, and they are a million times greater than the electron binding energies of atoms.
Generally, in both chemical and nuclear reactions, some conversion between rest mass and energy occurs so that the products generally have smaller or greater mass than the reactants. Therefore the new conservation principle is the conservation of mass energy.
See also: Energy Release from Fission.
Matter – Antimatter creation occurs naturally in high-energy processes involving cosmic rays and also in high-energy experiments in accelerators on Earth. High-energy cosmic rays impacting Earth’s atmosphere (or any other matter in the Solar System) produce minute quantities of antiparticles in the resulting particle jets, which are immediately annihilated by contact with nearby matter. The presence of the resulting antimatter is detectable by the two gamma rays (with 511 keV) produced every time positrons annihilate with nearby matter.
Antimatter creation is also very common in the nuclear decay of many isotopes. Let assume a decay of potassium-40. Naturally occurring potassium is composed of three isotopes, of which 40K is radioactive. Traces of 40K are found in all potassium, and it is the most common radioisotope in the human body. 40K is a radioactive isotope of potassium that has a very long half-life of 1.251×109 years and undergoes both types of beta decay.
- About 89.28% of the time (10.72% is by electron capture), it decays to calcium-40 (40Ca) with emission of a beta particle (β−, an electron) with a maximum energy of 1.33 MeV and an antineutrino, which is an antiparticle to the neutrino.
- Very rarely (0.001% of the time), it will decay to 40Ar by emitting a positron (β+) and a neutrino.
Another very interesting source of antimatter is, in fact, a nuclear reactor. Nuclear reactors are the major source of human-generated antineutrinos. This is because antineutrinos are produced in negative beta decay. A nuclear reactor occurs especially the β−decay because the common feature of the fission fragments is an excess of neutrons. Please note that billions of solar neutrinos per second pass (mostly without any interaction) through every square centimeter (~6×1010) on the Earth’s surface, and antineutrino radiation is by no means dangerous.
Finally, the fact is that antimatter is much more common than it may seem.
In January 2011, research by the American Astronomical Society discovered antimatter (positrons) originating above thunderstorm clouds. It is suggested that these positrons are formed in terrestrial gamma-ray flashes (TGF). These positions are produced in gamma-ray flashes created by electrons accelerated by strong electric fields in the clouds. TGFs are brief bursts occurring inside thunderstorms and associated with lightning. The streams of positrons and electrons collide higher in the atmosphere to generate more gamma rays. About 500 TGFs may occur every day worldwide but mostly go undetected.
See also: Electron-Positron Pair Production.
See also: Reactor as the Source of Antineutrinos
As was written, a particle and its antiparticle have the same mass but opposite electric charge and other differences in quantum numbers. That means a proton has a positive charge while an antiproton has a negative charge, and therefore they attract each other. A collision between any particle and its antiparticle partner is known to lead to their mutual annihilation. Since matter and antimatter carry an immense amount of energy (due to E = mc2), their mutual annihilation is associated with producing intense photons (gamma rays), neutrinos, and sometimes less-massive particle-antiparticle pairs.
One of the best-known processes is electron-positron annihilation. Electron–positron annihilation occurs when a negatively charged electron and a positively charged positron collide. When a low-energy electron annihilates a low-energy positron (the electron’s antiparticle), it can only produce two or more photons (gamma rays). The production of only one photon is forbidden because of the conservation of linear momentum and total energy. The production of another particle is also forbidden because both particles (electron-positron) together do not carry enough mass energy to produce heavier particles. When an electron and a positron collide, they annihilate, resulting in the complete conversion of their rest mass to pure energy (according to the E=mc2 formula) in the form of two oppositely directed 0.511 MeV gamma rays (photons).
e− + e+ → γ + γ (2x 0.511 MeV)
This process must satisfy many conservation laws, including:
- Conservation of electric charge. The net charge before and after is zero.
- Conservation of linear momentum and total energy. T
- Conservation of angular momentum.
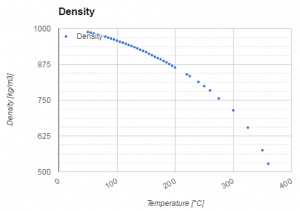
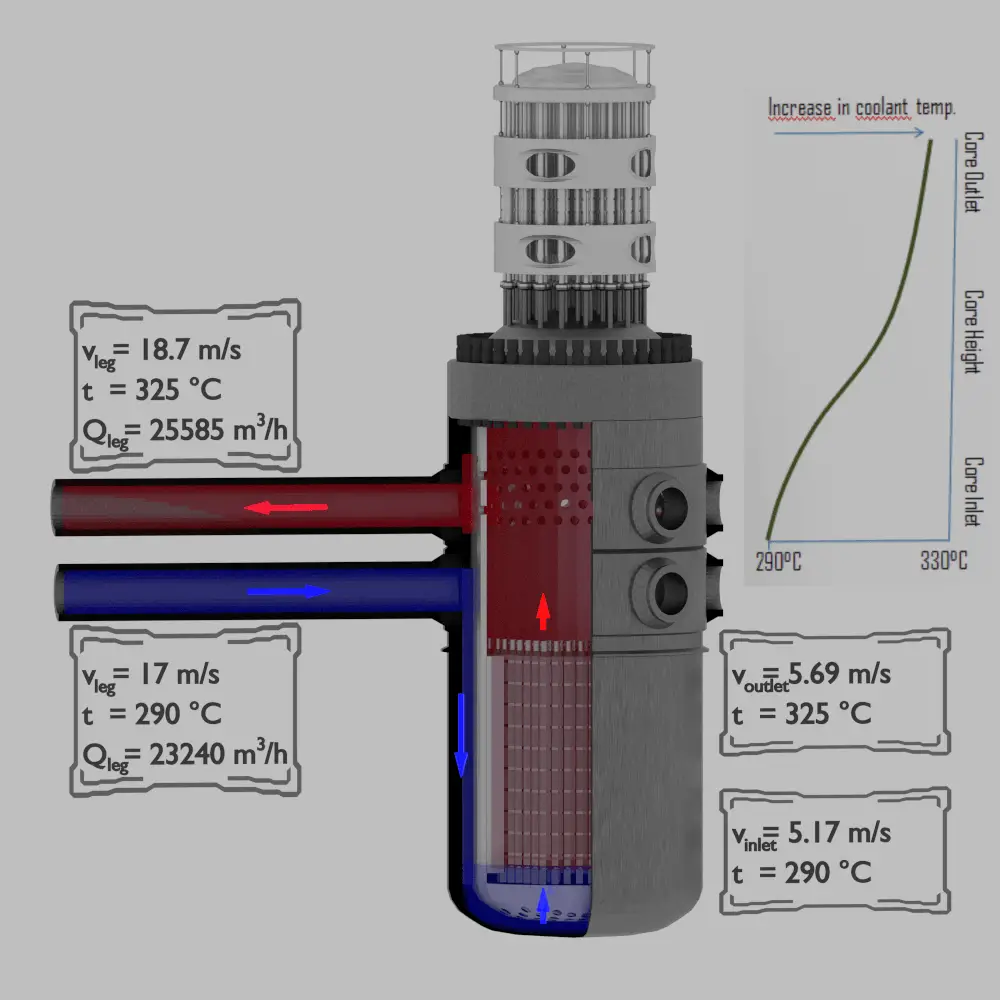
The Law of Conservation of Matter in Fluid Dynamics
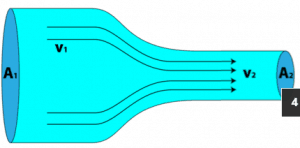
This principle is generally known as the conservation of matter principle. It states that the mass of an object or collection of objects never changes over time, no matter how the constituent parts rearrange themselves. This principle can be used in the analysis of flowing fluids. Conservation of mass in fluid dynamics states that all mass flow rates into a control volume are equal to all mass flow rates out of the control volume plus the rate of mass change within the control volume. This principle is expressed mathematically by the following equation:
ṁin = ṁout +∆m⁄∆t
Mass entering per unit time = mass leaving per unit time + Increase of mass in the control volume per unit time
This equation describes nonsteady-state flow. Nonsteady-state flow refers to the condition where the fluid properties at any single point in the system may change over time. Steady-state flow refers to the condition where the fluid properties (temperature, pressure, and velocity) at any single point in the system do not change over time. But one of the most significant constant properties in a steady-state flow system is the system mass flow rate. This means that there is no accumulation of mass within any component in the system.
See also: Continuity Equation
Continuity Equation
The continuity equation is simply a mathematical expression of the principle of conservation of mass. For a control volume with a single inlet and a single outlet, the principle of conservation of mass states that, for steady-state flow, the mass flow rate into the volume must equal the mass flow rate out.
ṁin = ṁout
Mass entering per unit time = mass leaving per unit time
This equation is called the continuity equation for steady one-dimensional flow. The net mass flow must be zero for a steady flow through a control volume with many inlets and outlets, where negative inflows and outflows are positive.
This principle can be applied to a stream tube such as that shown above. No fluid flows across the boundary made by the streamlines, so mass only enters and leaves through the two ends of this stream tube section.
When a fluid is in motion, it must move in such a way that mass is conserved. To see how mass conservation places restrictions on the velocity field, consider the steady flow of fluid through a duct (that is, the inlet and outlet flows do not vary with time).
Differential Form of Continuity Equation
A general continuity equation can also be written in a differential form:
∂⍴⁄∂t + ∇ . (⍴ ͞v) = σ
where
- ∇ . is divergence,
- ρ is the density of quantity q,
- ⍴ ͞v is the flux of quantity q,
- σ is the generation of q per unit volume per unit time. Terms that generate (σ > 0) or remove (σ < 0) q are referred to as a “sources” and “sinks” respectively. If q is a conserved quantity (such as energy), σ is equal to 0.