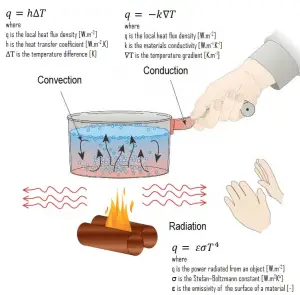
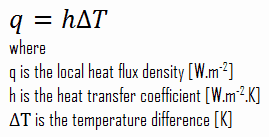In thermal conduction, energy is transferred as heat either due to the migration of free electrons or lattice vibrational waves (phonons). There is no mass movement in the direction of energy flow, and heat transfer by conduction depends on the driving “force” of temperature difference.
In general, convection is either the mass transfer or the heat transfer due to the bulk movement of molecules within fluids such as gases and liquids.
Conduction and convection are similar in that both mechanisms require the presence of a material medium (in comparison to thermal radiation). On the other hand, they are different in that convection requires the presence of fluid motion.
At the surface, it must be emphasized that energy flow occurs purely by conduction, even in conduction. It is because there is always a thin stagnant fluid film layer on the heat transfer surface. But in the next layers, both conduction and diffusion-mass movement occur at the molecular or macroscopic levels. Due to the mass movement, the rate of energy transfer is higher. The higher the mass movement rate, the thinner the stagnant fluid film layer will be, and the higher the heat flow rate.
It must be noted nucleate boiling at the surface effectively disrupts this stagnant layer. Therefore, nucleate boiling significantly increases the ability of a surface to transfer thermal energy to the bulk fluid.
As was written, heat transfer through a fluid is by convection in the presence of mass movement and conduction in its absence. Therefore, thermal conduction in a fluid can be viewed as the limiting case of convection, corresponding to the case of quiescent fluid.
Convection as a Conduction with Fluid Motion
Some experts do not consider convection to be a fundamental mechanism of heat transfer since it is essentially heat conduction in the presence of fluid motion. They consider it a special case of thermal conduction, known as “conduction with fluid motion”. On the other hand, it is practical to recognize convection as a separate heat transfer mechanism despite the valid arguments to the contrary.
What is Conduction
Thermal conduction, also called heat conduction, occurs within a body or between two bodies in contact without the involvement of mass flow and mixing. It is the direct microscopic exchange of kinetic energy of particles through the boundary between two systems. Heat transfer by conduction depends on the driving “force” of the temperature difference and the thermal conductivity (or the resistance to heat transfer). The thermal conductivity depends on the nature and dimensions of the heat transfer medium. All heat transfer problems involve the temperature difference, geometry, and the physical properties of the object being studied. In conduction heat transfer problems, the studied object is usually solid.
Microscopically this mode of energy transfer is attributed to free-electron flow from higher to lower energy levels, lattice vibration, and molecular collision. Consider a block of stone at high temperatures that consist of atoms oscillating intensely around their average positions. At low temperatures, the atoms continue to oscillate but with less intensity. If a hotter block of stone is put in contact with a cooler block, the intensely oscillating atoms at the edge of the hotter block give off their kinetic energy to the less oscillating atoms at the edge of the cool block. In this case, there is energy transfer between these two blocks, and heat flows from the hotter to the cooler block by these random vibrations. The modern view is to ascribe the energy transfer to lattice waves induced by atomic motion. In electrical insulators, the energy transfer is exclusive via these lattice waves. In a conductor, it is also due to the translational motion of the free electrons.
Fourier’s law of Thermal Conduction
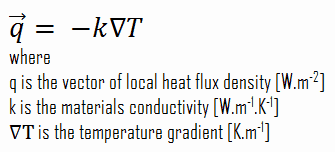
Heat transfer processes can be quantified in terms of appropriate rate equations. The rate equation in this heat transfer mode is based on Fourier’s law of thermal conduction. This law states that the time rate of heat transfer through a material is proportional to the negative gradient in the temperature and the area, at right angles to that gradient, through which the heat flows. Its differential form is:
What is Convection
In general, convection is either the mass transfer or the heat transfer due to the bulk movement of molecules within fluids such as gases and liquids. Although liquids and gases are generally not very good conductors of heat, they can transfer heat quite rapidly by convection.
Convection takes place through advection, diffusion, or both. Convection cannot occur in most solids because neither significant diffusion of matter nor bulk current flows can occur. Diffusion of heat occurs in rigid solids, but that is called thermal conduction.
The process of heat transfer between a surface and a fluid flowing in contact with it is called convective heat transfer. In engineering, convective heat transfer is one of the major mechanisms of heat transfer. When heat is transferred from one fluid to another through a barrier, convection is involved on both sides of the barrier. In most cases, the main resistance to heat flow is by convection. Convective heat transfer occurs both by thermal diffusion (the random motion of fluid molecules) and by advection, in which matter or heat is transported by the larger-scale motion of currents in the fluid.
Heat transfer by convection is more difficult to analyze than heat transfer by conduction because no single property of the heat transfer medium, such as thermal conductivity, can be defined to describe the mechanism. Convective heat transfer is complicated by the fact that it involves fluid motion as well as heat conduction. Heat transfer by convection varies from situation to situation (upon the fluid flow conditions), and it is frequently coupled with the mode of fluid flow. In forced convection, the rate of heat transfer through a fluid is much higher by convection than by conduction.
Newton’s Law of Cooling
Despite the complexity of convection, the rate of convection heat transfer is observed to be proportional to the temperature difference. It is conveniently expressed by Newton’s law of cooling, which states that:
The rate of heat loss of a body is directly proportional to the difference in the temperatures between the body and its surroundings provided the temperature difference is small and the nature of radiating surface remains the same.
Note that, ΔT is given by the surface or wall temperature, Twall and the bulk temperature, T∞, which is the temperature of the fluid sufficiently far from the surface.