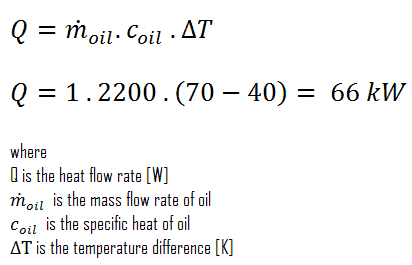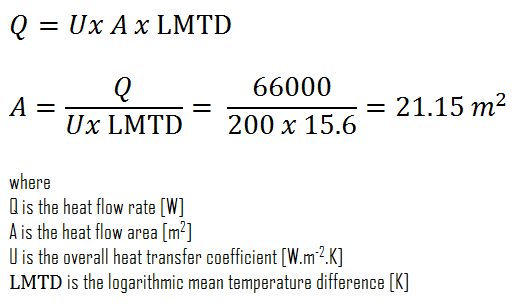In the analysis of heat exchangers, it is often convenient to work with an
overall heat transfer coefficient, known as a
U-factor. The U-factor is defined by an expression analogous to
Newton’s law of cooling. Moreover, engineers also use the logarithmic mean temperature difference (
LMTD) to determine the temperature driving force for heat transfer in heat exchangers.
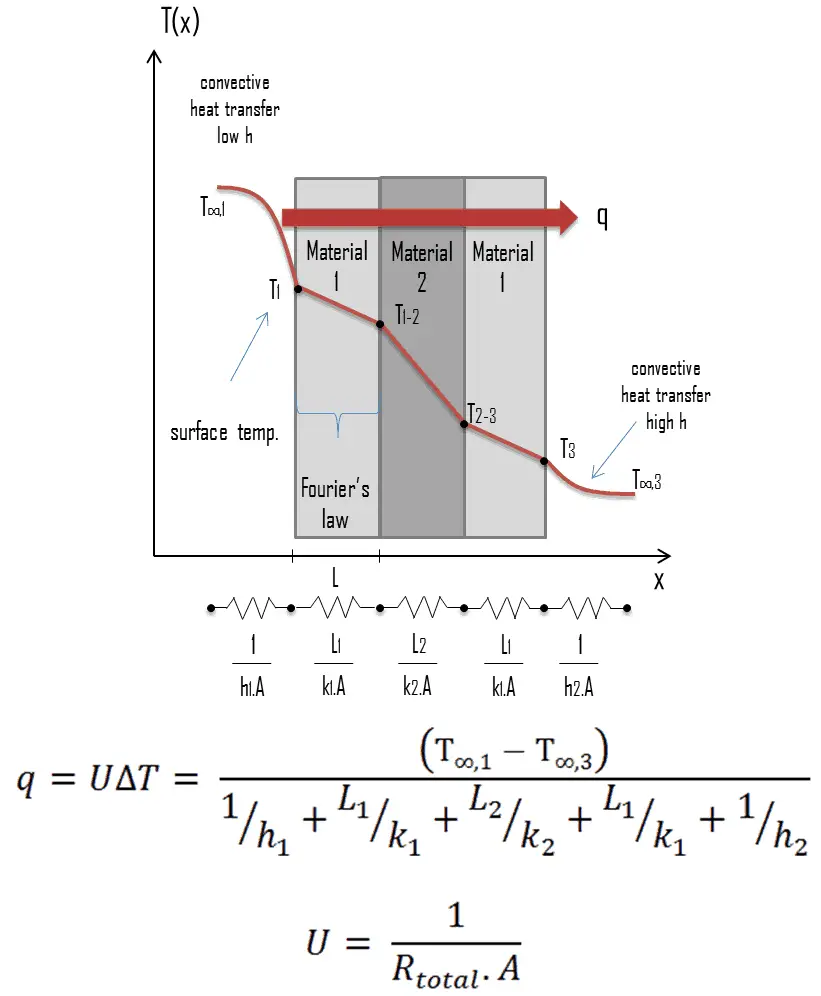
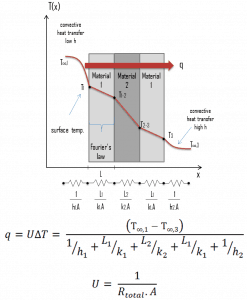 A heat exchanger typically involves two flowing fluids separated by a solid wall. Many of the heat transfer processes encountered in the industry involve composite systems and even involve a combination of both conduction and convection. Heat is first transferred from the hot fluid to the wall by convection, through the wall by conduction, and then to the cold fluid again by convection.
A heat exchanger typically involves two flowing fluids separated by a solid wall. Many of the heat transfer processes encountered in the industry involve composite systems and even involve a combination of both conduction and convection. Heat is first transferred from the hot fluid to the wall by convection, through the wall by conduction, and then to the cold fluid again by convection.
It is often convenient to work with an overall heat transfer coefficient, known as a U-factor with these composite systems. The U-factor is defined by an expression analogous to Newton’s law of cooling:
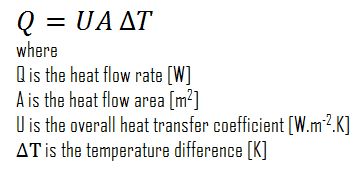
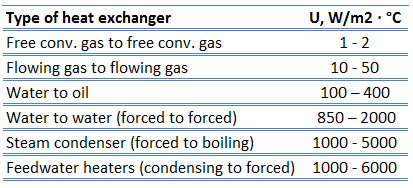 The overall heat transfer coefficient, U, is related to the total thermal resistance and depends on the geometry of the problem. For example, heat transfer in a steam generator involves convection from the bulk of the reactor coolant to the steam generator inner tube surface, conduction through the tube wall, and convection (boiling) from the outer tube surface to the secondary side fluid.
The overall heat transfer coefficient, U, is related to the total thermal resistance and depends on the geometry of the problem. For example, heat transfer in a steam generator involves convection from the bulk of the reactor coolant to the steam generator inner tube surface, conduction through the tube wall, and convection (boiling) from the outer tube surface to the secondary side fluid.
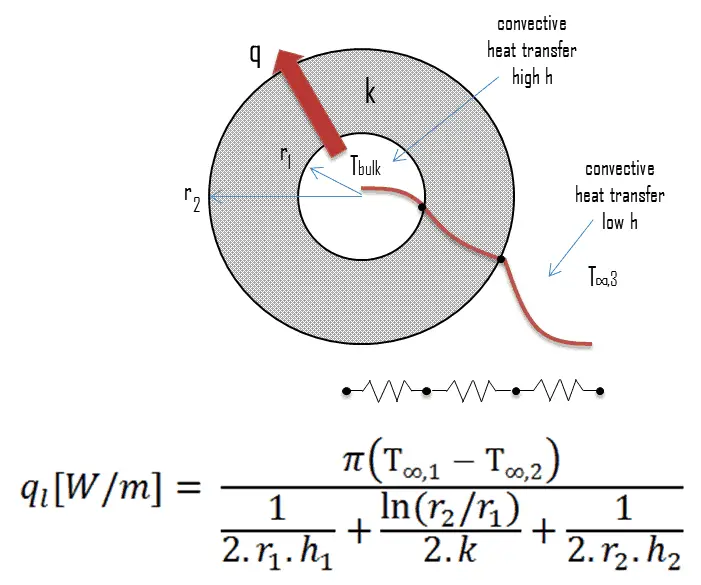
In cases of combined heat transfer for a heat exchanger, there are two values for h. The convective heat transfer coefficient (h) for the fluid film inside the tubes and a convective heat transfer coefficient for the fluid film outside the tubes. The tube wall’s thermal conductivity (k) and thickness (Δx) must also be accounted for.
Overall Heat Transfer Coefficient – Plane Wall
Overall Heat Transfer Coefficient – Cylindrical Tubes
Steady heat transfer through multilayered cylindrical or spherical shells can be handled just like multilayered plane walls.
Online monitoring of commercial heat exchangers is done by tracking the overall heat transfer coefficient because the overall heat transfer coefficient tends to decline over time due to fouling. By periodically calculating the overall heat transfer coefficient from exchanger flow rates and temperatures, the operator of the heat exchanger can estimate the lifetime of heat exchangers.
Fouling - Fouling Factor
Online monitoring of commercial heat exchangers is done by tracking the overall heat transfer coefficient because the overall heat transfer coefficient tends to decline over time due to fouling. Fouling is the accumulation of unwanted material on solid surfaces to the detriment of function. The fouling materials can consist of either living organisms or non-living substances (minerals or organic compounds). The layer of deposits represents additional resistance to heat transfer and causes the rate of heat transfer in a heat exchanger to decrease. As a result, fouling in heat exchangers reduces thermal efficiency, decreases heat flux, increases the temperature on the hot side, decreases temperature on the cold side, induces under-deposit corrosion, increases the use of cooling water. The net effect of these accumulations on heat transfer is represented by a fouling factor, Rf, which measures the overall thermal resistance introduced by fouling.
Example: Calculation of Heat Exchanger
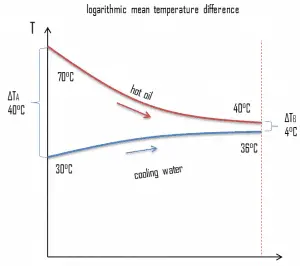 Consider a parallel-flow heat exchanger used to cool oil from 70°C to 40°C using water available at 30°C. The outlet temperature of the water is 36°C, and the rate of flow of oil is 1 kg/s. The specific heat of the oil is 2.2 kJ/kg K. The overall heat transfer coefficient U = 200 W/m2 K.
Consider a parallel-flow heat exchanger used to cool oil from 70°C to 40°C using water available at 30°C. The outlet temperature of the water is 36°C, and the rate of flow of oil is 1 kg/s. The specific heat of the oil is 2.2 kJ/kg K. The overall heat transfer coefficient U = 200 W/m2 K.
Calculate the logarithmic mean temperature difference. Determine the area of this heat exchanger required for this performance.
- LMTD
The logarithmic mean temperature difference can be calculated simply using its definition:

- Area of Heat Exchanger
To calculate the area of this heat exchanger, we have to calculate the heat flow rate using the mass flow rate of oil and LMTD.
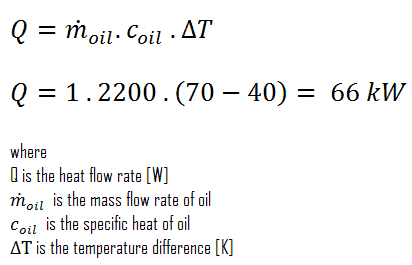
The required area of this heat exchanger can be then directly calculated using the general heat transfer equation:
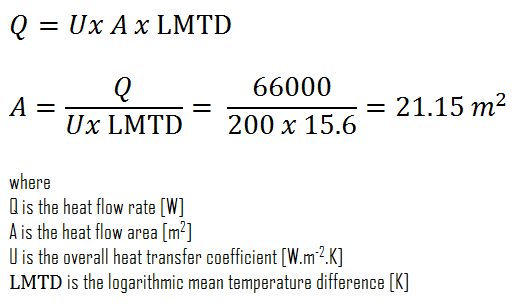
References:
Heat Transfer:
- Fundamentals of Heat and Mass Transfer, 7th Edition. Theodore L. Bergman, Adrienne S. Lavine, Frank P. Incropera. John Wiley & Sons, Incorporated, 2011. ISBN: 9781118137253.
- Heat and Mass Transfer. Yunus A. Cengel. McGraw-Hill Education, 2011. ISBN: 9780071077866.
- U.S. Department of Energy, Thermodynamics, Heat Transfer and Fluid Flow. DOE Fundamentals Handbook, Volume 2 of 3. May 2016.
Nuclear and Reactor Physics:
- J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
- J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
- W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
- Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
- W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
- G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
- Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
- U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.
- Paul Reuss, Neutron Physics. EDP Sciences, 2008. ISBN: 978-2759800414.
Advanced Reactor Physics:
- K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
- K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
- D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
- E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.
See above:
Heat Exchangers
 A heat exchanger typically involves two flowing fluids separated by a solid wall. Many of the heat transfer processes encountered in the industry involve composite systems and even involve a combination of both conduction and convection. Heat is first transferred from the hot fluid to the wall by convection, through the wall by conduction, and then to the cold fluid again by convection.
A heat exchanger typically involves two flowing fluids separated by a solid wall. Many of the heat transfer processes encountered in the industry involve composite systems and even involve a combination of both conduction and convection. Heat is first transferred from the hot fluid to the wall by convection, through the wall by conduction, and then to the cold fluid again by convection. The overall heat transfer coefficient, U, is related to the total thermal resistance and depends on the geometry of the problem. For example, heat transfer in a steam generator involves convection from the bulk of the reactor coolant to the steam generator inner tube surface, conduction through the tube wall, and convection (boiling) from the outer tube surface to the secondary side fluid.
The overall heat transfer coefficient, U, is related to the total thermal resistance and depends on the geometry of the problem. For example, heat transfer in a steam generator involves convection from the bulk of the reactor coolant to the steam generator inner tube surface, conduction through the tube wall, and convection (boiling) from the outer tube surface to the secondary side fluid. Consider a parallel-flow heat exchanger used to cool oil from 70°C to 40°C using water available at 30°C. The outlet temperature of the water is 36°C, and the rate of flow of oil is 1 kg/s. The specific heat of the oil is 2.2 kJ/kg K. The overall heat transfer coefficient U = 200 W/m2 K.
Consider a parallel-flow heat exchanger used to cool oil from 70°C to 40°C using water available at 30°C. The outlet temperature of the water is 36°C, and the rate of flow of oil is 1 kg/s. The specific heat of the oil is 2.2 kJ/kg K. The overall heat transfer coefficient U = 200 W/m2 K.