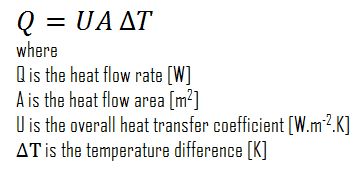
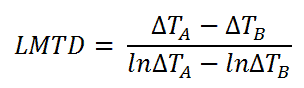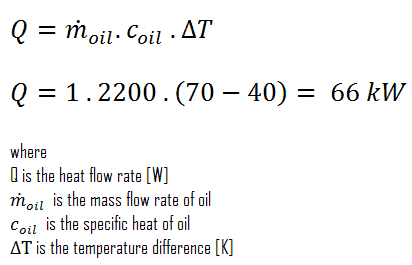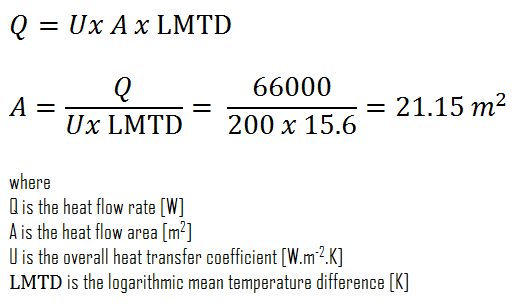Facebook Instagram Youtube Twitter

Logarithmic Mean Temperature Difference – LMTD

To solve certain heat exchanger problems, engineers often use a logarithmic mean temperature difference (LMTD), which is used to determine the temperature driving force for heat transfer in heat exchangers. LMTD is introduced because the temperature change that takes place across the heat exchanger from the entrance to the exit is not linear.

The heat transfer through the wall of a heat exchanger at a given location is given by the following equation:

overall heat transfer coefficient - equation

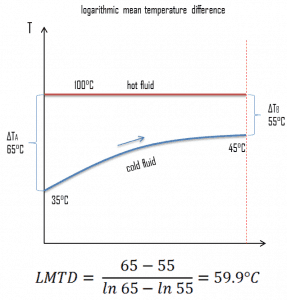logarithmic mean temperature difference - exampleHere, the overall heat transfer coefficient value can be assumed as a constant. On the other hand, the temperature difference continuously varies with location (especially in counter-flow arrangement). To determine the total heat flow, either the heat flow should be summed up using elemental areas and the temperature difference at the location. More conveniently, engineers can average the value of temperature difference. The heat exchanger equation can be solved much easier by defining a “Mean Temperature Difference” (MTD). It can be seen from the figure that the temperature difference varies along with the flow, and the arithmetic average may not be the real average; therefore, engineers use the logarithmic mean temperature difference. The “Logarithmic Mean Temperature Difference“ (LMTD) is a logarithmic average of the temperature difference between the hot and cold feeds at each end of the heat exchanger. The larger the LMTD, the more heat is transferred. It can be seen from the figure that the temperature difference varies along with the flow, and the arithmetic average may not be the real average.

For a heat exchanger that has two ends (which we call “A” and “B”) at which the hot and cold streams enter or exit on either side, the LMTD is defined as:

logarithmic mean temperature difference - definition

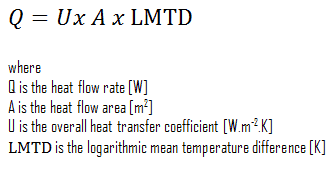
The heat transfer is then given by:

LMTD - heat transfer equation

This holds both for parallel-flow arrangement, where the streams enter from the same end, and for counter-flow arrangement, they enter from different ends.

In a cross-flow, where one system, usually the heat sink, has the same nominal temperature at all points on the heat transfer surface, a similar relation between exchanged heat and LMTD holds, but with a correction factor. A correction factor is also required for other more complex geometries, such as a shell and tube exchanger with baffles.

LMTD – Condensers and Boilers

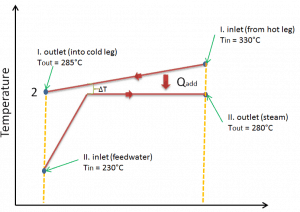
Steam generator - counterflow heat exchanger
Temperature gradients in a typical PWR steam generator.

Steam generators and condensers are also examples of components found in nuclear facilities where the concept of LMTD is needed to address certain problems. When the subcooled water enters the steam generator, it must be heated up to its boiling point, and then it must be evaporated. Because evaporation occurs at a constant temperature, it cannot be used as a single LMTD. In this case, the heat exchanger must be treated as a combination of two or three (when superheat occurs) heat exchangers.

Example: Calculation of Heat Exchanger

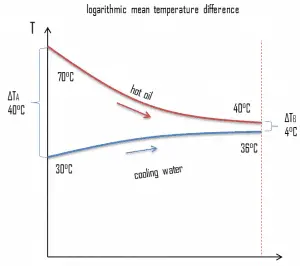example - heat exchanger calculation - LMTDConsider a parallel-flow heat exchanger used to cool oil from 70°C to 40°C using water available at 30°C. The outlet temperature of the water is 36°C, and the rate of flow of oil is 1 kg/s. The specific heat of the oil is 2.2 kJ/kg K. The overall heat transfer coefficient U = 200 W/m2 K.

Calculate the logarithmic mean temperature difference. Determine the area of this heat exchanger required for this performance.

  1. LMTD

The logarithmic mean temperature difference can be calculated simply using its definition:

LMTD - example

  1. Area of Heat Exchanger

To calculate the area of this heat exchanger, we have to calculate the heat flow rate using the mass flow rate of oil and LMTD.

Energy Balance - Example

The required area of this heat exchanger can be then directly calculated using the general heat transfer equation:

heat exchanger - calculation

 
References:
Heat Transfer:
  1. Fundamentals of Heat and Mass Transfer, 7th Edition. Theodore L. Bergman, Adrienne S. Lavine, Frank P. Incropera. John Wiley & Sons, Incorporated, 2011. ISBN: 9781118137253.
  2. Heat and Mass Transfer. Yunus A. Cengel. McGraw-Hill Education, 2011. ISBN: 9780071077866.
  3. U.S. Department of Energy, Thermodynamics, Heat Transfer and Fluid Flow. DOE Fundamentals Handbook, Volume 2 of 3. May 2016.

Nuclear and Reactor Physics:

  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  7. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  8. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.
  9. Paul Reuss, Neutron Physics. EDP Sciences, 2008. ISBN: 978-2759800414.

Advanced Reactor Physics:

  1. K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
  2. K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
  3. D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
  4. E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.

See above:

Heat Exchangers