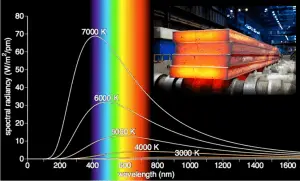
Wien’s displacement law, and the fact that the frequency is inversely proportional to the wavelength, also indicate that the peak frequency fmax (object’s color) is proportional to the absolute temperature T of the blackbody.
According to Wien’s displacement law, the spectral radiance of black body radiation per unit wavelength peaks at the wavelength λmax given by:
where T is the absolute temperature in Kelvins, b is a constant of proportionality, known as Wien’s displacement constant, equal to 2.8978 × 10−3 K.m.
As can be seen from the figure, the blackbody radiation curve for different temperatures peaks at a wavelength inversely proportional to the temperature. Wien’s law (named after a German physicist) describes the shift of that peak in terms of temperature. Wien’s displacement law, and the fact that the frequency is inversely proportional to the wavelength, also indicate that the peak frequency fmax (object’s color) is proportional to the absolute temperature T of the blackbody. Therefore, as temperature increases, the glow color changes from red to yellow to white to blue.
According to Wien’s displacement law, the spectral radiance of black body radiation per unit wavelength peaks at the wavelength λmax given by:
 where T is the absolute temperature in Kelvins, b is a constant of proportionality, known as Wien’s displacement constant, equal to 2.8978 × 10−3 K.m. It must be noted that even at a white-hot temperature of 2000 K, about 99% of the radiant energy is still radiated away in the infrared (invisible) spectrum.
where T is the absolute temperature in Kelvins, b is a constant of proportionality, known as Wien’s displacement constant, equal to 2.8978 × 10−3 K.m. It must be noted that even at a white-hot temperature of 2000 K, about 99% of the radiant energy is still radiated away in the infrared (invisible) spectrum.
Although the shift of that peak is a direct consequence of Planck’s law, it had been discovered by Wilhelm Wien several years before Max Planck developed that more general equation.
Blackbody Radiation
It is known that the amount of radiation energy emitted from a surface at a given wavelength depends on the material of the body and the condition of its surface, as well as the surface temperature. Therefore, various materials emit different amounts of radiant energy even when they are at the same temperature. A body that emits the maximum amount of heat for its absolute temperature is called a blackbody.
A blackbody is an idealized physical body that has specific properties. By definition, a black body in thermal equilibrium emissivity of ε = 1.0. Real objects do not radiate as much heat as a perfect black body, and they radiate less heat than a black body and therefore are called gray bodies.
The surface of a blackbody emits thermal radiation at the rate of approximately 448 watts per square meter at room temperature (25 °C, 298.15 K). Real objects with emissivities less than 1.0 (e.g., copper wire) emit radiation at correspondingly lower rates (e.g., 448 x 0.03 = 13.4 W/m2). Emissivity plays an important role in heat transfer problems. For example, solar heat collectors incorporate selective surfaces with very low emissivities. These collectors waste very little solar energy through the emission of thermal radiation.
Since the absorptivity and the emissivity are interconnected by Kirchhoff’s Law of thermal radiation, a blackbody is also a perfect absorber of electromagnetic radiation.
Kirchhoff’s Law of thermal radiation:
For an arbitrary body emitting and absorbing thermal radiation in thermodynamic equilibrium, the emissivity is equal to the absorptivity.
emissivity ε = absorptivity α
A blackbody absorbs all incident electromagnetic radiation, regardless of frequency or angle of incidence. Therefore, its absorptivity is equal to unity, which is also the highest possible value. A blackbody is a perfect absorber (and a perfect emitter).
Note that visible radiation occupies a very narrow spectrum band from 400 to 760 nm. We cannot make any judgments about the blackness of a surface based on visual observations. For example, consider a white paper that reflects visible light and thus appears white. On the other hand, it is essentially black for infrared radiation (absorptivity α = 0.94) since they strongly absorb long-wavelength radiation.