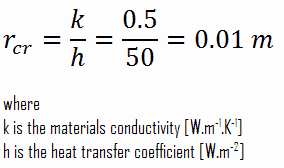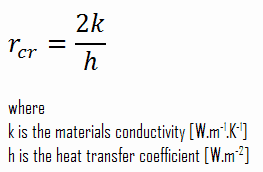In a plane wall, the area perpendicular to the direction of heat flow adding more insulation to a wall always decreases heat transfer. The thicker the insulation, the lower the heat transfer rate, and this is because the outer surface always has the same area.
But in cylindrical and spherical coordinates, the addition of insulation also increases the outer surface, which decreases the convection resistance at the outer surface. Moreover, in some cases, a decrease in the convection resistance due to the increase in surface area can be more significant than an increase in conduction resistance due to thicker insulation. As a result, the total resistance may actually decrease, resulting in increased heat flow.
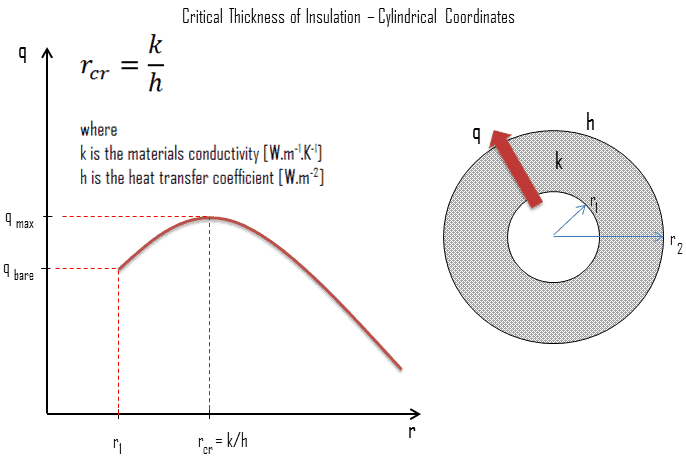
The thickness up to which heat flow increases and after which heat flow decreases is termed critical thickness. In the case of cylinders and spheres, it is called the critical radius. The critical radius of insulation can be derived depending on the thermal conductivity of the insulation k and the external convection heat transfer coefficient h.
As can be seen, if r1 < rcr, as it is in this case, the total resistance decreases, and the heat rate, therefore, increases with the addition of insulation. This trend continues until the outer radius of the insulation corresponds to the critical radius, where the heat rate reaches its maximum. This trend is desirable for the cooling of an electrical wire since the addition of electrical insulation would aid in transferring heat dissipated in the wire to the surroundings. On the other hand, any further addition of material (beyond rcr) would increase the total resistance and decrease heat loss. This behavior would be desirable for the insulation of pipes, where insulation is added to reduce heat loss to the surroundings.
Example – Critical Thickness of Insulation
Assume a steel pipe of r1 = 10 mm, exposed to natural convection at h = 50 W/m2.K. This pipe is insulated by the material of thermal conductivity k = 0.5 W/m.K. Determine the critical thickness of this combination:
Hence rcr > r1 and heat transfer will increase with the addition of insulation up to a thickness of rcr – r1 = (0.010 – 0.005)m = 0.005 m
Critical Thickness of Insulation – Spherical Coordinates
It can be shown similarly that the critical radius of insulation for a spherical shell is: