 The strength of materials considers the relationship between the external loads applied to a material and the resulting deformation or change in material dimensions. In designing structures and machines, it is important to consider these factors so that the material selected will have adequate strength to resist applied loads or forces and retain its original shape. The strength of a material is its ability to withstand this applied load without failure or plastic deformation.
The strength of materials considers the relationship between the external loads applied to a material and the resulting deformation or change in material dimensions. In designing structures and machines, it is important to consider these factors so that the material selected will have adequate strength to resist applied loads or forces and retain its original shape. The strength of a material is its ability to withstand this applied load without failure or plastic deformation.
However, we must note that the load which will deform a small component will be less than the load to deform a larger component of the same material. Therefore, the load (force) is not a suitable term for strength. Instead, we can use the force (load) per unit of area (σ = F/A), called stress, which is constant (until deformation occurs) for a given material regardless of the size of the component part. In this concept, strain is also a very important variable since it defines the deformation of an object. In summary, the mechanical behavior of solids is usually defined by constitutive stress-strain relations. A deformation is called elastic deformation if the stress is a linear function of strain. In other words, stress and strain follow Hooke’s law. Beyond the linear region, stress and strain show nonlinear behavior. This inelastic behavior is called plastic deformation.
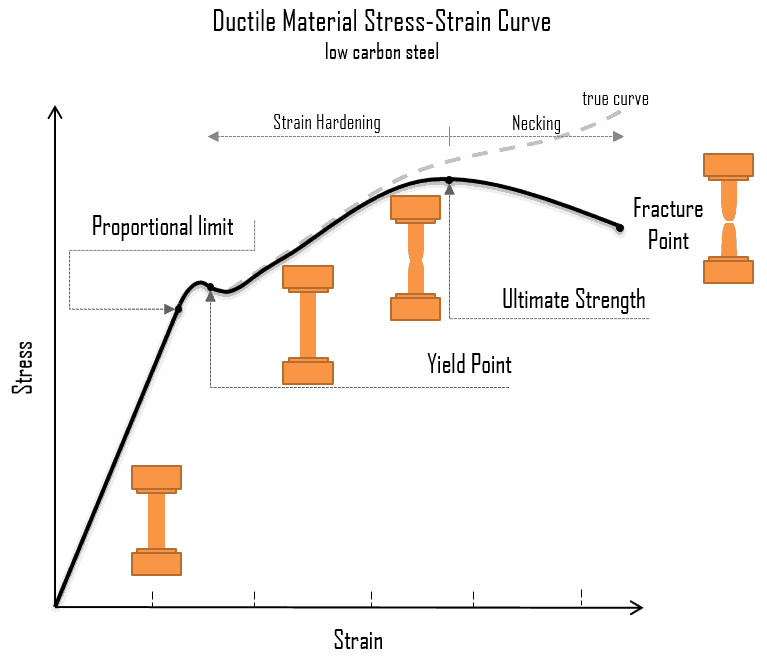
A schematic diagram for the stress-strain curve of low carbon steel at room temperature is shown in the figure. Several stages show different behaviors, which suggests different mechanical properties. Materials can miss one or more stages shown in the figure or have totally different stages to clarify. In this case, we have to distinguish between stress-strain characteristics of ductile and brittle materials. The following points describe the different regions of the stress-strain curve and the importance of several specific locations.
- Proportional limit. The proportional limit corresponds to the location of stress at the end of the linear region, so the stress-strain graph is a straight line, and the gradient will be equal to the elastic modulus of the material. For tensile and compressive stress, the slope of the portion of the curve where stress is proportional to strain is referred to as Young’s modulus, and Hooke’s Law applies. Between the proportional limit and the yield point, Hooke’s Law becomes questionable, and strain increases more rapidly.
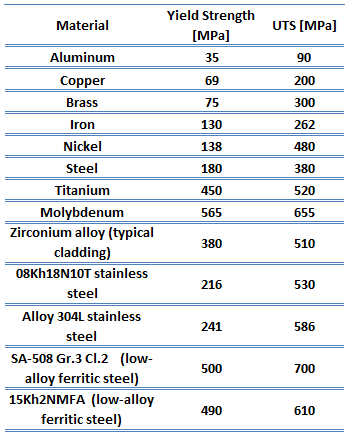 Yield point. The yield point is the point on a stress-strain curve that indicates the limit of elastic behavior and the beginning plastic behavior. Yield strength or yield stress is the material property defined as the stress at which a material begins to deform plastically. In contrast, the yield point is where nonlinear (elastic + plastic) deformation begins. Before the yield point, the material will deform elastically and return to its original shape when the applied stress is removed. Once the yield point is passed, some fraction of the deformation will be permanent and non-reversible. Some steels and other materials exhibit a behavior termed a yield point phenomenon. Yield strengths vary from 35 MPa for low-strength aluminum to greater than 1400 MPa for high-strength steel.
Yield point. The yield point is the point on a stress-strain curve that indicates the limit of elastic behavior and the beginning plastic behavior. Yield strength or yield stress is the material property defined as the stress at which a material begins to deform plastically. In contrast, the yield point is where nonlinear (elastic + plastic) deformation begins. Before the yield point, the material will deform elastically and return to its original shape when the applied stress is removed. Once the yield point is passed, some fraction of the deformation will be permanent and non-reversible. Some steels and other materials exhibit a behavior termed a yield point phenomenon. Yield strengths vary from 35 MPa for low-strength aluminum to greater than 1400 MPa for high-strength steel.- Ultimate tensile strength. The ultimate tensile strength is the maximum on the engineering stress-strain curve. This corresponds to the maximum stress sustained by a structure in tension. Ultimate tensile strength is often shortened to “tensile strength” or “the ultimate.” If this stress is applied and maintained, a fracture will result. Often, this value is significantly more than the yield stress (as much as 50 to 60 percent more than the yield for some types of metals). When a ductile material reaches its ultimate strength, it experiences necking where the cross-sectional area reduces locally. The stress-strain curve contains no higher stress than the ultimate strength. Even though deformations can continue to increase, the stress usually decreases after achieving the ultimate strength. It is an intensive property; therefore, its value does not depend on the size of the test specimen. However, it depends on other factors, such as the preparation of the specimen, the presence or otherwise of surface defects, and the temperature of the test environment and material. Ultimate tensile strengths vary from 50 MPa for aluminum to as high as 3000 MPa for very high-strength steel.
- Fracture point: The fracture point is the point of strain where the material physically separates. At this point, the strain reaches its maximum value, and the material actually fractures, even though the corresponding stress may be less than the ultimate strength at this point. Ductile materials have a fracture strength lower than the ultimate tensile strength (UTS), whereas, in brittle materials, the fracture strength is equivalent to the UTS. If a ductile material reaches its ultimate tensile strength in a load-controlled situation, it will continue to deform, with no additional load application, until it ruptures. However, if the loading is displacement-controlled, the deformation of the material may relieve the load, preventing rupture.
In many situations, the yield strength is used to identify the allowable stress to which a material can be subjected. This criterion is inadequate for components that withstand high pressures, such as those used in pressurized water reactors (PWRs). The maximum shear stress theory of failure has been incorporated into the ASME (The American Society of Mechanical Engineers) Boiler and Pressure Vessel Code, Section III, Rules for Construction of Nuclear Pressure Vessels to cover these situations. This theory states that failure of a piping component occurs when the maximum shear stress exceeds the shear stress at the yield point in a tensile test.
Ductile vs. Brittle – Stress-strain curves
Some materials break very sharply, without plastic deformation, in a brittle failure. Others, which are more ductile, including most metals, experience some plastic deformation and possibly necking before fracture. It is possible to distinguish some common characteristics among the stress-strain curves of various groups of materials. On this basis, it is possible to divide materials into two broad categories; namely:
- Ductile Materials. Ductility is the ability of a material to be elongated in tension. Ductile material will deform (elongate) more than brittle material. Ductile materials show large deformation before fracture. In ductile fracture, extensive plastic deformation (necking) takes place before fracture. Ductile fracture (shear fracture) is better than brittle fracture because there is slow propagation and an absorption of a large amount of energy before fracture. Ductility is desirable in the high temperature and high-pressure applications in reactor plants because of the added stresses on the metals. High ductility in these applications helps prevent brittle fracture.
- Brittle Materials. When subjected to stress, brittle materials break with little elastic deformation and without significant plastic deformation. Brittle materials absorb relatively little energy before fracture, even high-strength materials. In brittle fracture (transgranular cleavage), no apparent plastic deformation occurs before fracture, and cracks propagate rapidly.
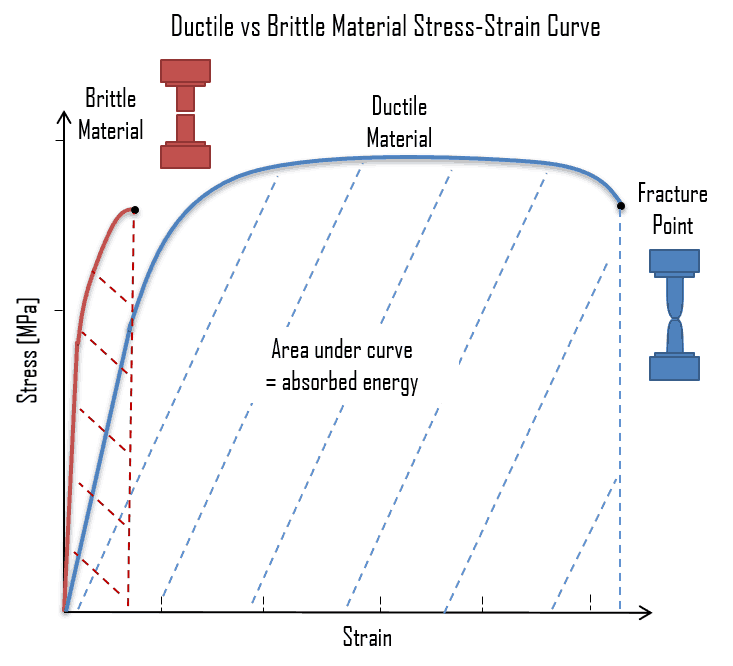 The following figure shows a typical stress-strain curve of ductile and brittle materials. Ductile material is a material with a small strength, and the plastic region is great. The material will bear more strain (deformation) before fracture. A brittle material is a material where the plastic region is small, and the strength of the material is high. The tensile test supplies three descriptive facts about a material. These are the stress at which observable plastic deformation or “yielding” begins; the ultimate tensile strength or maximum intensity of load that can be carried in tension; and the percent elongation or strain (the amount the material will stretch) and the accompanying percent reduction of the cross-sectional area caused by stretching. The rupture or fracture point can also be determined.
The following figure shows a typical stress-strain curve of ductile and brittle materials. Ductile material is a material with a small strength, and the plastic region is great. The material will bear more strain (deformation) before fracture. A brittle material is a material where the plastic region is small, and the strength of the material is high. The tensile test supplies three descriptive facts about a material. These are the stress at which observable plastic deformation or “yielding” begins; the ultimate tensile strength or maximum intensity of load that can be carried in tension; and the percent elongation or strain (the amount the material will stretch) and the accompanying percent reduction of the cross-sectional area caused by stretching. The rupture or fracture point can also be determined.