Detection of gamma radiation is very specific because gamma rays interact differently with the matter. Gamma rays can travel thousands of feet in the air and easily pass through various materials. Moreover, gamma rays can ionize atoms indirectly and directly (despite they are electrically neutral) through the photoelectric effect and the Compton effect. But secondary (indirect) ionization is much more significant.
To describe the principles of detecting gamma radiation, we must understand the interaction of radiation with matter, and each type of particle interacts differently. Therefore, we must describe interactions of gamma rays (radiation as a flow of these rays) separately.
Interaction of Gamma Radiation with Matter
Gamma rays consist of high-energy photons. These photons are particles/waves (Wave-Particle Duality) without rest mass or electrical charge. They can travel 10 meters or more in the air, which is a long distance compared to alpha or beta particles. However, gamma rays deposit less energy along their paths. Lead, water, and concrete stop gamma radiation. Photons (gamma rays and X-rays) can ionize atoms directly through the Photoelectric effect and the Compton effect, where the relatively energetic electron is produced. The secondary electron will go on to produce multiple ionization events; therefore, the secondary (indirect) ionization is much more significant.
Although many possible interactions are known, there are three key interaction mechanisms with the matter.
The photon is completely absorbed in the photoelectric effect and pair production, while only partial energy is deposited in any given Compton scattering. The probability of photoelectric absorption (dominates at lower gamma rays energies) per unit mass is approximately proportional to:
τ(photoelectric) = constant x ZN/E3.5
where Z is the atomic number, and the exponent n varies between 4 and 5. E is the energy of the incident photon. The probability of Compton scattering per one interaction with an atom increases linearly with atomic number Z because it depends on the number of electrons available for scattering in the target atom. The probability of pair production (dominates at higher gamma rays energies), characterized by cross-section, is a very complicated function based on quantum mechanics. The cross-section generally increases approximately with the square of atomic number (σp ~ Z2) and increases with photon energy, but this dependence is much more complex.
As a result, effective sensitive material for gamma radiation detection is, in most cases, based on the use of materials with two following material properties:
- high-density of material.
- the high atomic number of material (high Z materials)
Detectors of Gamma Radiation
Detectors may also be categorized according to sensitive materials and methods that can be utilized to make a measurement:
Detection of Gamma Radiation using Ionization Chamber

Gamma rays have very little trouble penetrating the metal walls of the chamber. Therefore, ionization chambers may be used to detect gamma radiation and X-rays, collectively known as photons, and for this, the windowless tube is used. Ionization chambers have a uniform response to radiation over a wide range of energies and are the preferred means of measuring high levels of gamma radiation. Some problems are caused by alpha particles being more ionizing than beta particles, and gamma rays, so more current is produced in the ionization chamber region by alpha than beta and gamma. Gamma rays deposit a significantly lower amount of energy into the detector than other particles.
Using a high-pressure gas can further increase the chamber’s efficiency. Typically a pressure of 8-10 atmospheres can be used, and various noble gases are employed. For example, high-pressure xenon (HPXe) ionization chambers are ideal for use in uncontrolled environments, as a detector’s response is uniform over large temperature ranges (20–170°C). The higher pressure results in a greater gas density and thereby a greater chance of collision with the fill gas and ion-pair creation by incident gamma radiation. Because of the increased wall thickness required to withstand this high pressure, only gamma radiation can be detected. These detectors are used in survey meters and for environmental monitoring.
Detection of Gamma Radiation using Geiger Counter

Geiger counter can detect ionizing radiation such as alpha and beta particles, neutrons, and gamma rays using the ionization effect produced in a Geiger–Müller tube, which gives its name to the instrument. The voltage of the detector is adjusted so that the conditions correspond to the Geiger-Mueller region.
The high amplification factor of the Geiger counter is the major advantage over the ionization chamber. The Geiger counter is a much more sensitive device than other chambers. This is often used to detect low-level gamma rays and beta particles.
Detection of Gamma Radiation using Scintillation Counter
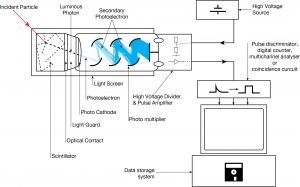
Scintillation counters are used to measure radiation in various applications, including hand-held radiation survey meters, personnel and environmental monitoring for radioactive contamination, medical imaging, radiometric assay, nuclear security, and nuclear plant safety. They are widely used because they can be made inexpensively yet with good efficiency and can measure both the intensity and the energy of incident radiation.
Scintillation counters can be used to detect alpha, beta, and gamma radiation, and they can also be used for the detection of neutrons. For these purposes, different scintillators are used.
- Gamma Rays. High-Z materials are best suited as scintillators for the detection of gamma rays. NaI(Tl) (thallium-doped sodium iodide) is the most widely used scintillation material. The iodine provides most of the stopping power in sodium iodide (since it has a high Z = 53). These crystalline scintillators are characterized by high density, high atomic number, and pulse decay times of approximately 1 microsecond (~ 10-6 sec). Scintillation in inorganic crystals is typically slower than in organic ones. They exhibit high efficiency for the detection of gamma rays and are capable of handling high count rates. Inorganic crystals can be cut to small sizes and arranged in an array configuration to provide position sensitivity. This feature is widely used in medical imaging to detect X-rays or gamma rays. Inorganic scintillators are better at detecting gamma rays and X-rays. This is due to their high density and atomic number, which gives a high electron density.
Detection of Gamma Radiation using Semiconductors – HPGe Detectors

High-purity germanium detectors (HPGe detectors) are the best solution for precise gamma and x-ray spectroscopy.
As was written, the study and analysis of gamma-ray spectra for scientific and technical use are called gamma spectroscopy. Gamma-ray spectrometers are the instruments that observe and collect such data. A gamma-ray spectrometer (GRS) is a sophisticated device for measuring the energy distribution of gamma radiation. For the measurement of gamma rays above several hundred keV, there are two detector categories of major importance, inorganic scintillators such as NaI(Tl) and semiconductor detectors. If a perfect energy resolution is required, we have to use a germanium-based detector, such as the HPGe detector. Germanium-based semiconductor detectors are most commonly used where a very good energy resolution is required, especially for gamma spectroscopy as well as x-ray spectroscopy. In gamma spectroscopy, germanium is preferred due to its atomic number being much higher than silicon, increasing the probability of gamma-ray interaction. Moreover, germanium has lower average energy necessary to create an electron-hole pair, which is 3.6 eV for silicon and 2.9 eV for germanium. This also provides the latter with a better resolution in energy. The FWHM (full width at half maximum) for germanium detectors is an energy function. For a 1.3 MeV photon, the FWHM is 2.1 keV, which is very low.