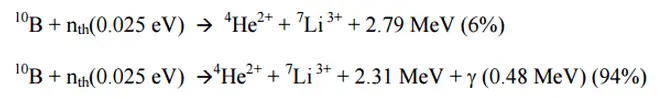A proportional counter, also known as the proportional detector, is an electrical device that detects various types of ionizing radiation. The voltage of the detector is adjusted so that the conditions correspond to the proportional region. In this region, the voltage is high enough to provide the primary electrons with sufficient acceleration and energy to ionize additional atoms of the medium. These secondary ions (gas amplification) formed are also accelerated, causing an effect known as Townsend avalanches, which creates a single large electrical pulse. Gaseous proportional counters usually operate in high electric fields of 10 kV/cm and achieve typical amplification factors of about 105. Since the amplification factor strongly depends on the applied voltage, the charge collected (output signal) also depends on the applied voltage, and proportional counters require constant voltage.
This is a subtle but important difference between ionization chambers and proportional counters. An ionization chamber will produce a current proportional to the number of electrons collected each second. This current is averaged and is used to drive a display reading in Bq, or μSv/h. Proportional counters do not work in this way. Instead, they amplify each of the individual bursts of ionization so that each ionizing event is detected separately. They, therefore, measure the number of ionizing events (which is why they are called counters).
The charge amplification process greatly improves the detector’s signal-to-noise ratio and reduces the subsequent electronic amplification required. When instruments are operated in the proportional region, the voltage must be kept constant, and if a voltage remains constant, the gas amplification factor also does not change. Proportional counter detection instruments are very sensitive to low levels of radiation. The proportional counter can detect alpha, beta, gamma, or neutron radiation in mixed radiation fields by proper functional arrangements, modifications, modifications, and biases. Moreover, proportional counters are capable of particle identification and energy measurement (spectroscopy). The pulse height reflects the energy deposited by the incident radiation in the detector gas. It is possible to distinguish the larger pulses produced by alpha particles from the smaller pulses produced by beta particles or gamma rays.
While ionization chambers can be operated in current or pulse mode, proportional counters or Geiger counters are almost always used in pulse mode. Detectors of ionizing radiation can be used both for activity measurements as well as for dose measurements. The dose can be obtained with knowledge about the energy needed to form a pair of ions.
Argon and helium are the most frequently used fill gases and allow the detection of alpha, beta, and gamma radiation. He-3 and BF3 (Boron Trifluoride) are the most commonly employed for neutron detection. For special purposes, other mixtures of gases have been used, such as a tissue-equivalent gas mixture consisting of 64.4% methane, 32.4% carbon dioxide, and 3.2% nitrogen.
See also: Advantages and Disadvantages of Proportional Counters
In the proportional region, the charge collected increases with a further increase in the detector voltage, while the number of primary ion pairs remains unchanged. Increasing the voltage provides the primary electrons with sufficient acceleration and energy to ionize additional atoms of the medium. These secondary ions formed are also accelerated, causing an effect known as Townsend avalanches, which creates a single large electrical pulse. Even though there is a large number of secondary ions (about 103 – 105) for each primary event, the chamber is always operated such that the number of secondary ions is proportional to the number of primary events. It is very important because the primary ionization is dependent on the type and energy of the particles or rays in the intercepted radiation field. The number of ion pairs collected divided by the number of ion pairs produced by the primary ionization provides the gas amplification factor (denoted by A). The gas amplification in this region can increase the total amount of ionization to a measurable value. The charge amplification process greatly improves the detector’s signal-to-noise ratio and reduces the subsequent electronic amplification required. The voltage must be kept constant when instruments are operated in the proportional region. If a voltage remains constant, the gas amplification factor also does not change. Proportional counter detection instruments are very sensitive to low levels of radiation. Moreover, proportional counters are capable of particle identification and energy measurement (spectroscopy). Different radiation energies and different radiation types can be distinguished by analyzing the pulse height since they significantly differ in the primary ionization.
Basic Principle of Proportional Counters
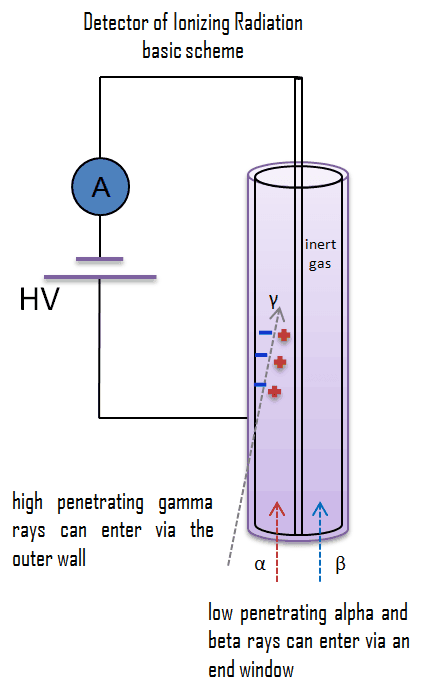
The proportional counter has a cathode and an anode that are held at some voltage (above 1000 V), and the device is characterized by a capacitance that is determined by the geometry of the electrodes. In a proportional counter, the fill gas of the chamber is an inert gas that is ionized by incident radiation and a quench gas to ensure each pulse discharge terminates; a common mixture is 90% argon and 10% methane, known as P-10.
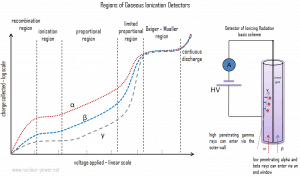
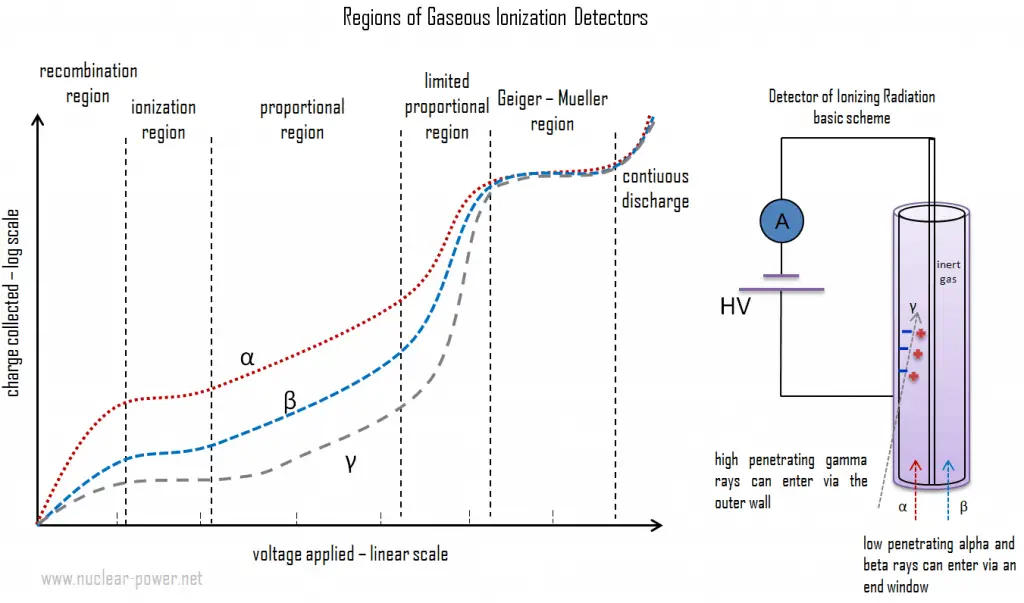
As ionizing radiation enters the gas between the electrodes, a finite number of ion pairs are formed. The average energy needed to produce an ion in the air is about 34 eV. Therefore a 1 MeV radiation completely absorbed in the detector produces about 3 x 104 pairs of ions. The behavior of the resultant ion pairs is affected by the potential gradient of the gas’s electric field and the fill gas’s type and pressure. Under the influence of the electric field, the positive ions will move toward the negatively charged electrode (outer cylinder), and the negative ions (electrons) will migrate toward the positive electrode (central wire). The electric field in this region keeps the ions from recombining with the electrons. Near the anode wire, the field strength becomes large enough to produce Townsend avalanches. This avalanche region occurs only fractions of a millimeter from the anode wire, which is of a very small diameter. This purpose is to use the multiplication effect of the avalanche produced by each ion pair. This is the “avalanche” region. A key design goal is that each original ionizing event due to incident radiation produces only one avalanche. Gas amplification factors can range from unity in the ionization region to 103 or 104 in the proportional region. The high amplification factor of the proportional counter is the major advantage over the ionization chamber.
Collecting all these electrons will produce a charge on the electrodes and an electrical pulse across the detection circuit. Each pulse corresponds to one gamma-ray or particle interaction. The pulse height is proportional to the number of original electrons produced. But in this case, the pulse height is significantly amplified by the detector. The proportionality factor, in this case, is the gas amplification factor. The number of electrons produced is proportional to the energy of the incident particle. Therefore, proportional counters are capable of particle identification and energy measurement (spectroscopy). Different radiation energies and different radiation types can be distinguished by analyzing the pulse height since they significantly differ in the primary ionization (low-LET vs. high-LET). Since the process of charge amplification greatly improves the detector’s signal-to-noise ratio, subsequent electronic amplification is usually not required.
Ionization chamber construction differs from the proportional counter. The flat plate design is preferred for ionization chambers, or concentric cylinders may be utilized in the construction to allow for integrating pulses produced by the incident radiation. Proportional counters and Geiger counters usually utilize cylinder and central electrodes. The proportional counter would require such exact control of the electric field between the electrodes that it would not be practical.
Quenching – Proportional Counters
In a proportional counter, the fill gas of the chamber is an inert gas that is ionized by incident radiation and a quench gas to ensure each pulse discharge terminates; a common mixture is 90% argon and 10% methane, known as P-10.
Each electron collected in the chamber has a positively charged gas ion left over. These gas ions are heavy compared to an electron and move much more slowly. Free electrons are much lighter than positive ions. Thus, they are drawn toward the positive central electrode much faster than the positive ions drawn to the chamber wall. The resulting cloud of positive ions near the electrode leads to distortions in gas multiplication. Eventually, the positive ions move from the positively charged central wire to the negatively charged wall and are neutralized by gaining an electron. In the process, some energy is given off, which causes additional ionization of the gas atoms. The electrons produced by this ionization move toward the central wire and are multiplied en route. This charge pulse is unrelated to the radiation to be detected and can set off a series of pulses. In practice, the avalanche’s termination is improved by using “quenching” techniques.
The quenching gas molecules have a weaker affinity for electrons than the chamber gas; therefore, the ionized atoms of the chamber gas readily take electrons from the quenching gas molecules. Thus, the ionized molecules of quenching gas reach the chamber wall instead of the chamber gas. The ionized molecules of the quenching gas are neutralized by gaining an electron, and the energy liberated does not cause further ionization but causes dissociation of the molecule.
Special Reference: U.S. Department of Energy, Instrumentation, and Control. DOE Fundamentals Handbook, Volume 2 of 2. June 1992.
Detection of Alpha, Beta, and Gamma Radiation using Proportional Counter
Proportional counters in the form of large-area planar detectors are used extensively to check for radioactive contamination on personnel, flat surfaces, tools, and clothing items. Proportional counters are normally used to detect alpha and beta particles. They can enable discrimination between them by providing a pulse output proportional to each particle’s energy deposited in the chamber. They have high efficiency for beta but lower for alpha. For alpha and beta particles to be detected by proportional counters, they must be given a thin window. This “end-window” must be thin enough for the alpha and beta particles to penetrate. However, a window of almost any thickness will prevent an alpha particle from entering the chamber. The window is usually made of mica with a density of about 1.5 – 2.0 mg/cm2 to allow low-energy beta particles (e.g., from carbon-14) to enter the detector. The efficiency reduction for alpha is due to the attenuation effect of the end window, though the distance from the surface being checked also has a significant effect. Ideally, a source of alpha radiation should be less than 10mm from the detector due to attenuation in the air.
Gamma rays have very little trouble penetrating the metal walls of the chamber. Therefore, proportional counters may be used to detect gamma radiation and X-rays (thin-walled tubes) collectively known as photons, and for this, the windowless tube is used.
The main drawback to using proportional counters in portable instruments is that they require a very stable power supply and amplifier to ensure constant operating conditions (in the middle of the proportional region). This isn’t easy to provide in a portable instrument, so proportional counters tend to be used more in fixed or lab instruments.
Proportional Counter-Spectroscopy
In general, spectroscopes are devices designed to measure the spectral power distribution of a source. The incident radiation generates a signal that determines the incident particle’s energy.
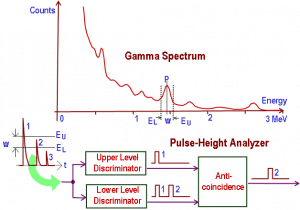
In proportional counters, the number of electrons produced is proportional to the energy and type of the incident particle. Therefore, proportional counters are capable of particle identification and energy measurement (spectroscopy). Different radiation energies and different radiation types can be distinguished by analyzing the pulse height since they significantly differ in the primary ionization (low-LET vs. high-LET). Proportional counters may be used to analyze a spectrum of alpha radiations or a spectrum of beta particles. However, the energy resolution of a proportional counter is limited because both the initial ionization event and the subsequent ‘multiplication’ event are subject to statistical fluctuations characterized by a standard deviation equal to the square root of the average number formed.
A large volume spherical proportional counter can be used for neutron measurements. The pure N2 gas is studied for thermal and fast neutron detection, providing a new way for neutron spectroscopy. The neutrons are detected via the 14N(n,p)14C and 14N(n,α)11B reactions.
For gamma spectrometry, the most common detectors include sodium iodide (NaI) scintillation counters and high-purity germanium detectors.
Multi-wire Proportional Chamber – MWPC
A multi-wire proportional chamber is a type of proportional counter used especially in high-energy particle physics to detect charged particles and photons. It can give positional information on their trajectory. This device was developed by Georges Charpak and his collaborators, and this invention resulted in him winning the Nobel Prize for Physics in 1992. The multi-wire chamber uses an array of wires at high voltage (anode), which run through a chamber with conductive walls held at ground potential (cathode). The principle is to have a plane of anode wires positioned precisely, with typical wire-spacings of about 2 mm. The particle trajectory can be found by computing pulses from all the wires.
Detection of Neutrons using Proportional Counter
Since the neutrons are electrically neutral particles, they are mainly subject to strong nuclear forces but not electric ones. Therefore, neutrons are not directly ionizing and usually have to be converted into charged particles before they can be detected. Generally, every type of neutron detector must be equipped with a converter (to convert neutron radiation to common detectable radiation) and one of the conventional radiation detectors (scintillation detector, gaseous detector, semiconductor detector, etc.).
Proportional counters are often used as the charged particle detection device. Gas-filled (BF3) proportional counters are normally used in nuclear power plants as source range detectors. These detectors use the gas boron trifluoride (BF3) instead of air in the chamber. The incoming neutrons produce alpha particles when reacting with the boron atoms in the detector gas. Most of (n,alpha) reactions of thermal neutrons are 10B(n,alpha)7Li reactions accompanied by 0.48 MeV gamma emission.
Moreover, isotope boron-10 has a high (n, alpha) reaction cross-section along the entire neutron energy spectrum. The alpha particle causes ionization within the chamber, and ejected electrons cause further secondary ionizations.
The proportional counter output is in the form of one pulse for every ionizing event; therefore, there is a series of random pulses varying in magnitude representing neutron and gamma ionizing events. The pulse height may only be a few millivolts, which is too low to be directly used without amplification. The discriminator excludes the passage of pulses that are less than a predetermined level. The function of the discriminator is to exclude noise and gamma pulses that are lower in magnitude than neutron pulses.