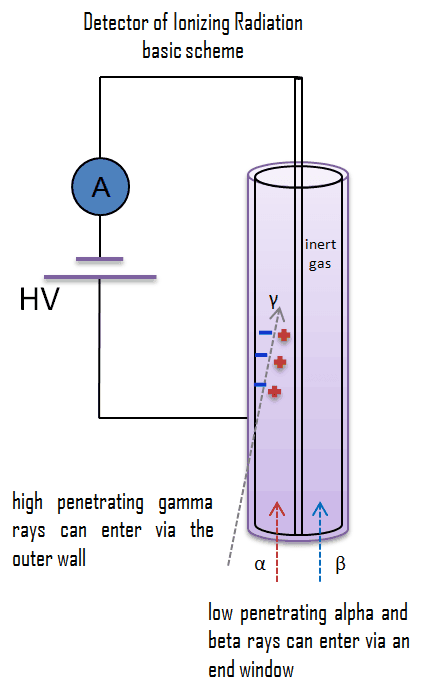
The proportional counter has a cathode and an anode that are held at some voltage (above 1000 V), and the device is characterized by a capacitance determined by the electrodes’ geometry. In a proportional counter, the fill gas of the chamber is an inert gas that is ionized by incident radiation and a quench gas to ensure each pulse discharge terminates; a common mixture is 90% argon and 10% methane, known as P-10.
As ionizing radiation enters the gas between the electrodes, a finite number of ion pairs are formed. The average energy needed to produce an ion in the air is about 34 eV. Therefore a 1 MeV radiation completely absorbed in the detector produces about 3 x 104 pairs of ions. The behavior of the resultant ion pairs is affected by the potential gradient of the electric field within the gas and the type and pressure of the fill gas. Under the influence of the electric field, the positive ions will move toward the negatively charged electrode (outer cylinder), and the negative ions (electrons) will migrate toward the positive electrode (central wire). The electric field in this region keeps the ions from recombining with the electrons. Near the anode wire, the field strength becomes large enough to produce Townsend avalanches. This avalanche region occurs only fractions of a millimeter from the anode wire, which is of a very small diameter. This purpose is to use the multiplication effect of the avalanche produced by each ion pair. This is the “avalanche” region. A key design goal is that each original ionizing event due to incident radiation produces only one avalanche. Gas amplification factors can range from unity in the ionization region to 103 or 104 in the proportional region. The high amplification factor of the proportional counter is the major advantage over the ionization chamber.
Collecting all these electrons will produce a charge on the electrodes and an electrical pulse across the detection circuit. Each pulse corresponds to one gamma-ray or particle interaction. The pulse height is proportional to the number of original electrons produced. But in this case, the pulse height is significantly amplified by the detector. The proportionality factor, in this case, is the gas amplification factor. The number of electrons produced is proportional to the energy of the incident particle. Therefore, proportional counters are capable of particle identification and energy measurement (spectroscopy). Different radiation energies and different radiation types can be distinguished by analyzing the pulse height since they significantly differ in the primary ionization (low-LET vs. high-LET). Since the process of charge amplification greatly improves the detector’s signal-to-noise ratio, subsequent electronic amplification is usually not required.
Ionization chamber construction differs from the proportional counter. The flat plate design is preferred for ionization chambers, or concentric cylinders may be utilized in the construction to allow for integrating pulses produced by the incident radiation. Proportional counters and Geiger counters usually utilize cylinder and central electrodes. The proportional counter would require such exact control of the electric field between the electrodes that it would not be practical.