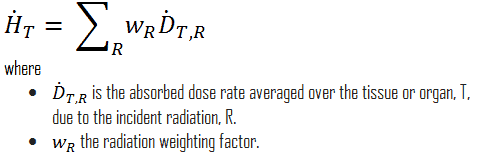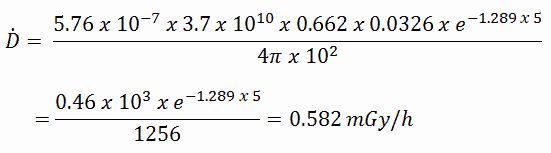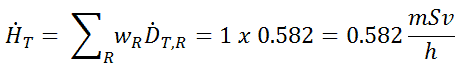 Equivalent dose (symbol HT) is a dose quantity calculated for individual organs (index T – tissue). The equivalent dose is based on the absorbed dose to an organ, adjusted to account for the effectiveness of the type of radiation. An equivalent dose is given the symbol HT. The SI unit of HT is the sievert (Sv) or but rem (roentgen equivalent man) is still commonly used (1 Sv = 100 rem). Unit of sievert was named after the Swedish scientist Rolf Sievert, who did a lot of the early work on dosimetry in radiation therapy.
Equivalent dose (symbol HT) is a dose quantity calculated for individual organs (index T – tissue). The equivalent dose is based on the absorbed dose to an organ, adjusted to account for the effectiveness of the type of radiation. An equivalent dose is given the symbol HT. The SI unit of HT is the sievert (Sv) or but rem (roentgen equivalent man) is still commonly used (1 Sv = 100 rem). Unit of sievert was named after the Swedish scientist Rolf Sievert, who did a lot of the early work on dosimetry in radiation therapy.
As was written, for radiation protection purposes, the absorbed dose is averaged over an organ or tissue, T. This absorbed dose average is weighted for the radiation quality in terms of the radiation weighting factor, wR, for the type and energy of radiation incident on the body. The radiation weighting factor is a dimensionless factor used to determine the equivalent dose from the absorbed dose averaged over a tissue or organ based on the type of radiation absorbed. The resulting weighted dose was designated as the organ- or tissue equivalent dose:
 An equivalent dose of one Sievert represents that quantity of radiation dose that is equivalent to specified biological damage to one gray of X-rays or gamma rays. An equivalent dose is a non-physical quantity (wR is derived from biological consequences of ionizing radiation) widely used in dosimetry measured by dosimeters. An equivalent dose is designated by the ICRP as a “limiting quantity”; to specify exposure limits to ensure that “the occurrence of stochastic health effects is kept below unacceptable levels and those tissue reactions are avoided.”
An equivalent dose of one Sievert represents that quantity of radiation dose that is equivalent to specified biological damage to one gray of X-rays or gamma rays. An equivalent dose is a non-physical quantity (wR is derived from biological consequences of ionizing radiation) widely used in dosimetry measured by dosimeters. An equivalent dose is designated by the ICRP as a “limiting quantity”; to specify exposure limits to ensure that “the occurrence of stochastic health effects is kept below unacceptable levels and those tissue reactions are avoided.”
An equivalent dose, HT, is used for assessing stochastic health risks due to external radiation fields that penetrate uniformly through the whole body. However, it needs further corrections when the field is applied only to part(s) of the body or non-uniformly to measure the overall stochastic health risk to the body. A further dose quantity called effective dose must be used to enable this. Effective dose is defined as the sum of the organ-equivalent doses weighted by the ICRP organ weighting factors, wT, which considers the varying sensitivity of different organs and tissues to radiation.
Units of equivalent dose:
- Sievert. A sievert is a derived unit of equivalent dose and effective dose. It represents the equivalent biological effect of depositing a joule of gamma rays energy in a kilogram of human tissue.
- REM. The rem (an abbreviation for Roentgen Equivalent Man) is the non-SI unit of the equivalent dose used predominantly in the USA. It is a term for dose equivalence and equals the biological damage that would be caused by one rad of dose.
A dose of one Sv caused by gamma radiation is equivalent to an energy deposition of one joule in a kilogram of tissue. That means one sievert is equivalent to one gray of gamma rays deposited in certain tissue. On the other hand, similar biological damage (one sievert) can be caused only by 1/20 gray of alpha radiation.
One sievert is a large amount of equivalent dose. A person who has absorbed a whole-body dose of 1 Sv has absorbed one joule of energy in each kg of body tissue (in case of gamma rays).
Equivalent doses measured in industry and medicine often have usually lower doses than one sievert, and the following multiples are often used:
1 mSv (millisievert) = 1E-3 Sv
1 µSv (microsievert) = 1E-6 Sv
Conversions from the SI units to other units are as follows:
- 1 Sv = 100 rem
- 1 mSv = 100 mrem
From Absorbed Dose to Equivalent Dose
Note that the sievert is not a physical dose unit. For example, an absorbed dose of 1 Gy by alpha particles will lead to an equivalent dose of 20 Sv. This may seem to be a paradox. It implies that the energy of the incident radiation field in joules has increased by a factor of 20, thereby violating the laws of Conservation of energy. However, this is not the case. Sievert is derived from the physical quantity absorbed dose but also considers the biological effectiveness of the radiation, which is dependent on the radiation type and energy. The radiation weighting factor causes the sievert cannot be a physical unit.
As was written, each type of radiation interacts with matter in a different way and causes different biological damages. For example, charged particles with high energies can directly ionize atoms. On the other hand, electrically neutral particles interact only indirectly but can also transfer some or all of their energies to the matter. It would certainly simplify matters if the biological effects of radiation were directly proportional to the absorbed dose. Unfortunately, biological effects depend also on the way in which the absorbed dose is distributed along the path of the radiation. Studies have shown that alpha and neutron radiation cause greater biological damage for a given energy deposition per kg of tissue than gamma radiation. Biological effects of any radiation increase with the linear energy transfer (LET) were discovered. In short, the biological damage from high-LET radiation (alpha particles, protons, or neutrons) is much greater than that from low-LET radiation (gamma rays). This is because the living tissue can more easily repair damage from radiation spread over a large area than that concentrated in a small area. Because more biological damage is caused for the same physical dose (i.e., the same energy deposited per unit mass of tissue), one gray of alpha or neutron radiation is more harmful than one gray of gamma radiation. The fact that radiations of different types (and energies) give different biological effects for the same absorbed dose is described in terms of factors known as the relative biological effectiveness (RBE) and the radiation weighting factor (wR).
Radiation Weighting Factor
In radiation protection, the radiation weighting factor is a dimensionless factor used to determine the equivalent dose from the absorbed dose averaged over a tissue or organ based on the type of radiation absorbed. In the past, a similar factor known as the quality factor was used for this purpose. The radiation weighting factor estimates the effectiveness per unit dose of the given radiation relative to a low-LET standard.
Before 1990, dose-equivalent quantities were defined in terms of a quality factor, Q(L), that was applied to the absorbed dose at a point to take into account the differences in the effects of different types of radiation. In its 1990 recommendations, the ICRP introduced a modified concept. For radiological protection purposes, the absorbed dose is averaged over an organ or tissue, T. This absorbed dose average is weighted for the radiation quality in terms of the radiation weighting factor, wR, for the type and energy of radiation incident on the body.
The reason for replacing the quality factor, i.e., the Q–L relationship, with wR values in the definition of the organ-equivalent doses and the effective dose was that the Commission believed:
‘that the detail and precision inherent in using a formal Q–L relationship to modify absorbed dose to reflect the higher probability of detriment resulting from exposure to radiation components with high LET is not justified because of the uncertainties in the radiological information.’
Noteworthy, these two factors, the radiation weighting factor and the quality factor, are restricted to the dose range of interest to radiation protection, i.e., to the general magnitude of the dose limits. In special circumstances where one deals with higher doses that can cause deterministic effects, the relevant RBE values are applied to obtain a weighted dose.
Special Reference: ICRP, 2003. Relative Biological Effectiveness (RBE), Quality Factor (Q), and Radiation Weighting Factor (wR). ICRP Publication 92. Ann. ICRP 33 (4).
Radiation Weighting Factors – ICRP
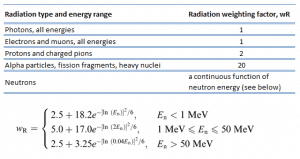
For photon and electron radiation, the radiation weighting factor has the value of 1, independently of the energy of the radiation, and for alpha radiation, the value is 20. For neutron radiation, the value is energy-dependent and amounts to 5 to 20.
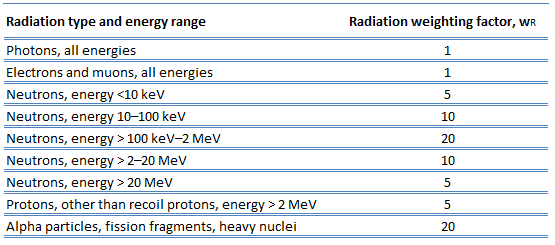
In 2007 ICRP published a new set of radiation weighting factors (ICRP Publ. 103: The 2007 Recommendations of the International Commission on Radiological Protection). These factors are given below.
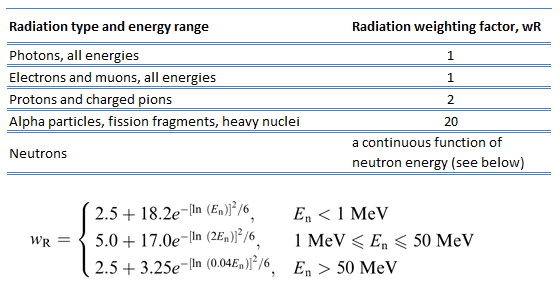
As shown in the table, a wR of 1 is for all low-LET radiations, i.e., X-rays and gamma rays of all energies as well as electrons and muons. A smooth curve, considered an approximation, was fitted to the wR values as a function of incident neutron energy. Note that En is the neutron energy in MeV.
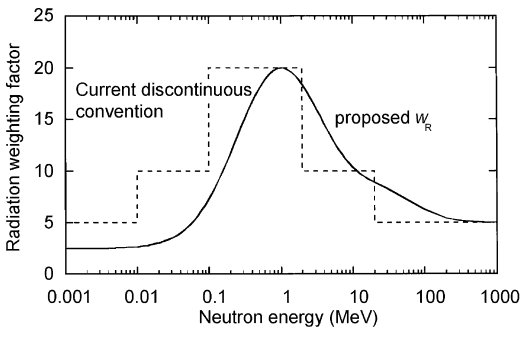
Thus, for example, an absorbed dose of 1 Gy by alpha particles will lead to an equivalent dose of 20 Sv, and an equivalent dose of radiation is estimated to have the same biological effect as an equal amount of absorbed dose of gamma rays, which is given a weighting factor of 1.
Quality Factor
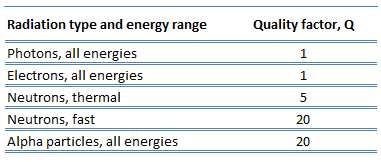 The quality factor of a radiation type is defined as the ratio of the biological damage produced by the absorption of 1 Gy of that radiation to the biological damage produced by 1 Gy of X-rays or gamma rays.
The quality factor of a radiation type is defined as the ratio of the biological damage produced by the absorption of 1 Gy of that radiation to the biological damage produced by 1 Gy of X-rays or gamma rays.
The Q of a certain type of radiation is related to the density of the ion tracks it leaves behind in tissue. The quality factors for the various types of radiation are listed in the table.
These quality factors are restricted to the dose range of interest to radiation protection, i.e., to the general magnitude of the dose limits. In special circumstances where one deals with higher doses that can cause deterministic effects, the relevant RBE values are applied to obtain a weighted dose.
Examples of Doses in Sieverts
We must note that radiation is all around us. In, around, and above the world we live in. It is a natural energy force that surrounds us, and it is a part of our natural world that has been here since the birth of our planet. In the following points, we try to express enormous ranges of radiation exposure, which can be obtained from various sources.
- 0.05 µSv – Sleeping next to someone
- 0.09 µSv – Living within 30 miles of a nuclear power plant for a year
- 0.1 µSv – Eating one banana
- 0.3 µSv – Living within 50 miles of a coal power plant for a year
- 10 µSv – Average daily dose received from natural background
- 20 µSv – Chest X-ray
- 40 µSv – A 5-hour airplane flight
- 600 µSv – mammogram
- 1 000 µSv – Dose limit for individual members of the public, total effective dose per annum
- 3 650 µSv – Average yearly dose received from natural background
- 5 800 µSv – Chest CT scan
- 10 000 µSv – Average yearly dose received from a natural background in Ramsar, Iran
- 20 000 µSv – single full-body CT scan
- 175 000 µSv – Annual dose from natural radiation on a monazite beach near Guarapari, Brazil.
- 5 000 000 µSv – Dose that kills a human with a 50% risk within 30 days (LD50/30) if the dose is received over a very short duration.
As can be seen, low-level doses are common in everyday life. The previous examples can help illustrate relative magnitudes. From biological consequences, it is very important to distinguish between doses received over short and extended periods. An “acute dose” occurs over a short and finite period, while a “chronic dose” is a dose that continues for an extended period so that a dose rate better describes it. High doses tend to kill cells, while low doses tend to damage or change them. Low doses spread out over long periods don’t cause an immediate problem to any body organ. The effects of low radiation doses occur at the cell level, and the results may not be observed for many years.
Equivalent Dose Rate
The equivalent dose rate is the rate at which an equivalent dose is received and a measure of radiation dose intensity (or strength). The equivalent dose rate is therefore defined as:
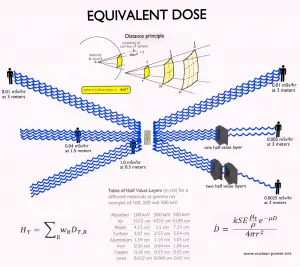
In conventional units, it is measured in mSv/sec, Sv/hr, mrem/sec or rem/hr. Since the amount of radiation exposure depends directly (linearly) on the time people spend near the source of radiation, the absorbed dose is equal to the strength of the radiation field (dose rate) multiplied by the length of time spent in that field. The example above indicates a person could expect to receive a dose of 25 millirems by staying in a 50 millirems/hour field for thirty minutes.
Calculation of Shielded Dose Rate
Assume the point isotropic source contains 1.0 Ci of 137Cs and has a half-life of 30.2 years. Note that the relationship between half-life and the amount of a radionuclide required to give an activity of one curie is shown below. This amount of material can be calculated using λ, which is the decay constant of certain nuclide:
About 94.6 percent decays by beta emission to a metastable nuclear isomer of barium: barium-137m. The main photon peak of Ba-137m is 662 keV. For this calculation, assume that all decays go through this channel.
Calculate the primary photon dose rate, in gray per hour (Gy.h-1), at the outer surface of a 5 cm thick lead shield. Then calculate the equivalent dose rate. Assume that this external radiation field penetrates uniformly through the whole body. The primary photon dose rate neglects all secondary particles. Assume that the effective distance of the source from the dose point is 10 cm. We shall also assume that the dose point is soft tissue and it can reasonably be simulated by water, and we use the mass-energy absorption coefficient for water.
See also: Gamma Ray Attenuation
See also: Shielding of Gamma Rays
Solution:
The primary photon dose rate is attenuated exponentially, and the dose rate from primary photons, taking account of the shield, is given by:
As can be seen, we do not account for the buildup of secondary radiation. If secondary particles are produced, or the primary radiation changes its energy or direction, the effective attenuation will be much less. This assumption generally underestimates the true dose rate, especially for thick shields and when the dose point is close to the shield surface, but this assumption simplifies all calculations. For this case, the true dose rate (with the buildup of secondary radiation) will be more than two times higher.
To calculate the absorbed dose rate, we have to use the formula:
- k = 5.76 x 10-7
- S = 3.7 x 1010 s-1
- E = 0.662 MeV
- μt/ρ = 0.0326 cm2/g (values are available at NIST)
- μ = 1.289 cm-1 (values are available at NIST)
- D = 5 cm
- r = 10 cm
Result:
The resulting absorbed dose rate in grays per hour is then:
Since the radiation weighting factor for gamma rays is equal to one, and we have assumed the uniform radiation field, we can directly calculate the equivalent dose rate from the absorbed dose rate as:
If we want to account for the buildup of secondary radiation, then we have to include the buildup factor. The extended formula for the dose rate is then:
——–