Radiation hormesis is a dose-response phenomenon. One of the alternative hypotheses is that low doses of ionizing radiation induce beneficial health effects. According to the radiation hormesis hypothesis, low doses of low LET radiation can stimulate the activation of repair mechanisms that protect against diseases that are not activated in the absence of ionizing radiation. A low dose means additional small doses comparable to the normal background radiation (10 µSv = average daily dose received from natural background). Since, at high doses, the negative effects are irrefutable, a threshold must exist between the beneficial and the negative effects of radiation. This threshold is known as the Zero Equivalent Point (ZEP).
The radiation hormesis hypothesis proposes that radiation exposure comparable to and just above the natural background level of radiation is not harmful but beneficial while accepting that much higher radiation levels are hazardous. Arguments for hormesis center around some large-scale epidemiological studies and the evidence from animal irradiation experiments, but most notably the recent advances in knowledge of the adaptive response. Proponents of radiation hormesis typically claim that radio-protective responses in cells and the immune system not only counter radiation’s harmful effects but also inhibit spontaneous cancer not related to radiation exposure.
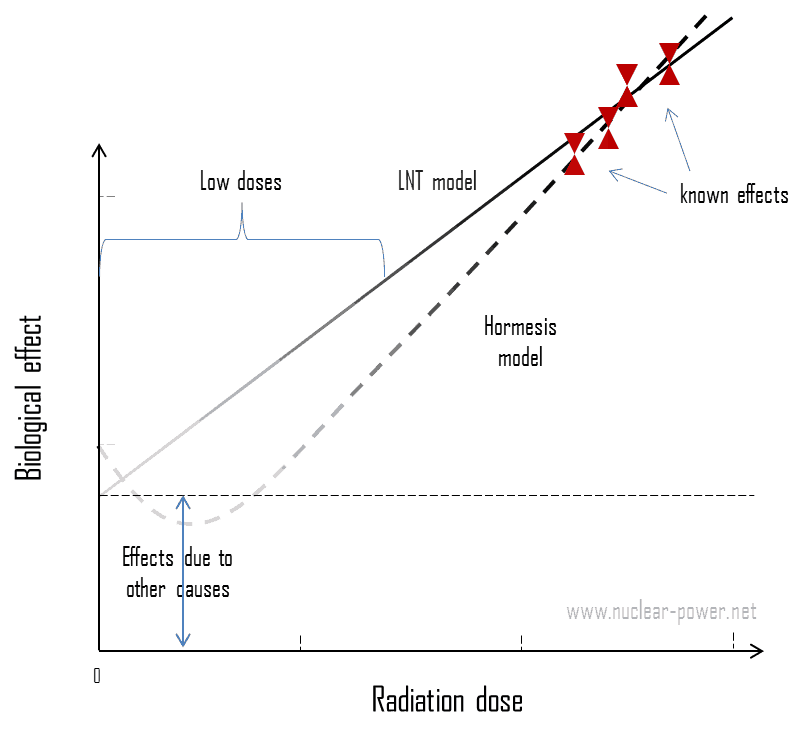
The controversy of the LNT Model
As was written previously (LNT model), today, the protection system is based on the LNT hypothesis, a conservative model used in radiation protection to estimate the health effects of small radiation doses. This model is excellent for setting up a protection system for all use of ionizing radiation. Compared to the hormesis model, the LNT model assumes that there is no threshold point and risk increases linearly with a dose, i.e., the LNT model implies that there is no safe dose of ionizing radiation. If this linear model is correct, natural background radiation is the most hazardous radiation source to general public health, followed by medical imaging as a close second.
The LNT model is primarily based on the life span study (LSS) of atomic bomb survivors in Japan. However, while this pattern is undisputed at high doses, this linear extrapolation of risk to low doses is challenged by many recent experiments involving cell mechanisms. There is also high uncertainty involved in estimating risk using only epidemiological studies. The problem is that, at very low doses, it is practically impossible to correlate any irradiation with certain biological effects. This is because the baseline cancer rate is already very high, and the risk of developing cancer fluctuates by 40% because of individual lifestyle and environmental effects, obscuring the subtle effects of low-level radiation. Government and regulatory bodies assume an LNT model instead of a threshold or hormesis not because it is the more scientifically convincing but because it is, the more conservative estimate.
In the case of low doses, its conservativeness (linearity) has enormous consequences. The model is sometimes wrongly (perhaps intentionally) used to quantify the cancerous effect of collective doses of low-level radioactive contaminations. A linear dose-effect curve makes it possible to use collective doses to calculate the detrimental effects on an irradiated population. It is also argued that the LNT model had caused an irrational fear of radiation since every microsievert can be converted to the probability of cancer induction, however small this probability is. For example, if ten million people receive an effective dose of 0.1 µSv (the equivalent of eating one banana), then the collective dose will be S = 1 Sv. Does it mean there is a 5.5% chance of developing cancer for one person due to eating a banana? Note that, for high doses, one sievert represents a 5.5% chance of developing cancer.
The problem with this model is that it neglects many defense biological processes that may be crucial at low doses. The research during the last two decades is very interesting and shows that small doses of radiation given at a low dose rate stimulate the defense mechanisms. Therefore the LNT model is not universally accepted, with some proposing an adaptive dose-response relationship where low doses are protective, and high doses are detrimental. Many studies have contradicted the LNT model, and many have shown adaptive response to low dose radiation resulting in reduced mutations and cancers.