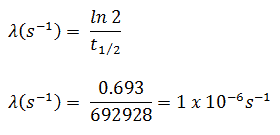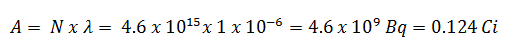
As was written, radioactivity (activity of certain radionuclides) is when an unstable nucleus spontaneously and randomly disintegrates to form a different nucleus (or a different energy state – gamma decay), giving off radiation in the form of atomic particles or high energy rays. This decay occurs at a constant, predictable rate referred to as decay constant. As a result, a measure of radioactivity is based on counting disintegrations per second. The activity depends only on the number of decays per second, not on the type of decay, the energy of the decay products, or the biological effects of the radiation. It can be used to characterize the rate of emission of ionizing radiation.
Units of radioactivity (the curie and the becquerel) can also be used to characterize an overall quantity of controlled or accidental releases of radioactive atoms. Because the probability of decay is a fixed physical quantity, for a known number of atoms of a particular radionuclide, a predictable number will decay in a given time. For example, according to the Nuclear Safety Commission (NSC) of Japan, the total amounts of activity released during the Fukushima Daiichi accident between 11 March and 5 April were about 130 PBq (petabecquerels, 3.5 megacuries) for iodine-131 and 11 PBq for cesium-137.
According to the UNSCEAR, from 1998 to 2002, the global annual average tritium releases to the atmosphere and the aqueous environment from nuclear facilities were estimated to be 11.7 PBq and 16.0 PBq, respectively. Note that tritium emits low-energy beta particles with a short range in body tissues and, therefore, poses a risk to health due to internal exposure only following ingestion in drinking water or food, or inhalation or absorption through the skin. According to the ICRP, a biological half-time of tritium is 10 days for HTO and 40 days for OBT (organically bound tritium) formed from HTO in the body of adults.
See also: SOURCES, EFFECTS, AND RISKS OF IONIZING RADIATION, UNSCEAR 2016, ISBN: 978-92-1-142316-7.
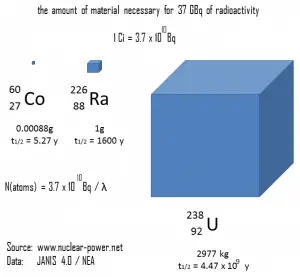
Historically, the original unit for measuring the amount of radioactivity was the curie (symbol Ci), a non-SI unit of radioactivity defined in 1910. The SI unit for measuring the amount of radioactivity is the becquerel (symbol Bq). The becquerel is named in honor of Henri Becquerel. Rutherford (symbol Rd) is also a non-SI unit defined as the activity of a quantity of radioactive material in which one million nuclei decay per second. This unit was introduced in 1946, but the rutherford became obsolete after the becquerel was introduced in 1975.
Example – Calculation of Radioactivity
 A sample of material contains 1 microgram of iodine-131. Note that iodine-131 plays a major role as a radioactive isotope present in nuclear fission products and is a major contributor to the health hazards when released into the atmosphere during an accident. Iodine-131 has a half-life of 8.02 days.
A sample of material contains 1 microgram of iodine-131. Note that iodine-131 plays a major role as a radioactive isotope present in nuclear fission products and is a major contributor to the health hazards when released into the atmosphere during an accident. Iodine-131 has a half-life of 8.02 days.
Calculate:
- The number of iodine-131 atoms is initially present.
- The activity of the iodine-131 in curies.
- The number of iodine-131 atoms will remain in 50 days.
- The time it will take for the activity to reach 0.1 mCi.
Solution:
- The number of atoms of iodine-131 can be determined using isotopic mass as below.
NI-131 = mI-131 . NA / MI-131
NI-131 = (1 μg) x (6.02×1023 nuclei/mol) / (130.91 g/mol)
NI-131 = 4.6 x 1015 nuclei
- The activity of the iodine-131 in curies can be determined using its decay constant:
The iodine-131 has a half-life of 8.02 days (692928 sec), and therefore its decay constant is:
Using this value for the decay constant, we can determine the activity of the sample:
3) and 4) The number of iodine-131 atoms that will remain in 50 days (N50d) and the time it will take for the activity to reach 0.1 mCi can be calculated using the decay law:
As can be seen, after 50 days, the number of iodine-131 atoms and thus the activity will be about 75 times lower. After 82 days, the activity will be approximately 1200 times lower. Therefore, the time of ten half-lives (factor 210 = 1024) is widely used to define residual activity.