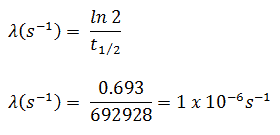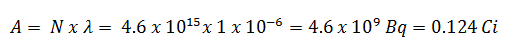The SI unit for measuring the amount of radioactivity is the becquerel (symbol Bq). The becquerel is named in honor of Henri Becquerel, a French physicist who discovered radioactivity in 1896. One becquerel (1Bq) is equal to 1 disintegration per second.
An older unit of radioactivity is the curie. The curie was originally defined as equivalent to the number of disintegrations one gram of radium-226 will undergo in one second. Currently, a curie is defined using becquerels as 1Ci = 3.7 x 1010 disintegrations per second. Therefore
1Ci = 3.7 x 1010 Bq = 37 GBq
One becquerel is a very small amount of activity. The human body contains roughly 3.7 kBq (14 mg) of naturally occurring potassium-40. A human body containing 16 kg of carbon would also have about 3.7 kBq of carbon-14 (24 nanograms). Activities measured in a nuclear power plant (except irradiated fuel) often have usually higher activity than becquerel, and the following multiples are often used:
1 kBq (kilobecquerel) = 1E3 Bq
1 MBq (megabecquerel) = 1E6 Bq
1 GBq (gigabecquerel) = 1E9 Bq
1 TBq (terabecquerel) = 1E12 Bq
Becquerel – Examples
The relationship between half-life and the amount of a radionuclide required to give an activity of 37 GBq (1 Ci) is shown in the figure. This amount of material can be calculated using λ, which is the decay constant of certain nuclide:
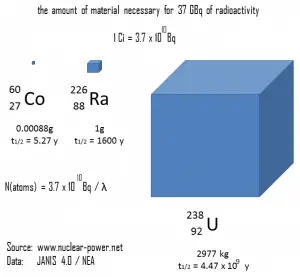 The following figure illustrates the amount of material necessary for 37 GBq of radioactivity. Obviously, the longer the half-life, the greater the quantity of radionuclide needed to produce the same activity. Of course, the longer-lived substance will remain radioactive for much longer. As can be seen, the amount of material necessary for 37 GBq of radioactivity can vary from an amount too small to be seen (0.00088 gram of cobalt-60), through 1 gram of radium-226, to almost three tons of uranium-238.
The following figure illustrates the amount of material necessary for 37 GBq of radioactivity. Obviously, the longer the half-life, the greater the quantity of radionuclide needed to produce the same activity. Of course, the longer-lived substance will remain radioactive for much longer. As can be seen, the amount of material necessary for 37 GBq of radioactivity can vary from an amount too small to be seen (0.00088 gram of cobalt-60), through 1 gram of radium-226, to almost three tons of uranium-238.
Example – Calculation of Radioactivity
 A sample of material contains 1 microgram of iodine-131. Note that iodine-131 plays a major role as a radioactive isotope present in nuclear fission products and is a major contributor to the health hazards when released into the atmosphere during an accident. Iodine-131 has a half-life of 8.02 days.
A sample of material contains 1 microgram of iodine-131. Note that iodine-131 plays a major role as a radioactive isotope present in nuclear fission products and is a major contributor to the health hazards when released into the atmosphere during an accident. Iodine-131 has a half-life of 8.02 days.
Calculate:
- The number of iodine-131 atoms is initially present.
- The activity of the iodine-131 in curies.
- The number of iodine-131 atoms will remain in 50 days.
- The time it will take for the activity to reach 0.1 mCi.
Solution:
- The number of atoms of iodine-131 can be determined using isotopic mass as below.
NI-131 = mI-131 . NA / MI-131
NI-131 = (1 μg) x (6.02×1023 nuclei/mol) / (130.91 g/mol)
NI-131 = 4.6 x 1015 nuclei
- The activity of the iodine-131 in curies can be determined using its decay constant:
The iodine-131 has a half-life of 8.02 days (692928 sec), and therefore its decay constant is:
Using this value for the decay constant, we can determine the activity of the sample:
3) and 4) The number of iodine-131 atoms that will remain in 50 days (N50d) and the time it will take for the activity to reach 0.1 mCi can be calculated using the decay law:
As can be seen, after 50 days, the number of iodine-131 atoms and thus the activity will be about 75 times lower. After 82 days, the activity will be approximately 1200 times lower. Therefore, the time of ten half-lives (factor 210 = 1024) is widely used to define residual activity.