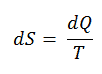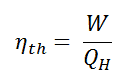Second Law of Thermodynamics

The direction of Thermodynamic Processes

Many thermodynamic processes proceed naturally in one direction but not the opposite. For example, when a temperature difference does exist, heat flows spontaneously from the warmer system to the colder system, never the reverse. In fact, such heat flow (from a colder body to a warmer system) would not violate the first law of thermodynamics, i.e., energy would be conserved. But it doesn’t happen in nature.

For example, burning gasoline to power cars is an energy conversion process we rely on. The chemical energy in gasoline is converted to thermal energy, then converted to mechanical energy that makes the car move. The mechanical energy has been converted to kinetic energy. When we use the brakes to stop a car, that kinetic energy is converted by friction back to heat or thermal energy. In this reverse direction, there are plenty of devices that convert heat partially into mechanical energy. But you cannot build a machine that converts heat completely into mechanical energy. There will always be significant energy losses.

Directions of thermodynamic processes are subject to the second law of thermodynamics, especially of the Clausius Statement of the Second Law.

Various Statements of the Law

The second law of thermodynamics may be expressed in many specific ways. Each statement expresses the same law. Listed below are three that are often encountered.

• Clausius Statement
• Kelvin-Planck Statement
• Entropy and the Second Law

Before these statements, we have to remind the French engineer and physicist, Nicolas Léonard Sadi Carnot, who advanced the study of the second law by forming a principle (also called Carnot’s rule) that specifies limits on the maximum efficiency of any heat engine can obtain.

Clausius Statement of the Second Law

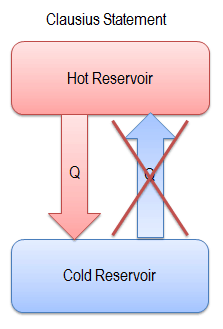One of the earliest statements of the Second Law of Thermodynamics was made by R. Clausius in 1850. He stated the following.

“It is impossible to construct a device which operates on a cycle and whose sole effect is the transfer of heat from a cooler body to a hotter body”.

Heat cannot spontaneously flow from cold system to hot system without external work being performed on the system. This is exactly what refrigerators and heat pumps accomplish. In a refrigerator, heat flows from cold to hot, but only when forced by external work. Refrigerators are driven by electric motors requiring work from their surroundings to operate.

The Clausius and the Kelvin-Planck statements are equivalent.

Kelvin-Planck Statement of the Second Law

“It is impossible to construct a device which operates on a cycle and produces no other effect than the production of work and the transfer of heat from a single body”.

This statement operates with the term “thermal reservoir” or “single reservoir”.  A reservoir is a large object in which the temperature remains constant while energy is extracted. Such a system can be approximated in many ways—by the earth’s atmosphere, large bodies of water like lakes, oceans, and so on.

The Kelvin–Planck statement does not exclude the existence of a system that develops a net amount of work from a heat transfer extracted from a thermal reservoir. According to this statement, a system undergoing a cycle cannot develop a positive net amount of work from a heat transfer extracted from a thermal reservoir.

Entropy and the Second Law

One consequence of the second law of thermodynamics is the development of the physical property of matter, which is known as the entropy (S). The change in this property is used to determine the direction in which a given process will proceed. Entropy quantifies the energy of a substance that is no longer available to perform useful work. This relates to the second law since the second law predicts that not all heat provided to a cycle can be transformed into an equal amount of work. Some heat rejection must take place.

See also: Entropy

According to Clausius, the entropy was defined via the change in entropy S of a system. The change in entropy S, when an amount of heat Q is added to it by a reversible process at a constant temperature, is given by:

Here Q is the energy transferred as heat to or from the system during the process, and T is the temperature of the system in kelvins during the process. The SI unit for entropy is J/K.

The second law of thermodynamics can also be expressed as ∆S≥0 for a closed cycle.

In words:

The entropy of any isolated system never decreases. In a natural thermodynamic process, the sum of the entropies of the interacting thermodynamic systems increases.

∆S≥0

Because entropy tells so much about the usefulness of an amount of heat transferred in performing work, the steam tables include values of specific entropy (s = S/m) as part of the information tabulated.

Heat Engines

Energy sources have always played a very important role in the development of human society. Energy is generally defined as the potential to do work or produce heat. Sometimes it is like the “currency” for performing work. One of the most wonderful properties of the universe is that energy can be transformed from one type to another and transferred from one object to another.

In general, it is easy to produce thermal energy by doing work, for example, by any frictional process. But to get work from thermal energy is more difficult. It is closely associated with the concept of entropy. For example, electricity is particularly useful since it has very low entropy (is highly ordered) and can be converted into other forms of energy very efficiently.

Sometimes, mechanical energy is directly available, for example, wind power and hydropower. But most of our energy comes from burning fossil fuels (coal, oil, and gas) and nuclear reactions. At present, fossil fuel is still the world’s predominant energy source. But the burning of fossil fuels generates only thermal energy. Therefore these energy sources are so-called “primary energy sources”, that must be converted to the secondary energy source, so-called energy carriers (electrical energy, etc.). To convert thermal energy into another form of energy, a heat engine must be used.

In general, a heat engine is a device that converts chemical energy to heat or thermal energy and then to mechanical energy or electrical energy.

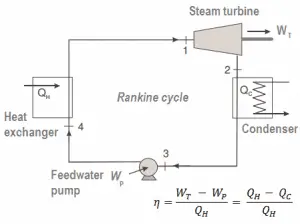
Many heat engines operate in a cyclic manner, adding energy in the form of heat in one part of the cycle and using that energy to do useful work in another part of the cycle.
For example, as is typical in all conventional thermal power plants, the heat is used to generate steam which drives a steam turbine connected to a generator that produces electricity. Steam generators, steam turbines, condensers, and feedwater pumps constitute a heat engine subject to the efficiency limitations imposed by the second law of thermodynamics. In modern nuclear power plants, the overall thermodynamic efficiency is about one-third (33%), so 3000 MWth of thermal power from the fission reaction is needed to generate 1000 MWe of electrical power.

Types of Heat Engines

In general, heat engines are categorized according to a combustion location as:

• External combustion engine. For example, steam engines are external combustion engines, where the working fluid is separate from the combustion products.
• Internal combustion engine. A typical example of an internal combustion engine is an engine used in automobiles. The high temperature is achieved by burning the gasoline-air mixture in the cylinder itself.

The detailed categorization is based on a working fluid used in a thermodynamic cycle:

• Gas Cycles. In these cycles, the working fluid is always a gas. The Otto and Diesel cycles (used in automobiles) are also typical examples of gas-only cycles. Modern gas turbine engines and airbreathing jet engines are also based on the gas-only cycle, and they follow the Brayton cycle.
• Liquid Cycles. Liquid-only cycles are rather exotic. In these cycles, the working fluid is always a liquid. The Malone liquid engine is an example of the liquid-only cycle. The Malone liquid engine modified the Stirling cycle, using water as the working fluid instead of gas.
• Cycles with Phase Changes. Steam engines are typical examples of external engines with phase change of working fluid.

Example of Heat Engine

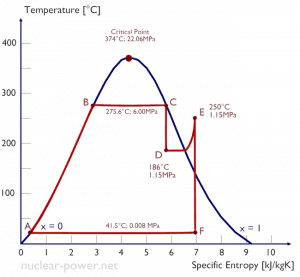
Steam engines and refrigerators are typical examples of external engines with phase change of working fluid. The typical thermodynamic cycle used to analyze this process is the Rankine cycle, which usually uses water as the working fluid.

The Rankine cycle closely describes the processes in steam-operated heat engines commonly found in most thermal power plants. The heat sources used in these power plants are usually the combustion of fossil fuels such as coal, natural gas, or nuclear fission.

A nuclear power plant (nuclear power station) looks like a standard thermal power station with one exception. The heat source in the nuclear power plant is a nuclear reactor. As is typical in all conventional thermal power stations, the heat is used to generate steam which drives a steam turbine connected to a generator that produces electricity.

Typically most nuclear power plants operate multi-stage condensing steam turbines. In these turbines, the high-pressure stage receives steam (this steam is nearly saturated steam – x = 0.995 – point C at the figure; 6 MPa; 275.6°C) from a steam generator and exhausts it to moisture separator-reheater (point D). The steam must be reheated to avoid damages caused to the steam turbine blades by low-quality steam. The reheater heats the steam (point D), and then the steam is directed to the low-pressure stage of the steam turbine, where it expands (point E to F). The exhausted steam then condenses in the condenser. It is at a pressure well below atmospheric (absolute pressure of 0.008 MPa) and is in a partially condensed state (point F), typically of a quality near 90%.

In this case, steam generators, steam turbines, condensers, and feedwater pumps constitute a heat engine subject to the efficiency limitations imposed by the second law of thermodynamics. In an ideal case (no friction, reversible processes, perfect design), this heat engine would have a Carnot efficiency of

= 1 – T_cold/T_hot = 1 – 315/549 = 42.6%

where the temperature of the hot reservoir is 275.6°C (548.7K), the temperature of the cold reservoir is 41.5°C (314.7K). But the nuclear power plant is the real heat engine, in which thermodynamic processes are somehow irreversible. They are not done infinitely slowly. In real devices (turbines, pumps, and compressors), mechanical friction and heat losses cause further efficiency losses.

Therefore nuclear power plants usually have an efficiency of about 33%. In modern nuclear power plants, the overall thermodynamic efficiency is about one-third (33%), so 3000 MWth of thermal power from the fission reaction is needed to generate 1000 MWe of electrical power.

According to Carnot’s principle, higher efficiencies can be attained by increasing the temperature of the steam. But this requires an increase in pressures inside boilers or steam generators. However, metallurgical considerations place upper limits on such pressures.  From this point of view, supercritical water reactors are considered a promising advancement for nuclear power plants because of their high thermal efficiency (~45 % vs. ~33 % for current LWRs). SCWRs are operated at supercritical pressure (i.e., greater than 22.1 MPa).

Thermal Efficiency and the Second Law

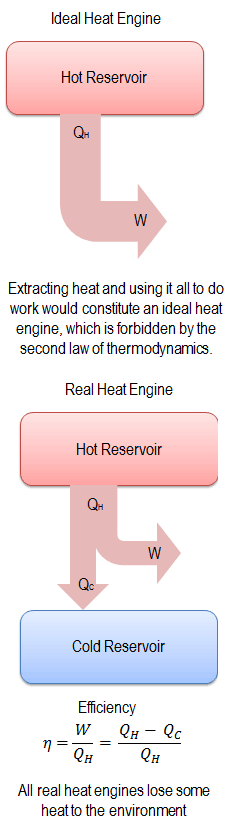
An ideal heat engine is an imaginary engine in which energy extracted as heat from the high-temperature reservoir is converted completely to work. But according to the Kelvin-Planck statement, such an engine would violate the second law of thermodynamics because there must be losses in the conversion process. The net heat added to the system must be higher than the net work done by the system.

Kelvin-Planck statement:

“It is impossible to construct a device which operates on a cycle and produces no other effect than the production of work and the transfer of heat from a single body”.

Thermal Efficiency Formula

As a result of this statement, we define the thermal efficiency, η_th, of any heat engine as the ratio of the work it does, W, to the heat input at the high temperature, Q_H.

The thermal efficiency, η_th, represents the fraction of heat, Q_H, converted to work. It is a dimensionless performance measure of a heat engine that uses thermal energy, such as a steam turbine, an internal combustion engine, or a refrigerator. For refrigeration or heat pumps, thermal efficiency indicates the extent to which the energy added by work is converted to net heat output. Since it is a dimensionless number, we must always express W, Q_H, and Q_C in the same units.

Since energy is conserved according to the first law of thermodynamics and energy cannot be converted to work completely, the heat input, Q_H, must equal the work done, W, plus the heat that must be dissipated as waste heat Q_C into the environment. Therefore we can rewrite the formula for thermal efficiency as:

To give the efficiency as a percent, we multiply the previous formula by 100. Note that η_th could be 100% only if the waste heat Q_C is zero.

In general, the efficiency of even the best heat engines is quite low. In short, it is very difficult to convert thermal energy to mechanical energy. The thermal efficiencies are usually below 50% and often far below. Be careful when you compare it with efficiencies of wind or hydropower (wind turbines are not heat engines). There is no energy conversion between thermal and mechanical energy.

Causes of Inefficiency

As was discussed, an efficiency can range between 0 and 1. Each heat engine is somehow inefficient. This inefficiency can be attributed to three causes.

• Irreversibility of Processes. There is an overall theoretical upper limit to the efficiency of conversion of heat to work in any heat engine. This upper limit is called the Carnot efficiency. According to the Carnot principle, no engine can be more efficient than a reversible engine (a Carnot heat engine) operating between the same high temperature and low-temperature reservoirs. For example, when the hot reservoir has T_hot of 400°C (673K) and T_cold of about 20°C (293K), the maximum (ideal) efficiency will be: = 1 – T_cold/T_hot = 1 – 293/673 = 56%. But all real thermodynamic processes are somehow irreversible. They are not done infinitely slowly. Therefore, heat engines must have lower efficiencies than limits on their efficiency due to the inherent irreversibility of the heat engine cycle they use.
• Presence of Friction and Heat Losses. In real thermodynamic systems or real heat engines, a part of the overall cycle inefficiency is due to the losses by the individual components. In real devices (such as turbines, pumps, and compressors), mechanical friction, heat losses, and losses in the combustion process cause further efficiency losses.
• Design Inefficiency. Finally, the last and important source of inefficiencies is the compromises made by engineers when designing a heat engine (e.g.,, power plant). They must consider cost and other factors in the design and operation of the cycle. As an example, consider the design of the condenser in the thermal power plants.  Ideally, the steam exhausted into the condenser would have no subcooling. But real condensers are designed to subcool the liquid by a few degrees Celsius to avoid the suction cavitation in the condensate pumps. But, this subcooling increases the inefficiency of the cycle because more energy is needed to reheat the water.

Thermal Efficiency of Heat Engines

In general, the efficiency of even the best heat engines is quite low. In short, it is very difficult to convert thermal energy to mechanical energy. The thermal efficiencies are usually below 50% and often far below.

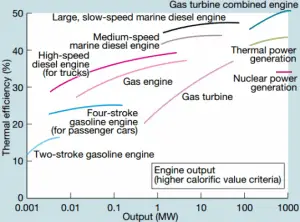
It is easy to produce thermal energy by doing work, for example, by any frictional process. But to get work from thermal energy is more difficult. It is closely associated with the concept of entropy, which quantifies the energy of a substance that is no longer available to perform useful work. For example, electricity is particularly useful since it has very low entropy (is highly ordered) and can be converted into other forms of energy very efficiently. Be careful when you compare it with efficiencies of wind or hydropower (wind turbines are not heat engines). There is no energy conversion between thermal and mechanical energy.

The thermal efficiency of various heat engines designed or used today has a large range:

For example:

Transportation

• In the middle of the twentieth century, a typical steam locomotive had a thermal efficiency of about 6%. That means for every 100 MJ of coal burned, 6 MJ of mechanical power were produced.
• A typical gasoline automotive engine operates at around 25% to 30% of thermal efficiency. About 70-75% is rejected as waste heat without being converted into useful work, i.e., work delivered to wheels.
• A typical diesel automotive engine operates at around 30% to 35%. In general, engines using the Diesel cycle are usually more efficient.
• In 2014, new regulations were introduced for Formula 1 cars. These motorsport regulations have pushed teams to develop highly efficient power units. According to Mercedes, their power unit is now achieving more than 45% and close to 50% thermal efficiency, i.e., 45 – 50% of the potential energy in the fuel is delivered to wheels.
• The diesel engine has the highest thermal efficiency of any practical combustion engine. Low-speed diesel engines (as used in ships) can have a thermal efficiency that exceeds 50%. The largest diesel engine in the world peaks at 51.7%.

Power Engineering

• Ocean thermal energy conversion (OTEC).  OTEC is a sophisticated heat engine that uses the temperature difference between cooler deep and warmer surface seawaters to run a low-pressure turbine. Since the temperature difference is low, about 20°C, its thermal efficiency is also very low, about 3%.
• In modern nuclear power plants, the overall thermal efficiency is about one-third (33%), so 3000 MWth of thermal power from the fission reaction is needed to generate 1000 MWe of electrical power. Higher efficiencies can be attained by increasing the temperature of the steam. But this requires an increase in pressures inside boilers or steam generators. However, metallurgical considerations place upper limits on such pressures. In comparison to other energy sources, the thermal efficiency of 33% is not much. But it must be noted that nuclear power plants are much more complex than fossil fuel power plants, and it is much easier to burn fossil fuel than to generate energy from nuclear fuel.
• Sub-critical fossil fuel power plants operated under critical pressure (i.e., lower than 22.1 MPa) can achieve 36–40% efficiency.
• Supercritical water reactors are considered a promising advancement for nuclear power plants because of their high thermal efficiency (~45 % vs. ~33 % for current LWRs).
• Supercritical fossil fuel power plants operated at supercritical pressure (i.e., greater than 22.1 MPa) have efficiencies of around 43%. Most efficient and complex coal-fired power plants operate at “ultra critical” pressures (i.e., around 30 MPa) and use multiple stage reheat to reach about 48% efficiency.
• Modern Combined Cycle Gas Turbine (CCGT) plants, in which the thermodynamic cycle consists of two power plant cycles (e.g.,, the Brayton cycle and the Rankine cycle), can achieve a thermal efficiency of around 55%, in contrast to a single cycle steam power plant which is limited to efficiencies of around 35-45%.