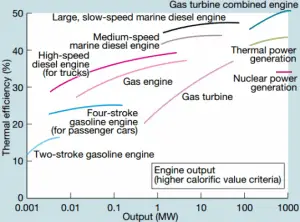
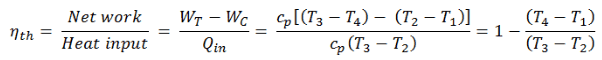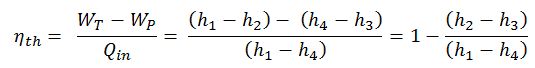In general, the efficiency of even the best heat engines is quite low. In short, it is very difficult to convert thermal energy to mechanical energy. The thermal efficiencies are usually below 50% and often far below.
It is easy to produce thermal energy by doing work, for example, by any frictional process. But to get work from thermal energy is more difficult. It is closely associated with the concept of entropy, which quantifies the energy of a substance that is no longer available to perform useful work. For example, electricity is particularly useful since it has very low entropy (is highly ordered) and can be converted into other forms of energy very efficiently. Be careful when you compare it with efficiencies of wind or hydropower (wind turbines are not heat engines). There is no energy conversion between thermal and mechanical energy.
The thermal efficiency of various heat engines designed or used today has a large range:
For example:
Transportation
- In the middle of the twentieth century, a typical steam locomotive had a thermal efficiency of about 6%. That means for every 100 MJ of coal burned, 6 MJ of mechanical power were produced.
- A typical gasoline automotive engine operates at around 25% to 30% of thermal efficiency. About 70-75% is rejected as waste heat without being converted into useful work, i.e., work delivered to wheels.
- A typical diesel automotive engine operates at around 30% to 35%. In general, engines using the Diesel cycle are usually more efficient.
- In 2014, new regulations were introduced for Formula 1 cars. These motorsport regulations have pushed teams to develop highly efficient power units. According to Mercedes, their power unit is now achieving more than 45% and close to 50% thermal efficiency, i.e., 45 – 50% of the potential energy in the fuel is delivered to wheels.
- The diesel engine has the highest thermal efficiency of any practical combustion engine. Low-speed diesel engines (as used in ships) can have a thermal efficiency that exceeds 50%. The largest diesel engine in the world peaks at 51.7%.
Power Engineering
- Ocean thermal energy conversion (OTEC). OTEC is a sophisticated heat engine that uses the temperature difference between cooler deep and warmer surface seawaters to run a low-pressure turbine. Since the temperature difference is low, about 20°C, its thermal efficiency is also very low, about 3%.
- In modern nuclear power plants, the overall thermal efficiency is about one-third (33%), so 3000 MWth of thermal power from the fission reaction is needed to generate 1000 MWe of electrical power. Higher efficiencies can be attained by increasing the temperature of the steam. But this requires an increase in pressures inside boilers or steam generators. However, metallurgical considerations place upper limits on such pressures. In comparison to other energy sources, the thermal efficiency of 33% is not much. But it must be noted that nuclear power plants are much more complex than fossil fuel power plants, and it is much easier to burn fossil fuel than to generate energy from nuclear fuel.
- Under critical pressure, sub-critical fossil fuel power plants (i.e., lower than 22.1 MPa) can achieve 36–40% efficiency.
- Supercritical water reactors are considered a promising advancement for nuclear power plants because of their high thermal efficiency (~45 % vs. ~33 % for current LWRs).
- Supercritical fossil fuel power plants operated at supercritical pressure (i.e., greater than 22.1 MPa have efficiencies around 43%. Most efficient and complex coal-fired power plants operate at “ultra critical” pressures (i.e., around 30 MPa) and use multiple stage reheat to reach about 48% efficiency.
- Modern Combined Cycle Gas Turbine (CCGT) plants, in which the thermodynamic cycle consists of two power plant cycles (e.g.,, the Brayton cycle and the Rankine cycle), can achieve a thermal efficiency of around 55%, in contrast to a single cycle steam power plant which is limited to efficiencies of around 35-45%.
Thermal Efficiency of Brayton Cycle
Let assume the ideal Brayton cycle that describes the workings of a constant pressure heat engine. Modern gas turbine engines and airbreathing jet engines also follow the Brayton cycle. This cycle consist of four thermodynamic processes:
-
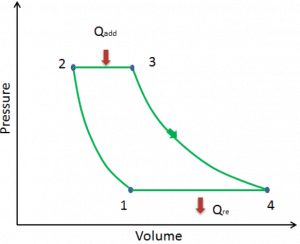
The ideal Brayton cycle consists of four thermodynamic processes. Two isentropic processes and two isobaric processes. Isentropic compression – ambient air is drawn into the compressor, pressurized (1 → 2). The work required for the compressor is given by WC = H2 – H1.
- Isobaric heat addition – the compressed air then runs through a combustion chamber, burning fuel, and air or another medium is heated (2 → 3). It is a constant-pressure process since the chamber is open to flow in and out. The net heat added is given by Qadd = H3 – H2
- Isentropic expansion – the heated, pressurized air then expands on a turbine, gives up its energy. The work done by the turbine is given by WT = H4 – H3
- Isobaric heat rejection – the residual heat must be rejected to close the cycle. The net heat rejected is given by Qre = H4 – H1
As can be seen, we can describe and calculate (e.g.,, thermodynamic efficiency) such cycles (similarly for Rankine cycle) using enthalpies.
To calculate the thermal efficiency of the Brayton cycle (single compressor and single turbine), engineers use the first law of thermodynamics in terms of enthalpy rather than in terms of internal energy.
The first law in terms of enthalpy is:
dH = dQ + Vdp
In this equation, the term Vdp is a flow process work. This work, Vdp, is used for open flow systems like a turbine or a pump in which there is a “dp”, i.e., change in pressure. There are no changes in the control volume. As can be seen, this form of the law simplifies the description of energy transfer.
There are expressions in terms of more familiar variables such as temperature and pressure:
dH = CpdT + V(1-αT)dp
where Cp is the heat capacity at constant pressure and α is the (cubic) thermal expansion coefficient. For ideal gas αT = 1 and therefore:
dH = CpdT
At constant pressure, the enthalpy change equals the energy transferred from the environment through heating:
Isobaric process (Vdp = 0):
dH = dQ → Q = H2 – H1 → H2 – H1 = Cp (T2 – T1)
At constant entropy, i.e., in isentropic process, the enthalpy change equals the flow process work done on or by the system:
Isentropic process (dQ = 0):
dH = Vdp → W = H2 – H1 → H2 – H1 = Cp (T2 – T1)

The enthalpy can be made into an intensive or specific variable by dividing by the mass. Engineers use the specific enthalpy in thermodynamic analysis more than the enthalpy itself. The thermal efficiency of such a simple Brayton cycle for ideal gas and in terms of specific enthalpies can now be expressed in terms of the temperatures:
Thermal Efficiency of Rankine Cycle
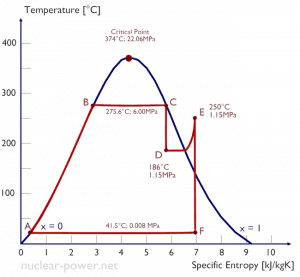
The Rankine cycle closely describes the processes in steam-operated heat engines commonly found in most thermal power plants. The heat sources used in these power plants are usually the combustion of fossil fuels such as coal, natural gas, or nuclear fission.
A nuclear power plant (nuclear power station) looks like a standard thermal power station with one exception. The heat source in the nuclear power plant is a nuclear reactor. As is typical in all conventional thermal power stations, the heat is used to generate steam which drives a steam turbine connected to a generator that produces electricity.
Typically most nuclear power plants operate multi-stage condensing steam turbines. In these turbines, the high-pressure stage receives steam (this steam is nearly saturated steam – x = 0.995 – point C at the figure; 6 MPa; 275.6°C) from a steam generator and exhausts it to moisture separator-reheater (point D). The steam must be reheated to avoid damages caused to the steam turbine blades by low-quality steam. The reheater heats the steam (point D), and then the steam is directed to the low-pressure stage of the steam turbine, where it expands (point E to F). The exhausted steam then condenses in the condenser. It is at a pressure well below atmospheric (absolute pressure of 0.008 MPa) and is in a partially condensed state (point F), typically of a quality near 90%.
In this case, steam generators, steam turbines, condensers, and feedwater pumps constitute a heat engine subject to the efficiency limitations imposed by the second law of thermodynamics. In an ideal case (no friction, reversible processes, perfect design), this heat engine would have a Carnot efficiency of
= 1 – Tcold/Thot = 1 – 315/549 = 42.6%
where the temperature of the hot reservoir is 275.6°C (548.7K), the temperature of the cold reservoir is 41.5°C (314.7K). But the nuclear power plant is the real heat engine, in which thermodynamic processes are somehow irreversible. They are not done infinitely slowly. In real devices (turbines, pumps, and compressors), mechanical friction and heat losses cause further efficiency losses.
To calculate the thermal efficiency of the simplest Rankine cycle (without reheating), engineers use the first law of thermodynamics in terms of enthalpy rather than in terms of internal energy.
The first law in terms of enthalpy is:
dH = dQ + Vdp
In this equation, the term Vdp is a flow process work. This work, Vdp, is used for open flow systems like a turbine or a pump in which there is a “dp”, i.e., change in pressure. There are no changes in the control volume. As can be seen, this form of the law simplifies the description of energy transfer. At constant pressure, the enthalpy change equals the energy transferred from the environment through heating:
Isobaric process (Vdp = 0):
dH = dQ → Q = H2 – H1
At constant entropy, i.e., in isentropic process, the enthalpy change equals the flow process work done on or by the system:
Isentropic process (dQ = 0):
dH = Vdp → W = H2 – H1
It will be very useful in analyzing both thermodynamic cycles used in power engineering, i.e., in the Brayton and Rankine cycles.
The enthalpy can be made into an intensive or specific variable by dividing by the mass. Engineers use the specific enthalpy in thermodynamic analysis more than the enthalpy itself. It is tabulated in the steam tables along with specific volume and specific internal energy. The thermal efficiency of such a simple Rankine cycle and in terms of specific enthalpies would be:
It is a very simple equation, and for the determination of the thermal efficiency, you can use data from steam tables.
In modern nuclear power plants, the overall thermal efficiency is about one-third (33%), so 3000 MWth of thermal power from the fission reaction is needed to generate 1000 MWe of electrical power. The reason lies in relatively low steam temperature (6 MPa; 275.6°C). Higher efficiencies can be attained by increasing the temperature of the steam. But this requires an increase in pressures inside boilers or steam generators. However, metallurgical considerations place upper limits on such pressures. In comparison to other energy sources, the thermal efficiency of 33% is not much. But it must be noted that nuclear power plants are much more complex than fossil fuel power plants, and it is much easier to burn fossil fuel than to generate energy from nuclear fuel. Sub-critical fossil fuel power plants that are operated under critical pressure (i.e., lower than 22.1 MPa) can achieve 36–40% efficiency.