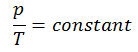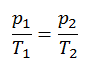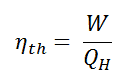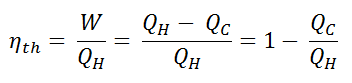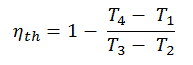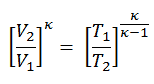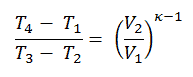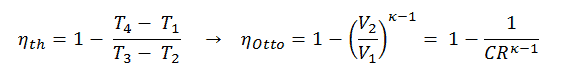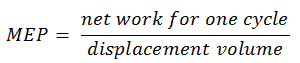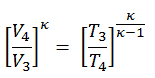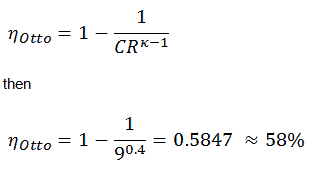Theory of Otto Cycle – Gasoline Engine

Otto Cycle – Otto Engine

In 1876, a German engineer, Nikolaus August Otto, advanced the study of heat engines by building the first working four-stroke engine. A stationary engine using a coal gas-air mixture for fuel. Wilhelm Maybach (1846-1929), one of the most important German engineers, perfected the construction, which was produced in large quantities already at the end of the year 1876. These inventions quickly reshaped the world in which they lived.

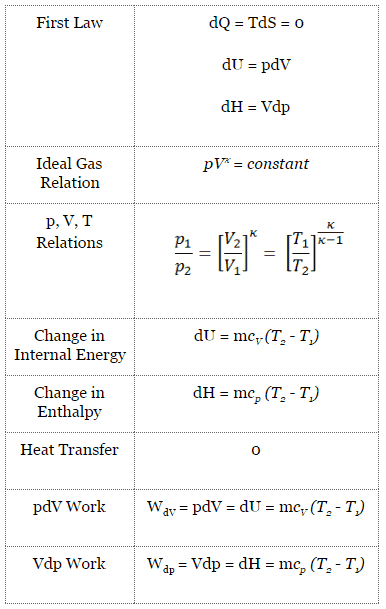
The cycle of the Otto engine is called the Otto cycle. It is one of the most common thermodynamic cycles found in automobile engines and describes the functioning of a typical spark ignition piston engine. In contrast to the Carnot cycle, the Otto cycle does not execute isothermal processes because these must be performed very slowly. In an ideal Otto cycle, the system executing the cycle undergoes a series of four internally reversible processes: two isentropic (reversible adiabatic) processes alternated with two isochoric processes.

Since Carnot’s principle states that no engine can be more efficient than a reversible engine (a Carnot heat engine) operating between the same high temperature and low-temperature reservoirs, the Otto engine must have lower efficiency than the Carnot efficiency. A typical gasoline automotive engine operates at around 25% to 30% of thermal efficiency. About 70-75% is rejected as waste heat without being converted into useful work, i.e., work delivered to wheels.

Otto Cycle – Processes

In an ideal Otto cycle, the system executing the cycle undergoes a series of four internally reversible processes: two isentropic (reversible adiabatic) processes alternated with two isochoric processes:

1. Isentropic compression (compression stroke) – The gas (fuel-air mixture) is compressed adiabatically from state 1 to state 2, as the piston moves from bottom dead center to top dead center. The surroundings do work on the gas, increasing its internal energy (temperature) and compressing it. On the other hand, the entropy remains unchanged. The volume changes, and the ratio (V₁ / V₂) is known as the compression ratio.
2. Isochoric compression (ignition phase) – In this phase (between state 2 and state 3), there is a constant volume (the piston is at rest ) heat transfer to the air from an external source while the piston is at rest at the top dead center. This process is intended to represent the ignition of the fuel-air mixture injected into the chamber and the subsequent rapid burning. The pressure rises, and the ratio (P₃ / P₂) is known as the “explosion ratio”.
3. Isentropic expansion (power stroke) – The gas expands adiabatically from state 3 to state 4 as the piston moves from top dead center to bottom dead center. The gas works on the surroundings (piston) and loses an amount of internal energy equal to the work that leaves the system. Again the entropy remains unchanged. The volume ratio (V₄ / V₃) is known as the isentropic expansion ratio, but it is equal to the compression ratio for the Otto cycle.
4. Isochoric decompression (exhaust stroke) – In this phase, the cycle completes by a constant-volume process in which heat is rejected from the air while the piston is at the bottom dead center. The working gas pressure drops instantaneously from point 4 to point 1. The exhaust valve opens at point 4. The exhaust stroke is directly after this decompression. As the piston moves from the bottom dead center (point 1) to the top dead center (point 0) with the exhaust valve opened, the gaseous mixture is vented to the atmosphere, and the process starts anew.

During the Otto cycle, work is done on the gas by the piston between states 1 and 2 (isentropic compression). Work is done by the gas on the piston between stages 3 and 4 (isentropic expansion). The difference between the work done by the gas and the work done on the gas is the network produced by the cycle, and it corresponds to the area enclosed by the cycle curve. The work produced by the cycle times the rate of the cycle (cycles per second) is equal to the power produced by the Otto engine.

Isentropic Process

An isentropic process is a thermodynamic process in which the entropy of the fluid or gas remains constant. It means the isentropic process is a special case of an adiabatic process in which there is no transfer of heat or matter. It is a reversible adiabatic process. The assumption of no heat transfer is very important since we can use the adiabatic approximation only in very rapid processes.

Isentropic Process and the First Law

For a closed system, we can write the first law of thermodynamics in terms of enthalpy:

dH = dQ + Vdp

or

dH = TdS + Vdp

Isentropic process (dQ = 0):

dH = Vdp     →     W = H₂ – H₁     →     H₂ – H₁ = C_p (T₂ – T₁)    (for ideal gas)

Isentropic Process of the Ideal Gas

The isentropic process (a special case of the adiabatic process) can be expressed with the ideal gas law as:

pV^κ = constant

or

p₁V₁^κ = p₂V₂^κ

in which κ = c_p/c_v is the ratio of the specific heats (or heat capacities) for the gas. One for constant pressure (c_p) and one for constant volume (c_v). Note that, this ratio κ  = c_p/c_v is a factor in determining the speed of sound in a gas and other adiabatic processes.

Isochoric Process

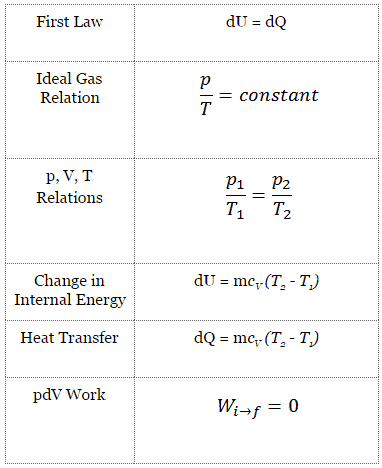
An isochoric process is a thermodynamic process in which the volume of the closed system remains constant (V = const). It describes the behavior of gas inside the container that cannot be deformed. Since the volume remains constant, the heat transfer into or out of the system does not the p∆V work but only changes the system’s internal energy (the temperature).

Isochoric Process and the First Law

The classical form of the first law of thermodynamics is the following equation:

dU = dQ – dW

In this equation, dW is equal to dW = pdV and is known as the boundary work. Then:

dU = dQ  – pdV

An isochoric process and the ideal gas, all of the heat added to the system, will increase the internal energy.

Isochoric process (pdV = 0):

dU = dQ    (for ideal gas)

dU = 0 = Q – W    →     W = Q      (for ideal gas)

Isochoric Process of the Ideal Gas

The isochoric process can be expressed with the ideal gas law as:

or

On a p-V diagram, the process occurs along a horizontal line with the equation V = constant.

See also: Guy-Lussac’s Law.

Comparison of Actual and Ideal Otto Cycles

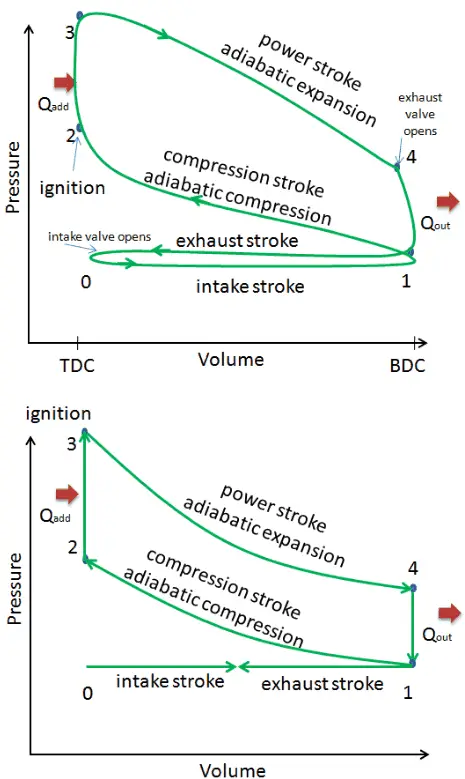This section shows an ideal Otto cycle in which there are a lot of assumptions that differ from the actual Otto cycle. The main differences between the actual and ideal Otto engine appear in the figure. In reality, the ideal cycle does not occur, and there are many losses associated with each process. For an actual cycle, the shape of the pV diagram is similar to the ideal, but the area (work) enclosed by the pV diagram is always less than the ideal value. The ideal Otto cycle is based on the following assumptions:

• Closed cycle. The largest difference between the two diagrams is the simplification of the intake and exhaust strokes in the ideal cycle. In the exhaust stroke, heat Q_out is ejected into the environment. In a real engine, the gas leaves the engine and is replaced by a new mixture of air and fuel.
• Instantaneous heat addition (isochoric heat addition). In real engines, the heat addition is not instantaneous. Therefore the peak pressure is not at TDC but just after TDC.
• No heat transfer (adiabatic)
  • Compression – The gas (fuel-air mixture) is compressed adiabatically from state 1 to state 2. In real engines, there are always some inefficiencies that reduce the thermal efficiency.
  • Expansion. The gas (fuel-air mixture) expands adiabatically from state 3 to state 4.
• Complete combustion of the fuel-air mixture.
• No pumping work. Pumping work is the difference between the work done during the exhaust stroke and the intake stroke. In real cycles, there is a pressure difference between exhaust and inlet pressures.
• No blowdown loss. Blowdown loss is caused by the early opening of exhaust valves. This results in a loss of work output during the expansion stroke.
• No blow-by loss. The blow-by loss is caused by the leakage of compressed gases through piston rings and other crevices.
• No frictional losses.

These simplifying assumptions and losses lead to the fact that the enclosed area (work) of the pV diagram for an actual engine is significantly smaller than the area (work) enclosed by the pV diagram of the ideal cycle. In other words, the ideal engine cycle will overestimate the network and, if the engines run at the same speed, greater power is produced by the actual engine by around 20%.

Compression Ratio – Otto Engine

The compression ratio, CR, is defined as the ratio of the volume at the bottom dead center and the volume at the top dead center.  It is a key characteristic of many internal combustion engines. In the following section, it will be shown that the compression ratio determines the thermal efficiency of the used thermodynamic cycle of the combustion engine. It is desired to have a high compression ratio because it allows an engine to reach higher thermal efficiency.

For example, let assume an Otto cycle with compression ratio of CR = 10 : 1. The volume of the chamber is 500 cm³ = 500×10^-6 m³ (0.5l) prior to the compression stroke. For this engine all required volumes are known:

• V₁ = V₄ = V_max = 500×10^-6 m³ (0.5l)
• V₂ = V₃ = V_min = V_max / CR = 55.56 ×10^-6 m³

Note that (V_max – V_min) x number of cylinders = total engine displacement.

Thermal Efficiency for Otto Cycle

In general, the thermal efficiency, η_th, of any heat engine is defined as the ratio of the work it does, W, to the heat input at the high temperature, Q_H.

The thermal efficiency, η_th, represents the fraction of heat, Q_H, converted to work. Since energy is conserved according to the first law of thermodynamics and energy cannot be converted to work completely, the heat input, Q_H, must equal the work done, W, plus the heat that must be dissipated as waste heat Q_C into the environment. Therefore we can rewrite the formula for thermal efficiency as:

The heat absorbed occurs during combustion of fuel-air mixture, when the spark occurs, roughly at constant volume. Since during an isochoric process there is no work done by or on the system, the first law of thermodynamics dictates ∆U = ∆Q. Therefore, the heat added and rejected is given by:

Q_add = mc_v (T₃ – T₂)

Q_out = mc_v (T₄ – T₁)

Substituting these expressions for the heat added and rejected in the expression for thermal efficiency yields:

We can simplify the above expression using the fact that the processes 1 → 2 and from 3 → 4 are adiabatic, and for an adiabatic process, the following p,V,T formula is valid:

It can be derived that:

In this equation, the ratio V₁/V₂ is known as the compression ratio, CR. When we rewrite the expression for thermal efficiency using the compression ratio, we conclude the air-standard Otto cycle thermal efficiency is a function of compression ratio and κ = c_p/c_v.

It is a very useful conclusion because it is desirable to achieve a high compression ratio to extract more mechanical energy from a given mass of the air-fuel mixture. A higher compression ratio permits the same combustion temperature to be reached with less fuel while giving a longer expansion cycle. This creates more mechanical power output and lowers the exhaust temperature. Lowering the exhaust temperature causes the lowering of the energy rejected to the atmosphere. This relationship is shown in the figure for κ = 1.4, representing ambient air.

 

Mean Effective Pressure – MEP

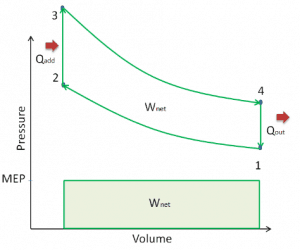
A parameter used by engineers to describe the performance of reciprocating piston engines is known as the mean effective pressure, or MEP. MEP is a useful measure of an engine’s capacity to do work independent of engine displacement. There are several types of MEP. These MEPs are defined by the location measurement and calculation method (e.g.,, BMEP or IMEP).

In general, the mean effective pressure is the constant theoretical pressure that would produce the same network as developed in one complete cycle if it acted on the piston during the power stroke. The MEP can be defined as:

For example, the net indicated mean effective pressure, known as IMEP_n, equals the mean effective pressure calculated from in-cylinder pressure (there must be this measurement) over the complete engine cycle. Note that it is 720° for a four-stroke engine and 360° for a two-stroke engine.

Some examples:

• MEP of an atmospheric gasoline engine can range from 8 to 11 bar in the region of maximum torque.
• MEP of a turbocharged gasoline engine can range from 12 to 17 bar.
• MEP of an atmospheric diesel engine can range from 7 to 9 bar.
• MEP of a turbocharged diesel engine can range from 14 to 18 bar

For example, a four-stroke gasoline engine producing 200 N·m from 2 litres of displacement has a MEP of (4π)(200 N·m)/(0.002 m³) = 1256000 Pa = 12 bar. As can be seen, the MEP is useful characteristics of an engine. For two engines of equal displacement volume, the one with a higher MEP would produce the greater net work and, if the engines run at the same speed, greater power.

Otto Cycle – Problem with Solution

Let assume the Otto cycle, which is one of the most common thermodynamic cycles that can be found in automobile engines. One of the key parameters of such engines is the change in volumes between the top dead center (TDC) to bottom dead center (BDC). The ratio of these volumes (V₁ / V₂) is known as the compression ratio.

The compression ratio in a gasoline-powered engine will usually not be much higher than 10:1 due to potential engine knocking (autoignition) and not lower than 6:1. For example, some sportscar engines can have a compression ratio up to 12.5 : 1 (e.g.,, Ferrari 458 Italia).

In this example, let assume an Otto cycle with a compression ratio of CR = 9 : 1. The intake air is at 100 kPa = 1 bar, 20 °C, and the chamber volume is 500 cm³ before the compression stroke. The temperature at the end of an adiabatic expansion is T₄ = 800 K.

• Specific heat capacity at a constant air pressure at atmospheric pressure and room temperature: c_p = 1.01 kJ/kgK.
• Specific heat capacity at constant air volume at atmospheric pressure and room temperature: c_v = 0.718 kJ/kgK.
• κ = c_p/c_v = 1.4

Calculate:

1. the mass of intake air
2. the temperature T₃
3. the pressure p₃
4. the amount of heat added by burning of fuel-air mixture
5. the thermal efficiency of this cycle
6. the MEP

Solution:

1) the mass of intake air

At the beginning of calculations, we must determine the amount of gas in the cylinder before the compression stroke. Using the ideal gas law, we can find the mass:

pV = mR_specificT

where:

• p is the absolute pressure of the gas
• m is the mass of the substance
• T is the absolute temperature
• V is the volume
• R_specific  is the specific gas constant, equal to the universal gas constant divided by the gas or mixture’s molar mass (M). For dry air R_specific = 287.1 J.kg^-1.K^-1.

therefore

m = p₁V₁/R_specificT₁ = (100000 × 500×10^-6 )/(287.1 × 293) = 5.95×10^-4 kg

In this problem all volumes are known:

• V₁ = V₄ = V_max = 500×10^-6 m³ (0.5l)
• V₂ = V₃ = V_min = V_max / CR = 55.56 ×10^-6 m³

Note that (V_max – V_min) x number of cylinders = total engine displacement.

2) the temperature T₃

Since the process is adiabatic, we can use the following p, V, T relation for adiabatic processes:

thus

T₃ = T₄ . CR^{κ – 1} = 800 . 9^0.4 = 1926 K

3) the pressure p₃

Again, we can use the ideal gas law to find the pressure at the beginning of the power stroke as:

p₃ = mR_specificT₃ / V₃ = 5.95×10^-4 x 287.1 x 1926 / 55.56 ×10^-6 = 5920000 Pa = 59.2 bar

4) the amount of heat added

To calculate the amount of heat added by burning of fuel-air mixture, Q_add, we have to use the first law of thermodynamics for isochoric process, which states the Q_add = ∆U, therefore:

Q_add = mc_v (T₃ – T₂)

The temperature at the end of the compression stroke can be determined using the p, V, T relation for adiabatic processes between points 1 → 2.

T₂ = T₁ . CR^{κ – 1} = 293 . 9^0.4 = 706 K

then

Q_add = mc_v (T₃ – T₂) = 5.95×10^-4 x 718 x 1220 = 521.2 J

5) the thermal efficiency

Thermal efficiency for an Otto cycle:

As was derived in the previous section, the thermal efficiency of an Otto cycle is a function of compression ratio and κ:

6) the mean effective pressure

The MEP was defined as:

In this equation, the displacement volume is equal to V_max – V_min. The network for one cycle can be calculated using the heat added and the thermal efficiency:

W_net = Q_add . η_Otto = 521.2 x 0.5847 = 304.7 J
MEP = 304.7 / (500×10^-6 – 55.56 ×10^-6) = 685.6 kPa = 6.856 bar

 

See above:

Otto Cycle