During the irreversible process, the entropy of the system increases. Many factors make a process irreversible:
- Presence of friction and heat losses. In real thermodynamic systems or real heat processes, we cannot exclude the presence of mechanical friction or heat losses.
- Finite temperature difference. Processes are not done infinitely slowly. For example, there could be turbulence in the gas. Therefore, heat engines must have lower efficiencies than limits on their efficiency due to the inherent irreversibility of the heat engine cycle they use.
- Mixing of two different substances
These factors are present in real, irreversible processes and prevent these processes from being reversible.
Irreversibility of Natural Processes
According to the second law of thermodynamics:
The entropy of any isolated system never decreases. In a natural thermodynamic process, the sum of the entropies of the interacting thermodynamic systems increases.
This law indicates the irreversibility of natural processes. Reversible processes are a useful and convenient theoretical fiction but do not occur in nature. From this law, it is impossible to construct a device that operates on a cycle and whose sole effect is the transfer of heat from a cooler body to a hotter body. It follows perpetual motion machines of the second kind are impossible.
Isentropic vs. Adiabatic Process
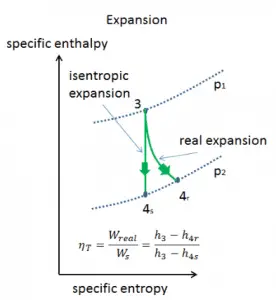
An isentropic process is a thermodynamic process in which the entropy of the fluid or gas remains constant. It means the isentropic process is a special case of an adiabatic process in which there is no transfer of heat or matter. It is a reversible adiabatic process. An isentropic process can also be called a constant entropy process. In engineering, such an idealized process is very useful for comparison with real processes.
One way to make real processes approximate reversible processes is to carry out the process in a series of small or infinitesimal steps or infinitely slowly so that the process can be considered a series of equilibrium states. For example, heat transfer may be considered reversible due to a small temperature difference between the system and its surroundings. But real processes are not done infinitely slowly. Reversible processes are a useful and convenient theoretical fiction but do not occur in nature. For example, there could be turbulence in the gas. Therefore, heat engines must have lower efficiencies than limits on their efficiency due to the inherent irreversibility of the heat engine cycle they use.