Throttling Process – Isenthalpic Process

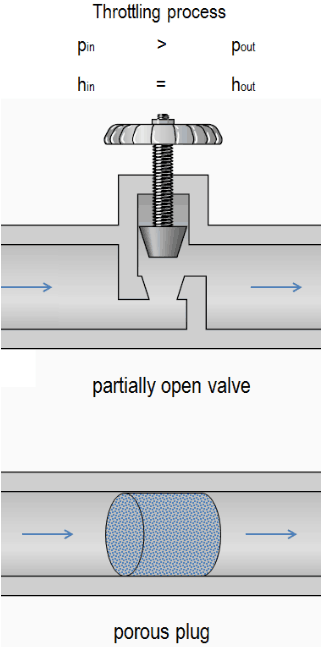
On the other, the throttling process cannot be isentropic. It is a fundamentally irreversible process. Characteristics of throttling process:

1. No Work Transfer
2. No Heat Transfer
3. Irreversible Process
4. Isenthalpic Process

Throttling of the flow causes a significant reduction in pressure because a throttling device causes a local pressure loss. Throttling can be achieved simply by introducing a restriction into a line through which a gas or liquid flows. This restriction is commonly done using a partially open valve or a porous plug. Such pressure losses are generally termed minor losses, although they often account for a major portion of the heat loss. The minor losses are roughly proportional to the square of the flow rate, and therefore they can be easily integrated into the Darcy-Weisbach equation through resistance coefficient K.

For example, consider a throttling of an ideal gas flowing through a partially open valve. From experience, we can observe that: p_in > p_out, v_in < v_out, where p is the pressure and v is the specific volume. We can also observe that specific enthalpies remain the same, i.e., h_in = h_out.

The specific enthalpy is equal to the specific internal energy of the system plus the product of pressure and specific volume.

h = u + pv

Therefore, if the pressure decreases, the specific volume must increase if enthalpy remains constant (assuming u is constant). Because mass flow is constant, the change in specific volume is observed as an increase in gas velocity, and this is also verified by observations.

If there is a change in the internal energy, u, there must be a temperature change. Normally the temperature of the fluid will drop. However, in special cases, the temperature may remain the same, or it may increase.

Joule–Thomson effect – Joule Thomson coefficient

The changes in the temperature during the throttling process are subject to the  Joule-Thomson effect. At room temperature and normal pressures, all gases except hydrogen and helium cool during gas expansion. The cooling occurs because work must be done to overcome the long-range attraction between the gas molecules as they move farther apart. The effect depends on the value of the Joule-Thomson coefficient, which is defined as:

An application of the throttling process occurs in vapor-compression refrigerators, where a throttling valve is used to reduce the pressure and reduce the temperature of the refrigerant from the pressure at the exit of the condenser to the lower pressure existing in the evaporator.

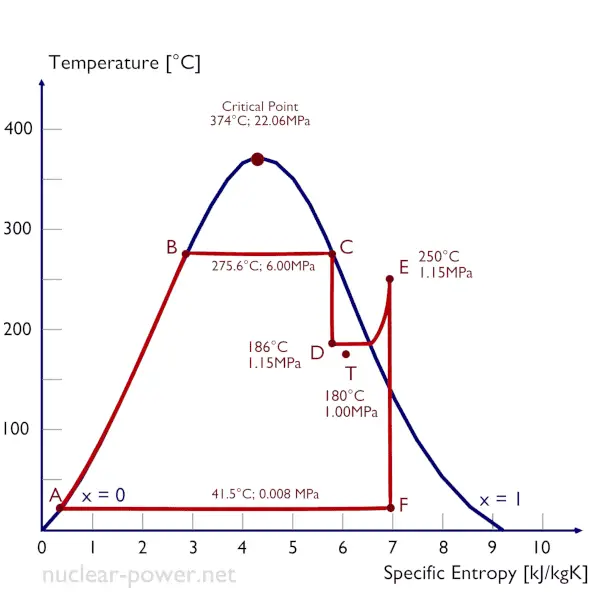
Throttling of Wet Steam

Wet steam is characterized by the vapor quality, ranging from zero to unity – open interval (0,1).  Throttling of the wet steam is also associated with the conservation of enthalpy. Enthalpy is conserved because no work is done by or on the system (dW = 0), and usually, there is no heat transfer (adiabatic) from or into the system (dQ = 0). But in this case, a reduction in pressure causes an increase in vapor quality. As the pressure drops, some of the liquid in the wet steam vaporizes and increases the vapor quality (i.e., dryness fraction). This process takes place because the saturation temperature is lower at the lower pressure. The lower temperature, lower pressure, higher quality steam contains the same enthalpy as the original steam.

Example: Throttling of Wet Steam

A high-pressure steam turbine stage operates at a steady state with inlet conditions of  6 MPa, t = 275.6°C, x = 1 (point C). Steam leaves this turbine stage at a pressure of 1.15 MPa, 186°C, and x = 0.87 (point D). Determine the vapor quality of the steam when throttled from 1.15 MPa to 1.0 MPa. Assume the process is adiabatic and no work is done by the system.

See also: Steam Tables

Solution:

The enthalpy for the state D must be calculated using vapor quality:

h_{D, wet} = h_D,vapor x + (1 – x ) h_D,liquid  = 2782 . 0.87 + (1 – 0.87) . 790 = 2420 + 103 = 2523 kJ/kg

Since it is an isenthalpic process, we know the enthalpy for point T. From steam tables we have to find the vapor quality using the same equation and solving the equation for vapor quality, x:

h_{T, wet} = h_T,vapor x + (1 – x ) h_T,liquid

x = (h_{T, wet} – h_{T, liquid}) / (h_{T, vapor} – h_{T, liquid}) = (2523 – 762) / (2777 – 762) = 0.874 = 87.4%

In this case of the throttling process (1.15MPa to 1MPa), the vapor quality increases from 87% to 87.4%, and the temperature decreases from 186°C to 179.9°C.