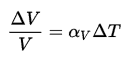Density of Water – H2O
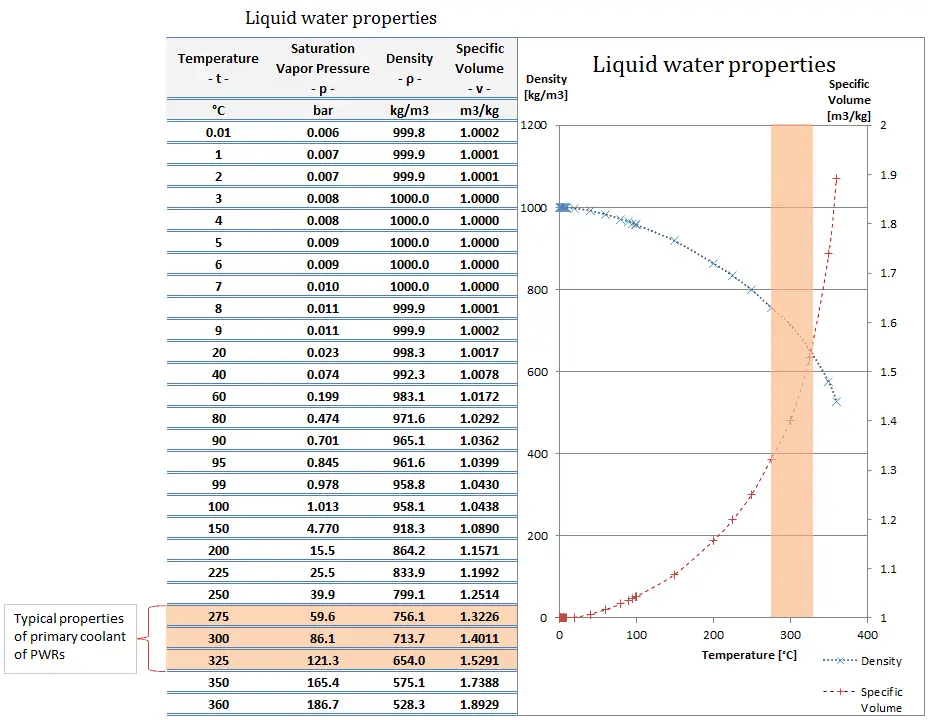
Pure water has its highest density of 1000 kg/m3 at a temperature of 3.98oC (39.2oF). Water differs from most liquids in that it becomes less dense as it freezes. It has a maximum density of 3.98 °C (1000 kg/m3), whereas the density of ice is 917 kg/m3. It differs by about 9%, and therefore ice floats on liquid water. It must be noted, the change in density is not linear with temperature because the volumetric thermal expansion coefficient for water is not constant over the temperature range. The density of water (1 gram per cubic centimeter) was originally used to define the gram. The density (⍴) of a substance is the reciprocal of its specific volume (ν).
ρ = m/V = 1/ν
The specific volume (ν) of a substance is the total volume (V) of that substance divided by the total mass (m) of that substance (volume per unit mass). It has units of cubic meters per kilogram (m3/kg).
The effect of temperature on the densities of liquids and solids is also very important. Most substances expand when heated and contract when cooled. However, the amount of expansion or contraction varies, depending on the material. This phenomenon is known as thermal expansion. The change in volume of a material that undergoes a temperature change is given by the following relation:
where ∆T is the temperature change, V is the original volume, ∆V is the volume change, and αV is the coefficient of volume expansion.
It must be noted and there are exceptions to this rule. For example, water differs from most liquids in that it becomes less dense as it freezes. It has a maximum density of 3.98 °C (1000 kg/m3), whereas the density of ice is 917 kg/m3. It differs by about 9% and therefore ice floats on liquid water
It is an illustrative example, and the following data do not correspond to any reactor design.
Pressurized water reactors are cooled and moderated by high-pressure liquid water (e.g.,, 16MPa). At this pressure, water boils at approximately 350°C (662°F). The inlet temperature of the water is about 290°C (⍴ ~ 720 kg/m3). The water (coolant) is heated in the reactor core to approximately 325°C (⍴ ~ 654 kg/m3) as the water flows through the core.
The primary circuit of typical PWRs is divided into 4 independent loops (piping diameter ~ 700mm). Each loop comprises a steam generator and one main coolant pump. Inside the reactor pressure vessel (RPV), the coolant first flows down outside the reactor core (through the downcomer). The flow is reversed up through the core from the bottom of the pressure vessel, where the coolant temperature increases as it passes through the fuel rods and the assemblies formed by them.
Calculate:
- Pressure loss due to the coolant acceleration in an isolated fuel channel
when
- channel inlet flow velocity is equal to 5.17 m/s
- channel outlet flow velocity is equal to 5.69 m/s
Solution:
The pressure loss due to the coolant acceleration in an isolated fuel channel is then:
This fact has important consequences. Due to the different relative power of fuel assemblies in a core, these fuel assemblies have different hydraulic resistance and this may induce local lateral flow of primary coolant and it must be considered in thermal-hydraulic calculations.
Density of Steam
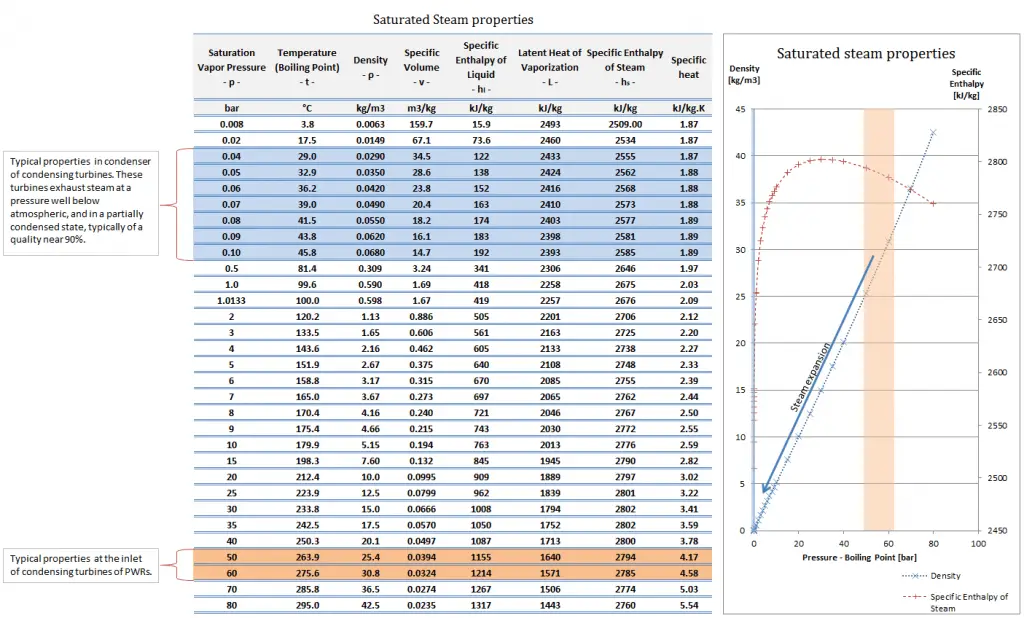
Water and steam are common medium because their properties are very well known. Their properties are tabulated in so-called “Steam Tables”. In these tables, the basic and key properties, such as pressure, temperature, enthalpy, density, and specific heat, are tabulated along the vapor-liquid saturation curve as a function of both temperature and pressure.
The density (⍴) of any substance is the reciprocal of its specific volume (ν).
ρ = m/V = 1/ν
The specific volume (ν) of a substance is the total volume (V) of that substance divided by the total mass (m) of that substance (volume per unit mass). It has units of cubic meters per kilogram (m3/kg).
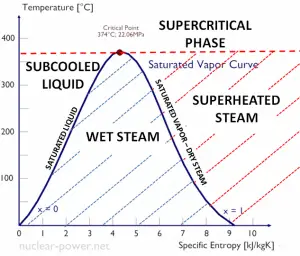
See also: Supercritical Fluid.
The density of Heavy Water
Pure heavy water (D2O) has a density about 11% greater than water but is otherwise physically and chemically similar.
The fact causes this difference, and the deuterium nucleus is twice as heavy as the hydrogen nucleus. Since about 89% of the molecular weight of water comes from the single oxygen atom rather than the two hydrogen atoms, the weight of a heavy water molecule is not substantially different from that of a normal water molecule. The molar mass of water is M(H2O) = 18.02, and the molar mass of heavy water is M(D2O) = 20.03 (each deuterium nucleus contains one neutron in contrast to the hydrogen nucleus). Therefore heavy water (D2O) has a density about 11% greater (20.03/18.03 = 1.112).
Pure heavy water (D2O) has its highest density of 1106 kg/m3 at a temperature of 11.6oC (52.9oF). Also, heavy water differs from most liquids in that it becomes less dense as it freezes. It has a maximum density of 11.6oC (1106 kg/m3), whereas its solid form ice density is 1017 kg/m3. It must be noted, the change in density is not linear with temperature because the volumetric thermal expansion coefficient for water is not constant over the temperature range.