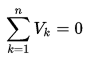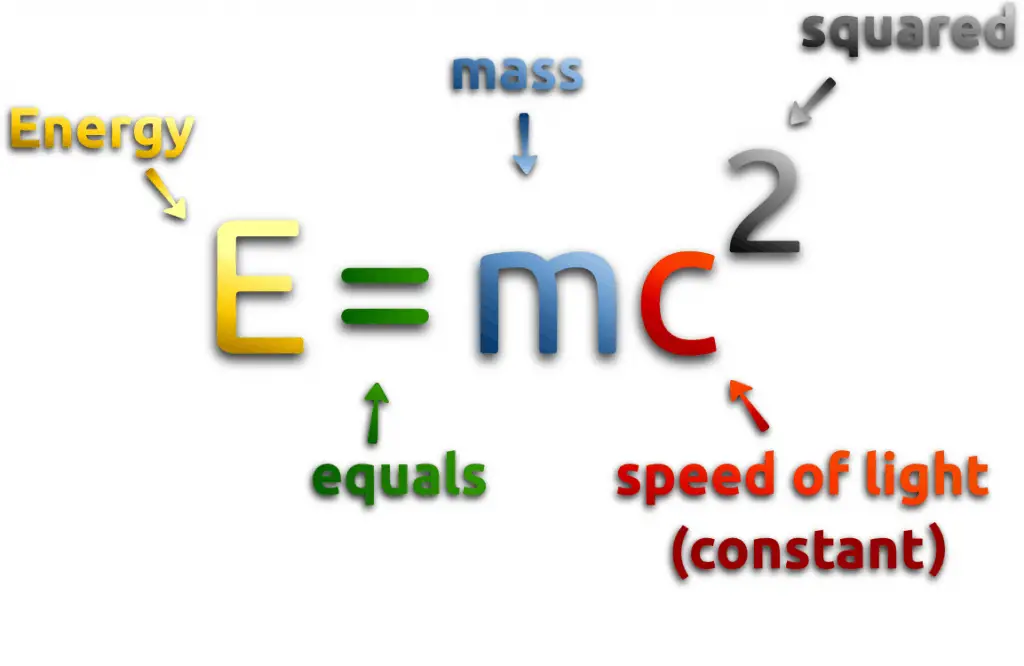The term energy is very broad, and it has many definitions. Technically, energy is a scalar physical quantity that is associated with the state of one or more objects. Energy is generally defined as the potential to do work or produce heat. Sometimes it is like the “currency” for performing work. You must have the energy to accomplish work. To do 1 kilojoule of work, you must expend 1 kilojoule of energy. It must be added, and this interpretation can be misleading because energy is not necessarily available to do work.
Source: hyperphysics.phy-astr.gsu.edu
One of the most wonderful properties of the universe is that energy can be transformed from one type to another and transferred from one object to another. Moreover, when transformed from one type to another and transferred from one object to another, the total amount of energy is always the same. It is one of the elementary properties of the universe.
For example, burning gasoline to power cars is an energy conversion process we rely on. The chemical energy in gasoline is converted to thermal energy, then converted to mechanical energy that makes the car move. The mechanical energy has been converted to kinetic energy. When we use the brakes to stop a car, that kinetic energy is converted by friction back to heat or thermal energy.
Forms of Energy
In thermodynamics, the concept of energy is broadened to account for other observed changes. Thermodynamics deals with another type of energy called “thermal energy” or “internal energy”. The only ways the energy of a closed system can be changed are through a transfer of energy by work or by heat. Further, based on the experiments of Joule and others, a fundamental aspect of the energy concept is that energy is conserved. This principle is known as the first law of thermodynamics. In general, energy is a fundamental concept of thermodynamics and one of the most significant aspects of engineering analysis.