The internal energy of a fixed mass of an ideal gas depends only on its temperature (not pressure or volume).
Potential energy and kinetic energy that was discussed in previous chapters are macroscopic forms of energy. They are dependent on macroscopic variables such as the position and the velocity of objects.
In thermodynamics, internal energy (also called thermal energy) is defined as the energy associated with microscopic forms of energy. It is an extensive quantity, and it depends on the size of the system or on the amount of substance it contains. The SI unit of internal energy is the joule (J). It is the energy contained within the system, excluding the kinetic energy of motion of the system as a whole and the system’s potential energy. Microscopic forms of energy include those due to the rotation, vibration, translation, and interactions among the molecules of a substance. None of these forms of energy can be measured or evaluated directly. Still, techniques have been developed to evaluate the change in the total sum of all these microscopic forms of energy.
In addition, energy can be stored in the chemical bonds between the atoms that make up the molecules. This energy storage on the atomic level includes energy associated with electron orbital states, nuclear spin, and binding forces in the nucleus.
Internal energy is represented by the symbol U, and the change in internal energy in a process is U2 – U1.
Microscopic Energy
Internal energy involves energy on a microscopic scale. It may be divided into microscopic potential energy, Upot, and microscopic kinetic energy, Ukin, components:
U = Upot + Ukin
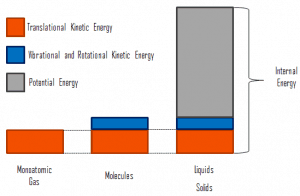 where the microscopic kinetic energy, Ukin, involves the motions of all the system’s particles for the center-of-mass frame. For an ideal monatomic gas, this is just the translational kinetic energy of the linear motion of the atoms. Monoatomic particles do not rotate or vibrate. The kinetic theory of gases well describes the behavior of the system. Kinetic theory is based on the fact that during an elastic collision between a molecule with high kinetic energy and one with low kinetic energy, part of the energy will transfer to the molecule of lower kinetic energy. However, for polyatomic gases, there is rotational and vibrational kinetic energy as well.
where the microscopic kinetic energy, Ukin, involves the motions of all the system’s particles for the center-of-mass frame. For an ideal monatomic gas, this is just the translational kinetic energy of the linear motion of the atoms. Monoatomic particles do not rotate or vibrate. The kinetic theory of gases well describes the behavior of the system. Kinetic theory is based on the fact that during an elastic collision between a molecule with high kinetic energy and one with low kinetic energy, part of the energy will transfer to the molecule of lower kinetic energy. However, for polyatomic gases, there is rotational and vibrational kinetic energy as well.
The microscopic potential energy, Upot, involves the chemical bonds between the atoms that make up the molecules, binding forces in the nucleus, and the physical force fields within the system (e.g.,, electric or magnetic fields).
There is a significant component of potential energy associated with the intermolecular attractive forces in liquids and solids.
Specific Internal Energy
The specific internal energy (u) of a substance is its internal energy per unit mass. It is an intensive property. It equals the total internal energy (U) divided by the total mass (m).
u = U/m
where:
u = specific internal energy (J/kg)
U = internal energy (J)
m = mass (kg)
Internal Energy of an Ideal Gas
Internal energy is the total of all the energy associated with the motion of the atoms or molecules in the system. Microscopic forms of energy include those due to the rotation, vibration, translation, and interactions among the molecules of a substance.
Monatomic Gas
For a monatomic ideal gas (such as helium, neon, or argon), the only contribution to the energy comes from translational kinetic energy. The average translational kinetic energy of a single atom depends only on the gas temperature and is given by the equation:
Kavg = 3/2 kT.
The internal energy of n moles of an ideal monatomic (one atom per molecule) gas is equal to the average kinetic energy per molecule times the total number of molecules, N:
Eint = 3/2 NkT = 3/2 nRT
where n is the number of moles, each direction (x, y, and z) contributes (1/2)nRT to the internal energy. This is where the equipartition of energy idea comes in – any other contribution to the energy must also contribute (1/2)nRT. As can be seen, the internal energy of an ideal gas depends only on temperature and the number of moles of gas.
Diatomic Molecule
If the gas molecules contain more than one atom, there are three translation directions, and rotational kinetic energy contributes, but only for rotations about two of the three perpendicular axes. The five contributions to the energy (five degrees of freedom) give:
Diatomic ideal gas:
Eint = (5/2)NkT = (5/2)nRT
This is only an approximation and applies at intermediate temperatures. Only the translational kinetic energy contributes at low temperatures, and at higher temperatures, two additional contributions (kinetic and potential energy) come from vibration.
The internal energy will be greater at a given temperature than for a monatomic gas, but it will still be a function only of temperature for an ideal gas.
The internal energy of real gases also depends mainly on temperature. Still, similar to the Ideal Gas Law, real gases’ internal energy depends somewhat on pressure and volume. All real gases approach the ideal state at low pressures (densities). At low pressures, molecules are far enough apart that they do not interact with one another. The internal energy of liquids and solids is quite complicated, for it includes electrical potential energy associated with the forces (or “chemical” bonds) between atoms and molecules.
Joule’s Second Law
For any gas whose equation of state is given exactly by pV = nRT (or pv = RT), the specific internal energy depends on temperature only. This rule was originally found in 1843 by Joule experimentally for real gases and is known as Joule’s second law:
The internal energy of a fixed mass of an ideal gas depends only on its temperature (not pressure or volume).
The specific enthalpy of a gas described by pV = nRT also depends on temperature only. Note that enthalpy is the thermodynamic quantity equivalent to the total heat content of a system. It is equal to the internal energy of the system plus the product of pressure and volume. In intensive variables the Joule’s second law is therefore given by h = h(T) = u(T) + pv = u(T) + RT.
These three equations constitute the ideal gas model, summarized as follows:
pv = RT
u = u(T)
h = h(T) = u(T) + RT
Internal Energy and the First Law of Thermodynamics
In thermodynamics, the concept of energy is broadened to account for other observed changes. The principle of conservation of energy is extended to include a wide variety of ways systems interact with their surroundings. The only ways the energy of a closed system can be changed are through the transfer of energy by work or by heat. Further, based on the experiments of Joule and others, a fundamental aspect of the energy concept is that energy is conserved. This principle is known as the first law of thermodynamics. The first law of thermodynamics can be written in various forms:
In words:
Equation form:
∆Eint = Q – W
where Eint represents the internal energy of the material, which depends only on the material’s state (temperature, pressure, and volume). Q is the net heat added to the system, and W is the net work done by the system. We must be careful and consistent in following the sign conventions for Q and W. Because W in the equation is the work done by the system, then if work is done on the system, W will be negative, and Eint will increase.
Similarly, Q is positive for heat added to the system, so if heat leaves the system, Q is negative. This tells us the following: The internal energy of a system tends to increase if the system absorbs heat or if positive work is done on the system. Conversely, the internal energy tends to decrease if the system loses heat or if negative work is done on the system. It must be added Q and W are path-dependent, while Eint is path-independent.
Differential form:
dEint = dQ – dW
The internal energy Eint of a system tends to increase if energy is added as heat Q and tends to decrease if energy is lost as work W is done by the system.
See also: Open System – Closed System – Isolated System
Distinguishing Temperature, Heat, and Internal Energy
Using the kinetic theory, a clear distinction between these three properties can be made.
- Temperature is related to the kinetic energies of the molecules of a material. It is the average kinetic energy of individual molecules.
- Internal energy refers to the total energy of all the molecules within the object. Therefore, it is an extensive property when two equal-mass hot ingots of steel may have the same temperature, but two of them have twice as much internal energy as one does.
- Finally, heat is the amount of energy flowing spontaneously from one body to another due to their temperature difference.
It must be added, and when a temperature difference does exist, heat flows spontaneously from the warmer system to the colder system. Thus, if a 5 kg cube of steel at 100°C is placed in contact with a 500 kg cube of steel at 20°C, heat flows from the cube at 300°C to the cube at 20°C even though the internal energy of the 20°C cubes is much greater because there is so much more of it.
A particularly important concept is thermodynamic equilibrium. In general, when two objects are brought into thermal contact, heat will flow between them until they come into equilibrium with each other.
