
Natural uranium refers to uranium with the same isotopic ratio as found in nature. It consists primarily of isotope 238U (99.28%). Therefore the atomic mass of the uranium element is close to the atomic mass of the 238U isotope (238.03u). Natural uranium also consists of two other isotopes: 235U (0.71%) and 234U (0.0054%). The abundance of isotopes in nature is caused by differences in the half-lives. All three naturally-occurring isotopes of uranium (238U, 235U, and 234U) are unstable. On the other hand, these isotopes (except 234U) belong to primordial nuclides because their half-life is comparable to the age of the Earth (~4.5×109 years for 238U).
Since natural uranium contains only 0.71% of fissile isotope 235U and most current power reactors require enriched uranium, this natural uranium must be enriched. The level of enrichment required depends on specific reactor design (e.g.,, PWRs and BWRs require 3% – 5% of 235U) and specific requirements of the nuclear power plant operator. Without required enrichment, these reactors cannot initiate and sustain a nuclear chain reaction for such a long period as 12 months (or more).
The enrichment process separates gaseous uranium hexafluoride into two streams, enriched to the required level and known as low-enriched uranium. The other stream is progressively depleted in U-235 and is called ‘tails’, or depleted uranium. Typically, to produce 1 kg of enriched uranium with 5% of 235U, about 10 kg of natural uranium is required with a byproduct of about 9 kg of depleted uranium. Therefore annual natural uranium consumption of 3000MWth reactor is about 250 tonnes of natural uranium (to produce about 25 tonnes of enriched uranium).
Summary:
Consumption of a 3000MWth (~1000MWe) reactor (12-months fuel cycle)
It is an illustrative example, and the following data do not correspond to any reactor design.
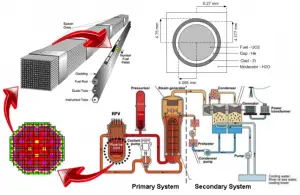
- A typical reactor may contain about 165 tonnes of fuel (including structural material)
- A typical reactor may contain about 100 tonnes of enriched uranium (i.e., about 113 tonnes of uranium dioxide).
- This fuel is loaded within, for example, 157 fuel assemblies composed of over 45,000 fuel rods.
- A common fuel assembly contains energy for approximately 4 years of operation at full power.
- Therefore about one-quarter of the core is yearly removed to the spent fuel pool (i.e., about 40 fuel assemblies). At the same time, the remainder is rearranged to a location in the core better suited to its remaining level of enrichment (see Power Distribution).
- The removed fuel (spent nuclear fuel) still contains about 96% of reusable material (it must be removed due to decreasing kinf of an assembly).
- The annual natural uranium consumption of this reactor is about 250 tonnes of natural uranium (to produce about 25 tonnes of enriched uranium).
- The annual enriched uranium consumption of this reactor is about 25 tonnes of enriched uranium.
- The annual fissile material consumption of this reactor is about 1 005 kg.
- The annual matter consumption of this reactor is about 1.051 kg.
- But it corresponds to about 3 200 000 tons of coal burned in coal-fired power plant per year.