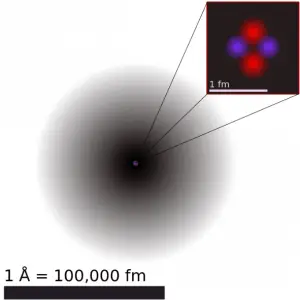
The atomic nucleus was discovered by Ernest Rutherford, who proposed a new model of the atom based on Geiger-Marsden experiments. These experiments were performed between 1908 and 1913 by Hans Geiger and Ernest Marsden under the direction of Ernest Rutherford. These experiments were a landmark series of experiments. Scientists discovered that every atom contains a nucleus (whose diameter is of the order of 10-14m). Its positive charge and most of its mass is concentrated in a small region called an atomic nucleus. In Rutherford’s atom, the diameter of its sphere (about 10-10 m) of influence is determined by its electrons. In other words, the nucleus occupies only about 10-12 of the total volume of the atom or less (the nuclear atom is largely empty space), but it contains all the positive charge and at least 99.95% of the total mass of the atom.
After discovering the neutron in 1932 by the English physicist James Chadwick, models for a nucleus composed of protons and neutrons were quickly developed by Dmitri Ivanenko and Werner Heisenberg.
All matter except dark matter is made of molecules, which are themselves made of atoms. The atoms consist of two parts. An atomic nucleus and an electron cloud are bound together by electrostatic force. The nucleus itself is generally made of protons and neutrons, but even these are composite objects. Inside the protons and neutrons, we find the quarks.
Inside the atomic nucleus, the residual strong force, also known as the nuclear force, holds neutrons and protons together in nuclei. In nuclei, this force acts against the enormous repulsive electromagnetic force of the protons. The term residual is associated with the fact that it is the residuum of the strong fundamental interaction between the quarks that make up the protons and neutrons. The residual strong force acts indirectly through the virtual π and ρ mesons, which transmit the force between nucleons that holds the nucleus together.
Properties of Nucleus
 The nuclear properties (atomic mass, nuclear cross-sections) of the element are determined by the number of protons (atomic number) and the number of neutrons (neutron number). For example, actinides with odd neutron numbers are usually fissile (fissionable with slow neutrons), while actinides with even neutron numbers are not fissile (but fissionable with fast neutrons). Due to the Pauli exclusion principle, heavy nuclei with an even number of protons and an even number of neutrons are very stable thanks to the occurrence of ‘paired spin.’ On the other hand, nuclei with an odd number of protons and neutrons are mostly unstable.
The nuclear properties (atomic mass, nuclear cross-sections) of the element are determined by the number of protons (atomic number) and the number of neutrons (neutron number). For example, actinides with odd neutron numbers are usually fissile (fissionable with slow neutrons), while actinides with even neutron numbers are not fissile (but fissionable with fast neutrons). Due to the Pauli exclusion principle, heavy nuclei with an even number of protons and an even number of neutrons are very stable thanks to the occurrence of ‘paired spin.’ On the other hand, nuclei with an odd number of protons and neutrons are mostly unstable.
Mass of Nucleus
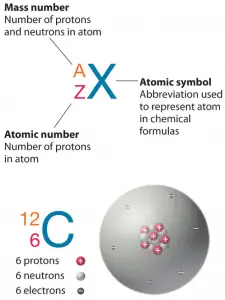 As was written, almost all of the mass of an atom is located in the nucleus, with a very small contribution from the electron cloud. The mass of the nucleus is associated with the atomic mass number, which is the total number of protons and neutrons in the nucleus of an atom. The mass number is different for each different isotope of a chemical element. The mass number is written either after the element name or as a superscript to the left of an element’s symbol. For example, the most common isotope of carbon is carbon-12 or 12C.
As was written, almost all of the mass of an atom is located in the nucleus, with a very small contribution from the electron cloud. The mass of the nucleus is associated with the atomic mass number, which is the total number of protons and neutrons in the nucleus of an atom. The mass number is different for each different isotope of a chemical element. The mass number is written either after the element name or as a superscript to the left of an element’s symbol. For example, the most common isotope of carbon is carbon-12 or 12C.
The size and mass of atoms are so small that the use of normal measuring units, while possible, is often inconvenient. Units of measure have been defined for mass and energy on the atomic scale to make measurements more convenient to express. The unit of measure for mass is the atomic mass unit (amu), and one atomic mass unit is equal to 1.66 x 10-24 grams.
Besides the standard kilogram, it is a second mass standard. It is the carbon-12 atom, which, by international agreement, has been assigned a mass of 12 atomic mass units (u). The relation between the two units is one atomic mass unit is equal:
1u = 1.66 x 10-24 grams.
One unified atomic mass unit is approximately the mass of one nucleon (either a single proton or neutron) and is numerically equivalent to 1 g/mol.
For 12C, the atomic mass is exactly 12u since the atomic mass unit is defined from it. The isotopic mass usually differs for other isotopes and is usually within 0.1 u of the mass number. For example, 63Cu (29 protons and 34 neutrons) has a mass number of 63, and an isotopic mass in its nuclear ground state is 62.91367 u.
There are two reasons for the difference between mass number and isotopic mass, known as the mass defect:
- The neutron is slightly heavier than the proton, increasing the mass of nuclei with more neutrons than protons relative to the atomic mass unit scale based on 12C with equal numbers of protons and neutrons.
- The nuclear binding energy varies between nuclei, and a nucleus with greater binding energy has lower total energy and a lower mass according to Einstein’s mass-energy equivalence relation E = mc2. For 63Cu, the atomic mass is less than 63, so this must be the dominant factor.
Note that it was found the rest mass of an atomic nucleus is measurably smaller than the sum of the rest masses of its constituent protons, neutrons, and electrons. Mass was no longer considered unchangeable in the closed system. The difference is a measure of the nuclear binding energy which holds the nucleus together. According to the Einstein relationship (E=mc2), this binding energy is proportional to this mass difference, known as the mass defect.
Radius and Density of Atomic Nucleus
Typical nuclear radii are of the order of 10−14 m. Assuming a spherical shape, nuclear radii can be calculated according to the following formula:
r = r0 . A1/3
where r0 = 1.2 x 10-15 m = 1.2 fm
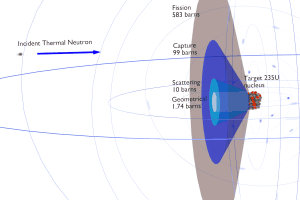
If we use this approximation, we expect the geometrical cross-sections of nuclei to be of the order of πr2 or 4.5×10−30 m² for hydrogen nuclei or 1.74×10−28 m² for 238U nuclei.
Nuclear density is the density of the nucleus of an atom, and it is the ratio of mass per unit volume inside the nucleus. Since the atomic nucleus carries most of the atom’s mass and the atomic nucleus is very small compared to the entire atom, the nuclear density is very high.
The nuclear density for a typical nucleus can be approximately calculated from the size of the nucleus and its mass. For example, natural uranium consists primarily of isotope 238U (99.28%). Therefore the atomic mass of the uranium element is close to the atomic mass of the 238U isotope (238.03u). The radius of this nucleus will be:
r = r0 . A1/3 = 7.44 fm.
Assuming it is spherical, its volume will be:
V = 4πr3/3 = 1.73 x 10-42 m3.
The usual definition of nuclear density gives for its density:
ρnucleus = m / V = 238 x 1.66 x 10-27 / (1.73 x 10-42) = 2.3 x 1017kg/m3.
Thus, the density of nuclear material is more than 2.1014 times greater than that of water, and it is an immense density. The descriptive term nuclear density is also applied to situations where similarly high densities occur, such as within neutron stars. Such immense densities are also found in neutron stars.
Excited Nucleus – Nuclear Resonance
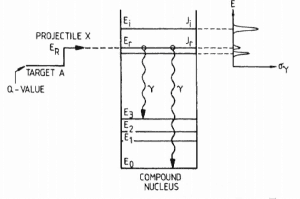
Highly excited nuclei formed by the combination of the incident particle and target nucleus are known as nuclear resonances. Suppose a target nucleus X is bombarded with particles a. In that case, it is sometimes observed that the ensuing nuclear reaction takes place with appreciable probability only if the energy of the particle a is in the neighborhood of certain definite energy values. These energy values are referred to as resonance energies. The compound nuclei of these certain energies are referred to as nuclear resonances. Resonances are usually found only at relatively low energies of the projectile. The widths of the resonances increase in general with increasing energies. At higher energies, the widths may reach the order of the distances between resonances, and then no resonances can be observed.