Facebook Instagram Youtube Twitter

Linear and Mass Attenuation Coefficient – X-rays

X-rays, also known as X-radiation, refer to electromagnetic radiation (no rest mass, no charge) of high energies. X-rays are high-energy photons with short wavelengths and thus very high frequency. The radiation frequency is the key parameter of all photons because it determines the energy of a photon. Photons are categorized according to their energies, from low-energy radio waves and infrared radiation, through visible light, to high-energy X-rays and gamma rays.

Most X-rays have a wavelength ranging from 0.01 to 10 nanometers (3×1016 Hz to 3×1019 Hz), corresponding to energies in the range of 100 eV to 100 keV. X-ray wavelengths are shorter than those of UV rays and typically longer than those of gamma rays. The distinction between X-rays and gamma rays is not so simple and has changed in recent decades. According to the currently valid definition, X-rays are emitted by electrons outside the nucleus, while gamma rays are emitted by the nucleus.

Linear Attenuation Coefficient – X-rays

The attenuation of X-rays can then be described by the following equation.

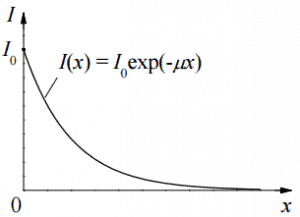
I=I0.e-μx

where I is intensity after attenuation,  Io is incident intensity,  μ is the linear attenuation coefficient (cm-1), and the physical thickness of the absorber (cm).

Attenuation
Dependence of gamma radiation intensity on absorber thickness

The materials listed in the table are air, water, and different elements from carbon (Z=6) through to lead (Z=82), and their linear attenuation coefficients are given for two X-ray energies. There are two main features of the linear attenuation coefficient:

  • The linear attenuation coefficient increases as the atomic number of the absorber increases.
  • The linear attenuation coefficient for all materials decreases with the energy of the X-rays.

Mass Attenuation Coefficient

We can sometimes use the mass attenuation coefficient when characterizing an absorbing material. The mass attenuation coefficient is defined as the ratio of the linear attenuation coefficient and absorber density (μ/ρ). The following equation can then describe the attenuation of X-rays:

I=I0.e-(μ/ρ).ρl

, where ρ is the material density, (μ/ρ) is the mass attenuation coefficient, and ρ.l is the mass thickness. The measurement unit was used for the mass attenuation coefficient cm2g-1. For intermediate energies, the Compton scattering dominates, and different absorbers have approximately equal mass attenuation coefficients. This is due to the fact that the cross-section of Compton scattering is proportional to the Z (atomic number). Therefore the coefficient is proportional to the material density ρ. At small values of X-ray energy, where the coefficient is proportional to higher powers of the atomic number Z (for photoelectric effect σf ~ Z3), the attenuation coefficient μ is not a constant.

See also calculator: Gamma activity to dose rate (with/without shield)

See also XCOM – photon cross-section DB: XCOM: Photon Cross Sections Database

Example:

How much water shielding do you require if you want to reduce the intensity of a 100 keV monoenergetic X-ray beam (narrow beam) to 1% of its incident intensity? The half-value layer for 100 keV X-rays in water is 4.15 cm, and the linear attenuation coefficient for 100 keV X-rays in water is 0.167 cm-1. The problem is quite simple and can be described by the following equation:

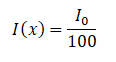If the half-value layer for water is 4.15 cm, the linear attenuation coefficient is: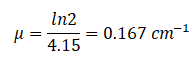Now, we can use the exponential attenuation equation: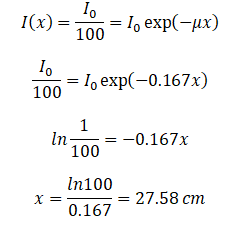x-ray attenuation - problem with solution

So the required thickness of water is about 27.58 cm. This is a relatively large thickness, and it is caused by small atomic numbers of hydrogen and oxygen. If we calculate the same problem for lead (Pb), we obtain the thickness x=0.077 cm.

Linear Attenuation Coefficients

Table of Linear Attenuation Coefficients (in cm-1) for different materials at photon energies of 100, 200, and 500 keV.

Absorber 100 keV 200 keV 500 keV
Air 0.0195/m 0.0159/m 0.0112/m
Water 0.167/cm 0.136/cm 0.097/cm
Carbon 0.335/cm 0.274/cm 0.196/cm
Aluminium 0.435/cm 0.324/cm 0.227/cm
Iron 2.72/cm 1.09/cm 0.655/cm
Copper 3.8/cm 1.309/cm 0.73/cm
Lead 59.7/cm 10.15/cm 1.64/cm
References:

Radiation Protection:

  1. Knoll, Glenn F., Radiation Detection and Measurement 4th Edition, Wiley, 8/2010. ISBN-13: 978-0470131480.
  2. Stabin, Michael G., Radiation Protection, and Dosimetry: An Introduction to Health Physics, Springer, 10/2010. ISBN-13: 978-1441923912.
  3. Martin, James E., Physics for Radiation Protection 3rd Edition, Wiley-VCH, 4/2013. ISBN-13: 978-3527411764.
  4. U.S.NRC, NUCLEAR REACTOR CONCEPTS
  5. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.

Nuclear and Reactor Physics:

  1. J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
  2. J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
  3. W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
  4. Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
  5. W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
  6. G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
  7. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.
  8. U.S. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993.
  9. Paul Reuss, Neutron Physics. EDP Sciences, 2008. ISBN: 978-2759800414.

See above:

X-rays