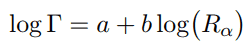This transition can be characterized as:
As can be seen from the figure, the alpha particle is emitted in alpha decay. Alpha particles are energetic nuclei of helium. Alpha particles consist of two protons and two neutrons bound together into a particle identical to a helium nucleus. Alpha particles are relatively large and carry a double positive charge. They are not very penetrating, and a piece of paper can stop them. They travel only a few centimeters but deposit all their energies along their short paths.
 In practice, this mode of decay has only been observed in nuclides considerably heavier than nickel, with the lightest known alpha emitters being the lightest isotopes (mass numbers 106–110) of tellurium (element 52). In nuclear reactors, alpha decay occurs, for example, in the fuel (alpha decay of heavy nuclei). Alpha particles are commonly emitted by all of the heavy radioactive nuclei occurring in nature (uranium, thorium, or radium), as well as the transuranic elements (neptunium, plutonium, or americium).
In practice, this mode of decay has only been observed in nuclides considerably heavier than nickel, with the lightest known alpha emitters being the lightest isotopes (mass numbers 106–110) of tellurium (element 52). In nuclear reactors, alpha decay occurs, for example, in the fuel (alpha decay of heavy nuclei). Alpha particles are commonly emitted by all of the heavy radioactive nuclei occurring in nature (uranium, thorium, or radium), as well as the transuranic elements (neptunium, plutonium, or americium).
Theory of Alpha Decay – Quantum Tunneling
Among the variety of channels in which a nucleus decays, alpha decay has been one of the most studied. The alpha decay channel in heavy and super heavy nuclei has provided information on the fundamental properties of nuclei far from stability, such as their ground state energies and the structure of their nuclear levels.
Alpha decay is a quantum tunneling process. To be emitted, the alpha particle must penetrate a potential barrier. This is similar to cluster decay, in which an atomic nucleus emits a small “cluster” of neutrons and protons (e.g., 12C).
The height of the Coulomb barrier for nuclei of A « 200 is about 20-25 MeV. The alpha particles emitted in nuclear decay have typical energies of about 5 MeV. On the one hand, an incoming 5 MeV alpha particle is scattered from a heavy nucleus. It cannot penetrate the Coulomb barrier and get sufficiently close to the nucleus to interact via the strong force. On the other hand, a 5 MeV alpha particle bound in a nuclear potential well can tunnel that same Coulomb barrier.
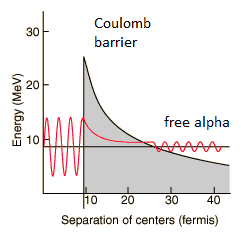 By 1928, George Gamow (and independently by Ronald Gurney and Edward Condon) had solved the theory of alpha decay via quantum tunneling. They assumed that the alpha particle and the daughter nucleus exist within the parent nucleus prior to its dissociation, namely the decay of quasistationary states (QS). A quasistationary state is defined as a long-lived state that eventually decays. Initially, the alpha cluster oscillates in the potential of the daughter nucleus, with the Coulomb potential preventing their separation. The alpha particle is trapped in a potential well by the nucleus. Classically, it is forbidden to escape, but according to the (then) newly discovered principles of quantum mechanics, it has a tiny (but non-zero) probability of “tunneling” through the barrier and appearing on the other side to escape the nucleus. Using the tunneling mechanism, Gamow, Condon, and Gurney calculated the penetrability of the tunneling α particle through the Coulomb barrier, finding the lifetimes of some α emitting nuclei. The main success of this model was the reproduction of the semi-empirical Geiger-Nuttall law that expresses the lifetimes of the α emitters in terms of the energies of the released α particles. It must be noted that other common forms of decay (e.g., beta decay) are governed by the interplay between nuclear and electromagnetic forces.
By 1928, George Gamow (and independently by Ronald Gurney and Edward Condon) had solved the theory of alpha decay via quantum tunneling. They assumed that the alpha particle and the daughter nucleus exist within the parent nucleus prior to its dissociation, namely the decay of quasistationary states (QS). A quasistationary state is defined as a long-lived state that eventually decays. Initially, the alpha cluster oscillates in the potential of the daughter nucleus, with the Coulomb potential preventing their separation. The alpha particle is trapped in a potential well by the nucleus. Classically, it is forbidden to escape, but according to the (then) newly discovered principles of quantum mechanics, it has a tiny (but non-zero) probability of “tunneling” through the barrier and appearing on the other side to escape the nucleus. Using the tunneling mechanism, Gamow, Condon, and Gurney calculated the penetrability of the tunneling α particle through the Coulomb barrier, finding the lifetimes of some α emitting nuclei. The main success of this model was the reproduction of the semi-empirical Geiger-Nuttall law that expresses the lifetimes of the α emitters in terms of the energies of the released α particles. It must be noted that other common forms of decay (e.g., beta decay) are governed by the interplay between nuclear and electromagnetic forces.
Special Reference: W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467.
Geiger-Nuttall Law
Geiger-Nuttall law is a semi-empirical law that expresses the lifetime (half-life) of the alpha emitter in terms of the energy of the released alpha particle. In other words, it states that short-lived isotopes emit more energetic alpha particles than long-lived ones. This rule was formulated by Hans Geiger and John Mitchell Nuttall in 1911, prior to the development of the theoretical formulation. Geiger-Nuttall law can be mathematically expressed as:
where a and b are empirical constants found from logarithmic plots of experimental data, Rα represents the linear range of alpha particles. Thus it is a direct measure of the kinetic energy of the alpha particle. The width of the resonance (Γ) is generally related to the mean lifetime (τ) of the excited nucleus by the relation: Γ = ℏ / τ
Conservation Laws in Alpha Decay
In analyzing nuclear reactions, we apply the many conservation laws. Nuclear reactions are subject to classical conservation laws for the charge, momentum, angular momentum, and energy(including rest energies). Additional conservation laws not anticipated by classical physics are:
- Law of Conservation of Lepton Number
- Law of Conservation of Baryon Number
- Law of Conservation of Electric Charge
Certain laws are obeyed under all circumstances, and others are not. We have accepted the conservation of energy and momentum. In all the examples, we assume that the number of protons and neutrons is separately conserved. We shall find circumstances and conditions in which this rule is not true. Where we are considering non-relativistic nuclear reactions, it is essentially true. However, when considering relativistic nuclear energies or those involving weak interactions, we shall find that these principles must be extended.
Some conservation principles have arisen from theoretical considerations, and others are just empirical relationships. Notwithstanding, any reaction not expressly forbidden by the conservation laws will generally occur, perhaps at a slow rate. This expectation is based on quantum mechanics. Unless the barrier between the initial and final states is infinitely high, there is always a non-zero probability that a system will make the transition between them.
For purposes of analyzing non-relativistic reactions, it is sufficient to note four of the fundamental laws governing these reactions.
- Conservation of nucleons. The total number of nucleons before and after a reaction are the same.
- Conservation of charge. The sum of the charges on all the particles before and after a reaction are the same.
- Conservation of momentum. The total momentum of the interacting particles before and after a reaction is the same.
- Conservation of energy. Energy, including rest mass energy, is conserved in nuclear reactions.
Reference: Lamarsh, John R. Introduction to Nuclear engineering 2nd Edition
Alpha Decay – Q-value
In nuclear and particle physics, the energetics of nuclear reactions is determined by the Q-value of that reaction. The Q-value of the reaction is defined as the difference between the sum of the rest masses of the initial reactants and the sum of the masses of the final products in energy units (usually in MeV).
Consider a typical reaction in which the projectile a and the target A give place to two products, B and b. This can also be expressed in the notation we have used so far, a + A → B + b, or even in a more compact notation, A(a,b)B.
See also: E=mc2
The Q-value of this reaction is given by:
Q = [ma + mA – (mb + mB)]c2
When describing alpha decay (a reaction without projectile), the disintegrating nucleus is usually referred to as the parent nucleus and the nucleus remaining after the event as the daughter nucleus. The total rest mass of the daughter nucleus and the nuclear radiation released in an alpha disintegration, mDaughter + mRadiation, is always less than that of the parent nucleus, mparent. The mass-energy difference,
Q = [mparent – (mDaughter + mRadiation)]c2
appears as the disintegration energy, liberated in the process. For example, the Q-value of typical alpha decay is:
The disintegration energy of about 5 MeV is the typical kinetic energy of the alpha particle. To fulfill the law of conservation of momentum, most of the disintegration energy must appear as the kinetic energy of the alpha particle. After an alpha or beta decay, the daughter nucleus is often left in an excited energy state. To stabilize itself, it subsequently emits high-energy photons, γ-rays.