The concentration of daughter nuclei in the radioactive equilibrium depends primarily on the proportions of parent and daughter nuclei’s half-lives (decay constants). Since the production and decay rates are equal, the number of atoms present remains constant over time. In any case, a radioactive equilibrium is not established immediately, but it only takes place after a transition period. This period is the order of a few half-lives of the longest-lived nucleus in the decay chain. In the case of radioactive decay chains, a radioactive equilibrium may be established between each member of the decay chain.
As was written, the proportionality of half-lives is a key parameter that determines the type of radioactive equilibrium:
- Radioactive equilibrium is not established when the half-life of the parent nucleus is shorter than the half-life of the daughter nucleus. In this case, the production rate and decay rate of a certain member of the decay chain cannot be equal.
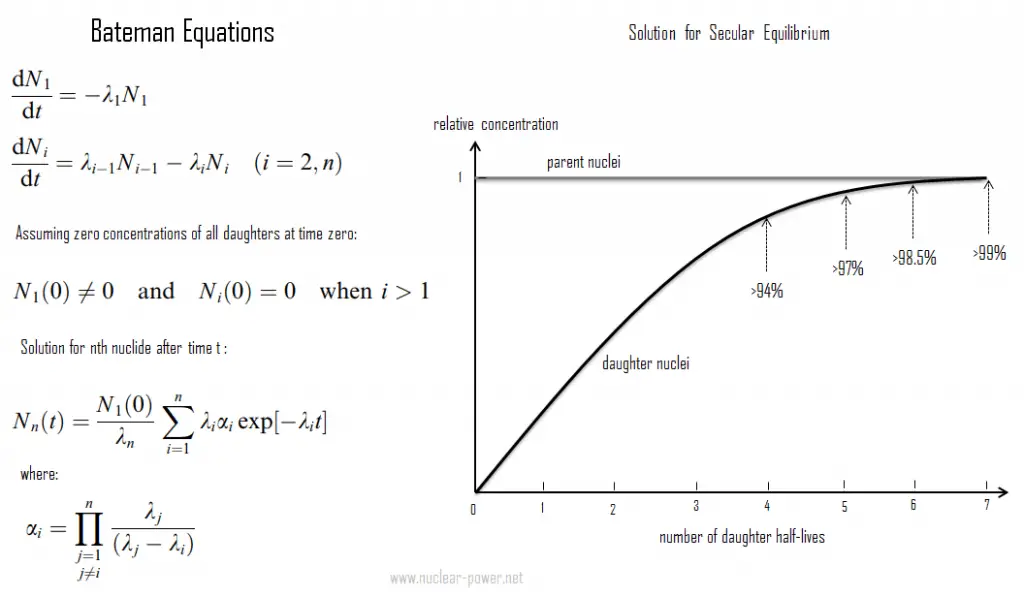
- Secular radioactive equilibrium exists when the parent nucleus has an extremely long half-life. This type of equilibrium is particularly important in nature. Over the 4.5 billion years of the Earth’s history, especially uranium 238, uranium 235, and thorium 232, members of their decay chains have reached radioactive equilibria between the parent nucleus and the various descendants.
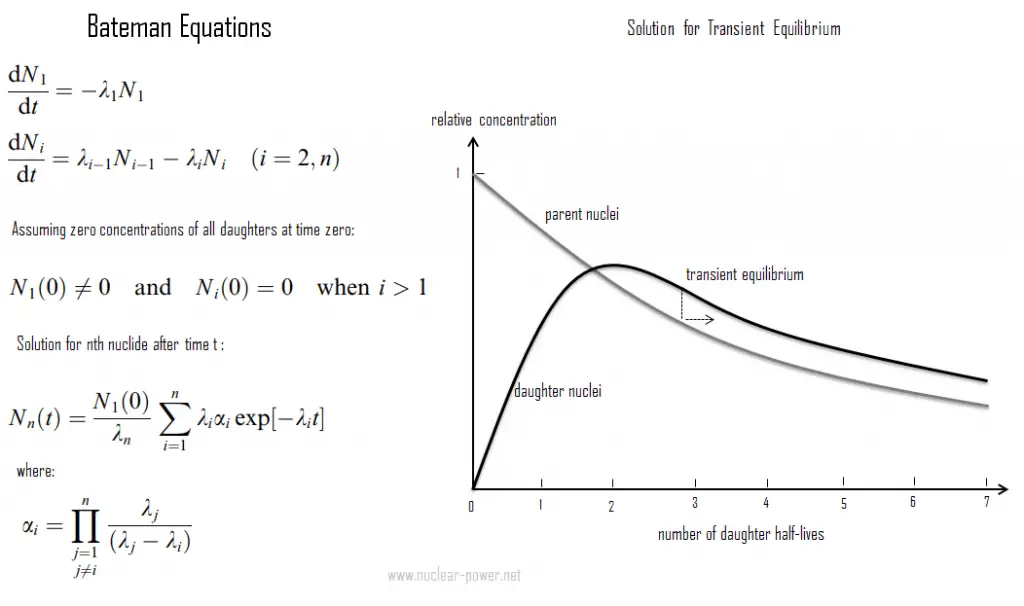
- Transient radioactive equilibrium exists when the half-life of the parent nucleus is longer than the half-life of the daughter nucleus. In this case, the parent nuclide and the daughter nuclide decay at the same rate.
Secular Equilibrium
Secular radioactive equilibrium exists when the parent nucleus has an extremely long half-life. Secular equilibrium is typical for natural radioactive series, such as the thorium series or the uranium series. For the uranium series with uranium-238 (with a half-life of 4.47 billion years), where all of the elements in the chain are in secular equilibrium, each of the descendants has built up to an equilibrium amount, and all decay at the rate set by the original parent. The only exception is the final stable element (lead-206) on the end of the chain, and its number of atoms is constantly increasing. In any case, a radioactive equilibrium is not established immediately, but it only takes place after a transition period. This period comprises a few half-lives of the longest-lived nucleus in the decay chain (234U for the uranium series; 231Pa for the actinium series). In the case of radioactive decay chains, a radioactive equilibrium may be established between each member of the decay chain.
As can be seen, the secular equilibrium is particularly important in nature. Over the 4.5 billion years of the Earth’s history, especially uranium 238, uranium 235, and thorium 232, members of their decay chains have reached radioactive equilibria between the parent nucleus and the various descendants. The half-lives of all their descendants are extremely variable, and it isn’t easy to represent a range of timescales going from individual seconds to millions of years. Since daughter radioisotopes have different half-lives, secular equilibrium is reached after some time. In the long decay chain for a naturally radioactive element, such as uranium-238, where all of the elements in the chain are in secular equilibrium, each of the descendants has built up to an equilibrium amount, and all decay at the rate set by the original parent. If and when equilibrium is achieved, each successive daughter isotope is present in direct proportion to its half-life (or decay constant).
Since its activity is inversely proportional to its half-life, each nuclide in the decay chain finally contributes as many individual transformations as the head of the chain. Radioactive cascade in the secular equilibrium significantly influences radioactivity (disintegrations per second) of natural samples and natural materials. All the descendants are present, at least transiently, in any natural sample, whether metal, compound or mineral. For example, pure uranium-238 is weakly radioactive (proportional to its long half-life). Still, aa uranium ore is about 13 times more radioactive (approximately 13 descendants in the decay chain) than the pure uranium-238 metal because of its daughter isotopes (e.g., radon, radium, etc.) it contains. Not only are unstable radium isotopes significant radioactivity emitters, but as the next stage in the decay chain, they also generate radon, a heavy, inert, naturally occurring radioactive gas. Moreover, the decay heat of uranium and its decay products (e.g., radon, radium, etc.) contributes to the heating of Earth’s core. Together with thorium and potassium-40 in the Earth’s mantle, these elements are the main source of heat that keeps the Earth’s core liquid.
The secular equilibrium can occasionally be disrupted when one intermediary nucleus leaves the sample (e.g., radon released from the soil) where its ancestors are confined. These local disruptions are important to consider in the use of dating techniques. Disruption of the secular equilibrium also influences the radioactivity of fresh nuclear fuel. Most PWRs use the uranium fuel, which is in the form of uranium dioxide. But this uranium dioxide must be chemically purified, and most of its decay chain is not present in the fresh nuclear fuel.
Radioactive Equilibrium of Uranium-234
Isotope of uranium-234 is a member of the uranium series. This isotope has a half-life of only 2.46×105 years; therefore, it does not belong to primordial nuclides (unlike 235U and 238U). On the other hand, this isotope is still present in the Earth’s crust, but this is because 234U is an indirect decay product of 238U. 238U decays via alpha decay into 234U. 234U decays via alpha decay into 230Th, except a very small fraction (on the order of ppm) of nuclei decays by spontaneous fission.
In a natural sample of uranium, these nuclei are present in the unalterable proportions of the radioactive equilibrium of the 238U filiation at a ratio of one atom of 234U for about 18 500 nuclei of 238U. As a result of this equilibrium, these two isotopes (238U and 234U) contribute equally to the radioactivity of natural uranium.
Transient Radioactive Equilibrium
The transient radioactive equilibrium exists when the half-life of the parent nucleus is longer than the half-life of the daughter nucleus. Still, the concentration of parent nuclei significantly decreases in time. In this case, the parent nuclide and the daughter nuclide may decay at essentially the same rate, but both concentrations of nuclides decrease as the concentration of parent nuclei decreases. Contrary to secular equilibrium, the half-life of the daughter nuclei is not negligible compared to the parent’s half-life.
An example of this compound decay process is a Technetium-99m generator producing technetium-99m for nuclear medicine diagnostic procedures from molybdenum-99. Technetium-99 m’s short half-life of 6 hours makes storage impossible and would make transport very expensive. Therefore, for medical purposes, molybdenum-99 is used to produce technetium-99m. These two isotopes are in the transient equilibrium. The decay constant for molybdenum-99 is considerably smaller than the decay constant for technetium-99m. Although the decay constant for molybdenum-99 is smaller, the actual decay rate is initially larger than that of molybdenum-99 because of the great difference in their initial concentrations. As the daughter’s concentration increases, the daughter’s decay rate will approach and eventually match the decay rate of the parent. When this occurs, they are said to be in the transient equilibrium. In the case of Technetium-99m generator, transient equilibrium occurs after about four half-lives. Today, technetium-99m is the most utilized element in nuclear medicine and is employed in various nuclear medicine imaging studies.
Also, the transient equilibrium can occasionally be disrupted when one intermediary nucleus leaves the sample where its ancestors are confined.
Transient Radioactive Equilibrium with Source – Example
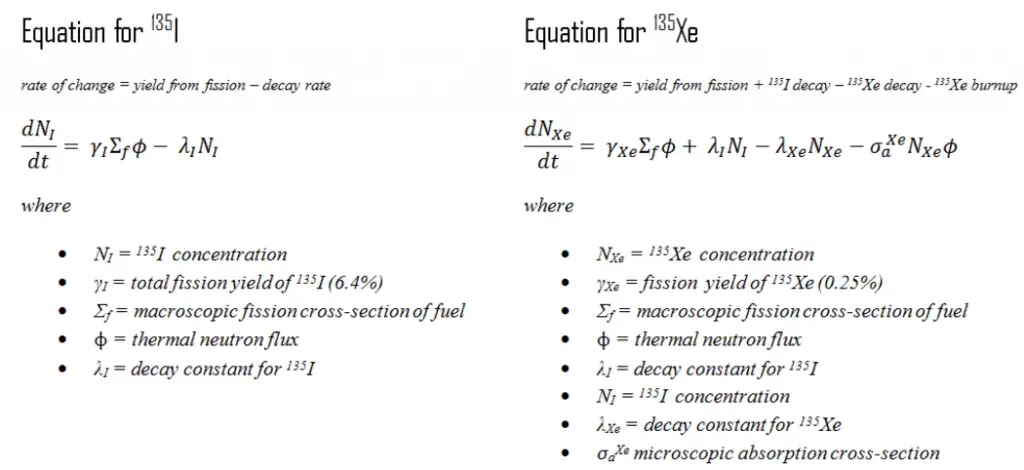
A special example of radioactive equilibrium is concentrations of iodine-135 and xenon-135 in a nuclear reactor, but in this case, the xenon burnup must be considered. Note that, in this special case, the half-life of the parent nucleus is shorter than the half-life of the daughter nucleus. The production and removal of xenon can be characterized by the following differential equations:
 When the rate of production of iodine equals the rate of removal of iodine, equilibrium exists. This equilibrium is also known as the “xenon 135 reservoir” since all of this iodine must undergo decay to xenon. In equilibrium, the iodine concentration remains constant and is designated NI(eq). The following equation for the iodine equilibrium concentration can be determined from the preceding equation by setting the dNI/dt =0. Since the equilibrium iodine concentration is proportional to the fission reaction rate, it is also proportional to the reactor power level.
When the rate of production of iodine equals the rate of removal of iodine, equilibrium exists. This equilibrium is also known as the “xenon 135 reservoir” since all of this iodine must undergo decay to xenon. In equilibrium, the iodine concentration remains constant and is designated NI(eq). The following equation for the iodine equilibrium concentration can be determined from the preceding equation by setting the dNI/dt =0. Since the equilibrium iodine concentration is proportional to the fission reaction rate, it is also proportional to the reactor power level.
When the rate of production of xenon 135 equals the rate of removal, equilibrium also exists for xenon. The xenon concentration remains constant and is designated NXe(eq). The following equation (1) for the xenon equilibrium concentration can be determined from the preceding equation by setting the dNXe/dt =0. For xenon 135 to be in equilibrium, iodine 135 must also be in equilibrium. Substituting the expression for equilibrium iodine 135 concentration into the equation for equilibrium xenon (1) results in the following (2).
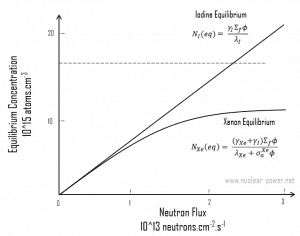
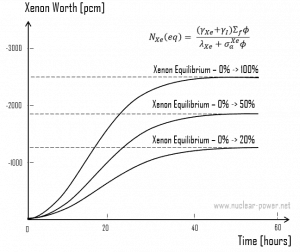 This equation shows that the equilibrium value for xenon 135 increases as power increases because the numerator is proportional to the fission reaction rate. But the thermal flux is also in the denominator. Therefore, as the thermal flux exceeds some value, the xenon burnup begins to dominate, and at approximately 1015 neutrons.cm-2.s-1, the xenon-135 concentration approaches a limiting value. The equilibrium iodine 135 and xenon 135 concentrations as a function of neutron flux are illustrated in the following figure.
This equation shows that the equilibrium value for xenon 135 increases as power increases because the numerator is proportional to the fission reaction rate. But the thermal flux is also in the denominator. Therefore, as the thermal flux exceeds some value, the xenon burnup begins to dominate, and at approximately 1015 neutrons.cm-2.s-1, the xenon-135 concentration approaches a limiting value. The equilibrium iodine 135 and xenon 135 concentrations as a function of neutron flux are illustrated in the following figure.
Bateman Equations
In physics, the Bateman equations are a set of first-order differential equations which describe the time evolution of nuclide concentrations undergoing serial or linear decay chain. Ernest Rutherford formulated the model in 1905, and the analytical solution for the case of radioactive decay in a linear chain was provided by Harry Bateman in 1910. This model can also be used in nuclear depletion codes to solve nuclear transmutation and decay problems.
For example, ORIGEN is a computer code system for calculating radioactive materials’ buildup, decay, and processing. ORIGEN uses a matrix exponential method to solve a large system of coupled, linear, first-order ordinary differential equations (similar to the Bateman equations) with constant coefficients.
The Bateman equations for radioactive decay case of n – nuclide series in linear chain describing nuclide concentrations are as follows:
Bateman Equations for Nuclear Transmutation
As was written, this model can also be used in nuclear depletion codes to solve nuclear transmutation and decay problems. In the case of transmutation, the decay constants that govern Bateman equations for a decay case are substituted by transmutation constants. By the transmutation constant λi,j, we understand the probability of the ith nuclide production per time unit from the jth nuclide destruction as a result of nuclear interaction with the whole spectrum of interacting particles or due to the natural nuclear decay.
These equations are usually used for the exact evolution of isotopic changes in the nuclear fuel during fuel depletion. Fuel depletion is usually modeled mathematically as a set of differential equations known as evolution equations.
Special Reference: Jerzy Cetnar, General solution of Bateman equations for nuclear transmutations. Annals of Nuclear Energy 33 (2006). January 2006.