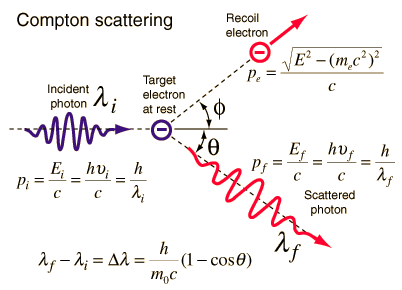
Source: hyperphysics.phy-astr.gsu.edu
Compton scattering is the inelastic or nonclassical scattering of a photon (which may be an X-ray or gamma-ray photon) by a charged particle, usually an electron. In Compton scattering, the incident gamma-ray photon is deflected through an angle Θ with respect to its original direction. This deflection decreases the photon’s frequency’s energy (decrease in photon’s frequency) and is called the Compton effect. The photon transfers a portion of its energy to the recoil electron. The energy transferred to the recoil electron can vary from zero to a large fraction of the incident gamma-ray energy because all scattering angles are possible. A. H.Compton observed the Compton scattering in 1923 at Washington University in St. Louis. Compton earned the Nobel Prize in Physics in 1927 for this new understanding of the particle nature of photons.
Key characteristics of Compton Scattering
- Compton scattering dominates at intermediate energies.
- It is the scattering of photons by atomic electrons.
- Photons undergo a wavelength shift called the Compton shift.
- The energy transferred to the recoil electron can vary from zero to a large fraction of the incident gamma-ray energy.
Compton Scattering Formula
The Compton formula was published in 1923 in the Physical Review. Compton explained that the particle-like momentum of photons causes the X-ray shift. Compton scattering formula is the mathematical relationship between the shift in wavelength and the scattering angle of the X-rays. In the case of Compton scattering, the photon of frequency f collides with an electron at rest. The photon bounces off the electron upon collision, giving up some of its initial energy (given by Planck’s formula E=hf). While the electron gains momentum (mass x velocity), the photon cannot lower its velocity. As a result of momentum conservation law, the photon must lower its momentum given by: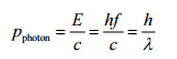
So the decrease in photon’s momentum must be translated into a decrease in frequency (increase in wavelength Δλ = λ’ – λ). The shift of the wavelength increased with scattering angle according to the Compton formula:
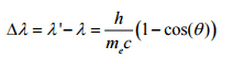where λ is the initial wavelength of photonλ’ is the wavelength after scattering,h is the Planck constant = 6.626 x 10-34 J.s me is the electron rest mass (0.511 MeV) c is the speed of light Θ is the scattering angle. The minimum change in wavelength (λ′ − λ) for the photon occurs when Θ = 0° (cos(Θ)=1) and is at least zero. The maximum change in wavelength (λ′ − λ) for the photon occurs when Θ = 180° (cos(Θ)=-1). In this case, the photon transfers to the electron as much momentum as possible. The maximum change in wavelength can be derived from the Compton formula:
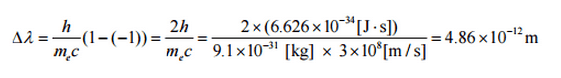The quantity h/mec is known as the Compton wavelength of the electron and is equal to 2.43×10−12 m.
Compton Scattering – Cross-Sections
The probability of Compton scattering per one interaction with an atom increases linearly with atomic number Z because it depends on the number of electrons available for scattering in the target atom. The Klein-Nishina formula describes the angular distribution of photons scattered from a single free electron: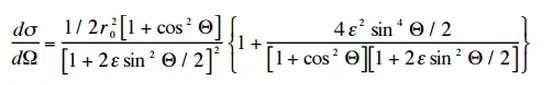
where ε = E0/mec2 and r0 is the “classical radius of the electron” equal to about 2.8 x 10-13 cm. The formula gives the probability of scattering a photon into the solid angle element dΩ = 2π sin Θ dΘ when the incident energy is E0.
Source: hyperphysics.phy-astr.gsu.edu/
Cross section of compton scattering of photons by atomic electrons..
Energies of a photon at 500 keV and an electron after Compton scattering.
Compton Edge
In spectrophotometry, the Compton edge is a feature of the spectrograph that results from the Compton scattering in the scintillator or detector. This feature is due to photons that undergo Compton scattering with a scattering angle of 180° and then escape the detector. When a gamma-ray scatters off the detector and escapes, only a fraction of its initial energy can be deposited in the sensitive layer of the detector. It depends on the scattering angle of the photon, how much energy will be deposited in the detector. This leads to a spectrum of energies. The Compton edge energy corresponds to a full backscattered photon.
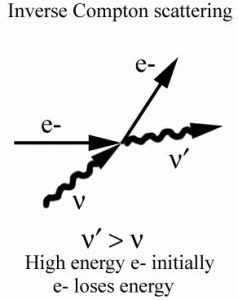
Inverse Compton Scattering
Inverse Compton scattering is the scattering of low-energy photons to high energies by relativistic electrons. Relativistic electrons can boost the energy of low-energy photons by a potentially enormous amount (even gamma rays can be produced). This phenomenon is very important in astrophysics.
Compton edge of 60Co on gamma spectrometer Na(Tl).
source: venables.asu.edu
