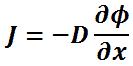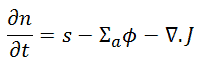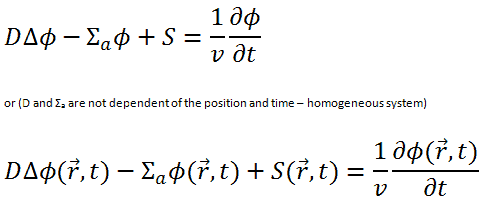The derivation of the diffusion equation depends on Fick’s law, which states that solute diffuses from high concentration to low. But first, we have to define a neutron flux and neutron current density. The neutron flux is used to characterize the neutron distribution in the reactor, and it is the main output of solutions of diffusion equations. The neutron flux, φ, does not characterize the flow of neutrons. There may be no flow of neutrons, yet many interactions may occur (I = Σ.φ). The neutrons move in random directions and hence may not flow. Therefore the neutron flux φ is more closely related to densities. Neutrons will exhibit a net flow when there are spatial differences in their density. Hence we can have a flux of neutron flux! This flux of neutron flux is called the neutron current density.
Fick’s Law
In chemistry, Fick’s law states that:
Suppose the concentration of a solute in one region is greater than in another of a solution. In that case, the solute diffuses from the higher concentration region to the lower concentration region, with a magnitude proportional to the concentration gradient.
In one (spatial) dimension, the law is:
where:
- J is the diffusion flux,
- D is the diffusion coefficient,
- φ (for ideal mixtures) is the concentration.
The use of this law in nuclear reactor theory leads to the diffusion approximation.
Fick’s law in reactor theory stated that:
The current density vector J is proportional to the negative of the gradient of the neutron flux. The proportionality constant is called the diffusion coefficient and is denoted by the symbol D.
In one (spatial) dimension, the law is:
where:
- J is the neutron current density (neutrons.cm-2.s-1) along the x-direction, the net flow of neutrons that pass per unit of time through a unit area perpendicular to the x-direction.
- D is the diffusion coefficient, it has the unit of cm, and it is given by:
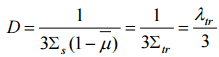
- φ is the neutron flux, the number of neutrons crossing through some arbitrary cross-sectional unit area in all directions per unit time.
The generalized Fick’s law (in three dimensions) is:
where J denotes the diffusion flux vector. Note that the gradient operator turns the neutron flux, a scalar quantity, into the neutron current, a vector quantity.
See also: Validity of Fick’s Law
Physical Interpretation
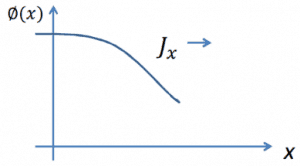The physical interpretation is similar to the fluxes of gases. The neutrons exhibit a net flow in the direction of least density. This is a natural consequence of greater collision densities at positions of greater neutron densities.
Consider neutrons passing through the plane at x=0 from left to right due to collisions to the left of the plane. Since the concentration of neutrons and the flux is larger for negative values of x, there are more collisions per cubic centimeter on the left. Therefore more neutrons are scattered from left to right, then the other way around. Thus the neutrons naturally diffuse toward the right.
Neutron Balance – Continuity Equation
The mathematical formulation of neutron diffusion theory is based on the balance of neutrons in a differential volume element. Since neutrons do not disappear (β decay is neglected), the following neutron balance must be valid in an arbitrary volume V.
rate of change of neutron density = production rate – absorption rate – leakage rate
where
Substituting for the different terms in the balanced equation and by dropping the integral over (because the volume V is arbitrary), we obtain:
where
- n is the density of neutrons,
- s is the rate at which neutrons are emitted from sources per cm3 (either from external sources (S) or from fission (ν.Σf.Ф)),
- J is the neutron current density vector
- Ф is the scalar neutron flux
- Σa is the macroscopic absorption cross-section
In steady state, when n is not a function of time:
The Diffusion Equation
In previous chapters, we introduced two bases for the derivation of the diffusion equation:
Fick’s law:
which states that neutrons diffuse from high concentration (high flux) to low concentration.
Continuity equation:
which states that rate of change of neutron density = production rate – absorption rate – leakage rate.
We return now to the neutron balance equation and substitute the neutron current density vector by J = -D∇Ф. Assuming that ∇.∇ = ∇2 = Δ (therefore div J = -D div (∇Ф) = -DΔФ) we obtain the diffusion equation.
