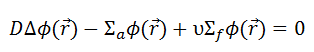Solutions of the Diffusion Equation – Multiplying Systems
This condition can be expressed conveniently in terms of the multiplication factor. The infinite multiplication factor is the ratio of the neutrons produced by fission in one neutron generation to the number of neutrons lost through absorption in the preceding neutron generation. This can be mathematically expressed as shown below.
The infinite multiplication factor in a multiplying system measures the change in the fission neutron population from one neutron generation to the subsequent generation.
- k∞ < 1. Suppose the multiplication factor for a multiplying system is less than 1.0. In that case, the number of neutrons decreases in time (with the mean generation time), and the chain reaction will never be self-sustaining. This condition is known as the subcritical state.
- k∞ = 1. If the multiplication factor for a multiplying system is equal to 1.0, then there is no change in neutron population in time, and the chain reaction will be self-sustaining. This condition is known as the critical state.
- k∞ > 1. If the multiplication factor for a multiplying system is greater than 1.0, then the multiplying system produces more neutrons than are needed to be self-sustaining. The number of neutrons is exponentially increasing in time (with the mean generation time). This condition is known as the supercritical state.
in various geometries that satisfy the boundary conditions. In this equation ν is number of neutrons emitted in fission and Σf is macroscopic cross-section of fission reaction. Ф denotes a reaction rate. For example a fission of 235U by thermal neutron yields 2.43 neutrons.
It must be noted that we will solve the diffusion equation without any external source. This is very important because such an equation is a linear homogeneous equation in the flux. Therefore if we find one solution of the equation, then any multiple is also a solution. This means that the absolute value of the neutron flux cannot possibly be deduced from the diffusion equation. This is different from problems with external sources, which determine the absolute value of the neutron flux.
We will start with simple systems (planar) and increase complexity gradually. The most important assumption is that all neutrons are lumped into a single energy group, they are emitted and diffuse at thermal energy (0.025 eV). Solutions of diffusion equations in this case provides an illustrative insights, how can be the neutron flux distributed in a reactor core.
Solution for the Infinite Slab Reactor
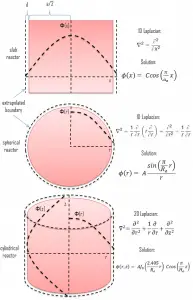
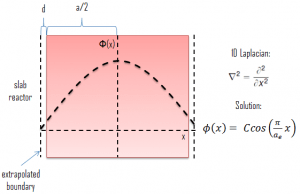Let us assume a uniform reactor (multiplying system) in the shape of a slab of physical width a in the x-direction and infinite in the y- and z-directions. This reactor is situated in the center at x=0. In this geometry, the flux does not vary in y and z allowing us to eliminate the y and z derivatives from ∇2. The flux is then a function of x only, and therefore the Laplacian and diffusion equation can be written as:
The quantity Bg2 is called the geometrical buckling of the reactor and depends only on the geometry. This term is derived from the notion that the neutron flux distribution is somehow “buckled” in a homogeneous finite reactor. It can be derived the geometrical buckling is the negative relative curvature of the neutron flux (Bg2 = ∇2Ф(x) / Ф(x)). The neutron flux has more concave downward or “buckled” curvature (higher Bg2) in a small reactor than in a large one. This is a very important parameter, and it will be discussed in the following sections.
For x > 0, this diffusion equation has two possible solutions sin(Bgx) and cos(Bgx), which give a general solution:
Φ(x) = A.sin(Bgx) + C.cos(Bg x)
From finite flux condition (0≤ Φ(x) < ∞), which required only reasonable values for the flux, it can be derived that A must be equal to zero. The term sin(Bgx) goes to negative values as x goes to negative values, and therefore it cannot be part of a physically acceptable solution. The physically acceptable solution must then be:
Φ(x) = C.cos(Bg x)
where Bg can be determined from the vacuum boundary condition.
The vacuum boundary condition requires the relative neutron flux near the boundary to have a slope of -1/d, i.e., the flux would extrapolate linearly to 0 at a distance d beyond the boundary. This zero flux boundary condition is more straightforward and can be written mathematically as:
If d is not negligible, physical dimensions of the reactor are increased by d, and an extrapolated boundary is formulated with dimension ae/2 = a/2 + d. This condition can be written as Φ(a/2 + d) = Φ(ae/2) = 0.
Therefore, the solution must be Φ(ae/2) = C.cos(Bg .ae/2) = 0 and the values of geometrical buckling, Bg, are limited to Bg = nπ/a_e, where n is any odd integer. The only one physically acceptable odd integer is n=1 because higher values of n would give cosine functions which would become negative for some values of x. The solution of the diffusion equation is:
It must be added the constant C cannot be obtained from this diffusion equation because this constant gives the absolute value of neutron flux. The neutron flux can have any value, and the critical reactor can operate at any power level. It should be noted the cosine flux shape is only in a hypothetical case in a uniform homogeneous reactor at low power levels (at “zero power criticality”).
In the power reactor core (at full power operation), the neutron flux can reach, for example, about 3.11 x 1013 neutrons.cm-2.s-1, but this value depends significantly on many parameters (type of fuel, fuel burnup, fuel enrichment, position in fuel pattern, etc.).
The power level does not influence the criticality (keff) of a power reactor unless thermal reactivity feedbacks act (operation of a power reactor without reactivity feedbacks is between 10E-8% – 1% of rated power).
