Neutron Transport Theory
Neutron transport theory is concerned with the transport of neutrons through various media. As was discussed, neutrons are neutral particles. Therefore they travel in straight lines, deviating from their path only when they collide with a nucleus to be scattered into a new direction or absorbed.
Transport theory is relatively simple in principle, and an exact transport equation governing this phenomenon can easily be derived. This equation is called the Boltzmann transport equation, and the entire study of transport theory focuses on this equation. In general, the neutron balance can be expressed graphically as:
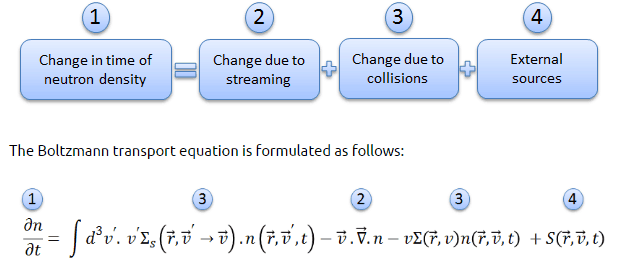
Boltzmann Transport Equation
The Boltzman transport equation is a balance statement that conserves neutrons. Each term represents a gain or a loss of a neutron, and the balance, in essence, claims that neutrons gained equals neutrons lost. It must be added that it is much easier to derive the Boltzmann transport equation than to solve it. Deterministic methods solve the Boltzmann transport equation in a numerically approximated manner everywhere throughout a modeled system. This task demands enormous computational resources because the problem has many dimensions. But the Boltzmann transport equation can be treated in a rather straightforward way. This simplified version of the Boltzmann transport equation is just the neutron diffusion equation. Naturally, many assumptions have to be fulfilled when using the diffusion equation, but the diffusion equation usually provides a sufficiently accurate approximation to the exact transport equation.
Nowadays, reactor core analyses and design can be performed using nodal two-group diffusion methods. These methods are based on pre-computed assembly homogenized cross-sections and assembly discontinuity factors (pin factors) obtained by single assembly calculation with reflective boundary conditions (infinite lattice). Two methods exist for the calculation of the pre-computed assembly cross-sections and pin factors.
- Deterministic methods that solve the Boltzmann transport equation.
- Stochastic methods are known as Monte Carlo methods that model the problem almost exactly.
These methods are very efficient and accurate when applied to the current Pressurized Water Reactors (PWRs) or Boiling Water Reactors (BWRs).