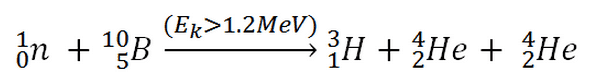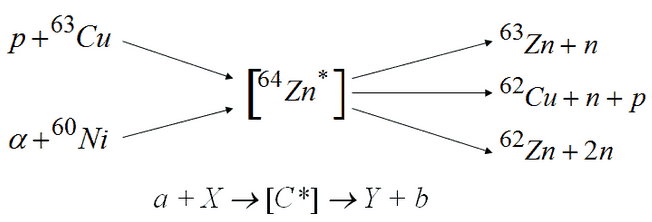The study of neutron nuclear reactions and nuclear reactions, in general, is of paramount importance in the physics of nuclear reactors. Progress in the understanding of nuclear reactions generally has occurred faster than similar studies of chemical reactions. Generally, a higher level of sophistication has been achieved.
 Shortly after the neutron was discovered in 1932, it was quickly realized that neutrons might act to form a nuclear chain reaction. When nuclear fission was discovered in 1938, it became clear that if a neutron-induced fission reaction produces new free neutrons, each of these neutrons might cause further fission reaction in a cascade known as a chain reaction. Therefore the calculations of nuclear reactors are determined by the transport of neutrons, their interaction with matter, and their multiplication within a nuclear reactor.
Shortly after the neutron was discovered in 1932, it was quickly realized that neutrons might act to form a nuclear chain reaction. When nuclear fission was discovered in 1938, it became clear that if a neutron-induced fission reaction produces new free neutrons, each of these neutrons might cause further fission reaction in a cascade known as a chain reaction. Therefore the calculations of nuclear reactors are determined by the transport of neutrons, their interaction with matter, and their multiplication within a nuclear reactor.
Definition
A neutron nuclear reaction is considered when a neutron interacts with a nuclear particle to produce two or more nuclear particles or ˠ-rays (gamma rays). Thus, a neutron nuclear reaction must cause a transformation of the target nuclide to another nuclide. Sometimes if a nucleus interacts with another nucleus or particle without changing the nature of the nuclide, the process is referred to as a nuclear scattering rather than a neutron nuclear reaction.
To understand the nature of neutron nuclear reactions, the classification according to the time scale of these reactions has to be introduced. Interaction time is critical for defining the reaction mechanism.
There are two extreme scenarios for nuclear reactions (not only neutron reactions):
- A projectile and a target nucleus are within the range of nuclear forces for a very short time allowing for an interaction of a single nucleon only. These types of reactions are called direct reactions.
- A projectile and a target nucleus are within the range of nuclear forces, allowing for a large number of interactions between nucleons. These types of reactions are called the compound nucleus reactions.
There is always some non-direct (multiple internuclear interactions) component in all reactions, but the direct reactions have this component limited.
Basic characteristics of direct reactions:
- The direct reactions are fast and involve a single-nucleon interaction.
- The interaction time must be very short (~10-22 s).
- The direct reactions require incident particle energy larger than ∼ 5 MeV/Ap. (Ap is the atomic mass number of a projectile)
- Incident particles interact on the surface of a target nucleus rather than in the volume of a target nucleus.
- Products of the direct reactions are not distributed isotropically in angle, but they are forward-focused.
- Direct reactions are of importance in measurements of nuclear structure.
Basic characteristics of compound nucleus reactions:
- The compound nucleus is a relatively long-lived intermediate state of the particle-target composite system.
- The compound nucleus reactions involve many nucleon-nucleon interactions.
- A large number of collisions between the nucleons leads to a thermal equilibrium inside the compound nucleus.
- The time scale of compound nucleus reactions is of the order of 10-18 s – 10-16 s.
- The compound nucleus reactions are usually created if the projectile has low energy.
- Incident particles interact in the volume of a target nucleus.
- Products of the compound nucleus reactions are distributed near isotropically in angle (the nucleus loses memory of how it was created – Bohr’s hypothesis of independence).
- The decay mode of the compound nucleus does not depend on how the compound nucleus is formed.
- Resonances in the cross-section are typical for the compound nucleus reaction.
Direct reactions have another very important property. Products of a direct reaction are not distributed isotropically in angle, but they are forward-focused. This reflects that the projectiles make only one, or very few, collisions with nucleons in the target nucleus, and its forward momentum is not transferred to an entire compound state.
The cross-sections for direct reactions vary smoothly and slowly with energy in contrast to the compound nucleus reactions. These cross-sections are comparable to the geometrical cross-sections of target nuclei.
Types of direct reactions:
- Elastic scattering in which a passing particle and targes stay in their ground states.
- Inelastic scattering in which a passing particle changes its energy state. For example, the (p, p’) reaction.
- Transfer reactions in which one or more nucleons are transferred to the other nucleus. These reactions are further classified as:
- Stripping reaction in which one or more nucleons are transferred to a target nucleus from passing particles. For example, the neutron stripping in the (d, p) reaction.
- Pick-up reaction in which one or more nucleons are transferred from a target nucleus to a passing particle. For example, the neutron pick-up in the (p, d) reaction.
- Break-up reaction in which a breakup of a projectile into two or more fragments occurs.
- Knock-out reaction in which a single nucleon or a light cluster is removed from the projectile by a collision with the target.
Example: This threshold reaction of a fast neutron with an isotope 10B is one of the ways how radioactive tritium in the primary circuit of all PWRs is generated.
This compound system is a relatively long-lived intermediate state of the particle-target composite system. From the definition, the compound nucleus must live for at least several times longer than is the time of transit of an incident particle across the nucleus (~10-22 s). The time scale of compound nucleus reactions is of the order of 10-18 s – 10-16 s, but lifetimes as long as 10-14 s have also been observed.
A very important feature and a direct consequence of the thermal equilibrium inside a compound nucleus is that the mode of decay of the compound nucleus does not depend on how the compound nucleus is formed. Many collisions between nucleons lead to the loss of information on the entrance channel from the system. The decay mechanism (channel) that dominates the decay of C* is determined by the excitation energy in C*.
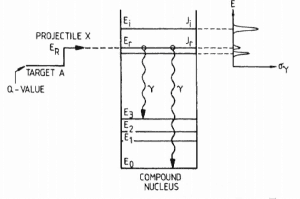
These reactions can be considered as two-stage processes.
- The first stage is the formation of a compound nucleus expressed by σa+X➝C*
- The second stage is the decay of a compound nucleus expressed by PC*➝b+Y
- The result cross-section of certain reaction a+X➝[C*]➝b+Y is given by σ(a,b)= σa+X➝C* . PC*➝b+Y
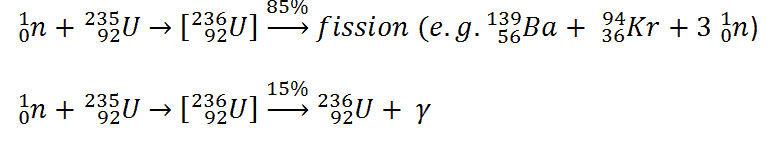
For the compound nucleus, peaks in the cross-section are typical. Each peak manifests a particular compound state of the nucleus. These peaks and the associated compound nuclei are usually called resonances. The behavior of the cross-section between two resonances is usually strongly affected by the effect of nearby resonances.
Resonances (particular compound states) are mostly created in neutron nuclear reactions, but it is by no means restricted to neutron nuclear reactions. The quantum nature of nuclear forces causes the formation of resonances. Each nuclear reaction is a transition between different quantum discrete states or energy levels. The discrete nature of energy transitions plays a key role. Suppose the energy of the projectile (the sum of the Q value and the kinetic energy of the projectile) and the energy of the target nucleus are equal to a compound nucleus at one of the excitation states. In that case, a resonance can be created, and a peak occurs in the cross-section. The allowable state density in this energy region is much lower for the light nucleus, and the “distance” between states is higher. For heavy nuclei, such as 238U, we can observe a large resonance region in the neutron absorption cross-section.
The compound states (resonances) are observed at low excitation energies. This is due to the fact, the energy gap between the states is large. At high excitation energy, the gap between two compound states is very small, and the widths of resonances may reach the order of the distances between resonances. Therefore, no resonances can be observed at high energies, and the cross-section in this energy region is continuous and smooth.
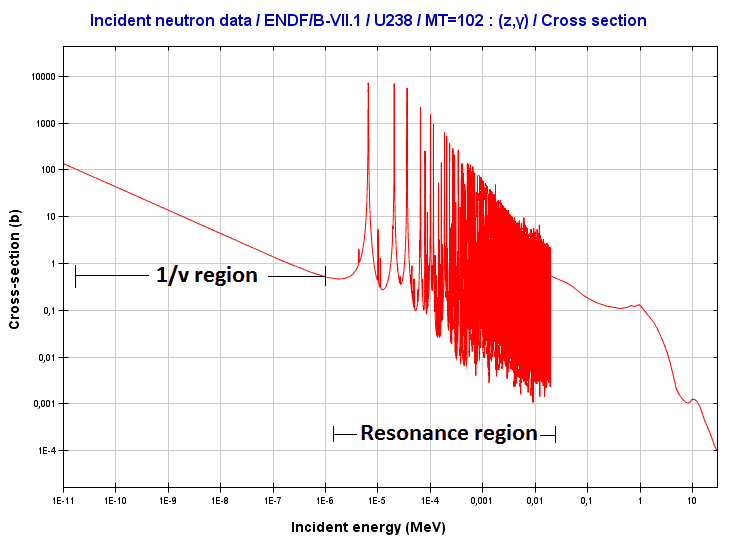
Source: JANIS (Java-based Nuclear Data Information Software); The ENDF/B-VII.1 Nuclear Data Library
The ENDF/B-VII.1 Nuclear Data Library
Types of neutron-nuclear reactions
- Radiative Capture. Most absorption reactions result in the loss of a neutron coupled with the production of one or more gamma rays. This is referred to as a capture reaction, and it is denoted by σγ.
- Neutron-induced Fission Reaction. Some nuclei (fissionable nuclei) may undergo a fission event, leading to two or more fission fragments (nuclei of intermediate atomic weight) and a few neutrons. In a fissionable material, the neutron may be captured, or it may cause nuclear fission. For fissionable materials, we thus divide the absorption cross-section as σa = σγ + σf.
The radiative capture is a reaction in which the incident neutron is completely absorbed, and the compound nucleus is formed. The compound nucleus then decays to its ground state by gamma emission. This process can occur at all incident neutron energies, but the probability of the interaction strongly depends on the incident neutron energy and the target energy (temperature). The energy in the center-of-mass system determines this probability.
The case of neutron-induced charged particle reactions is not so common. Still, some neutron-induced charged particle reactions are important in reactivity control and the detection of neutrons.
Quiz-summary
0 of 20 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
Information
|
You must specify a text. |
Test your Knowledge – Nuclear Reactions
With our simple quizzes, you can test your knowledge.
It is intuitive: start quiz and answer questions.
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
0 of 20 questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 points, (0)
| Average score |
|
| Your score |
|
Categories
- Not categorized 0%
- Nuclear Reactions 0%
-
Here, you can find answers on all the questions:
Share your results on Social Media.
| Pos. | Name | Entered on | Points | Result |
|---|---|---|---|---|
| Table is loading | ||||
| No data available | ||||
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- Answered
- Review
-
Question 1 of 20
1. Question
These nuclear reactions are fast and involve a single-nucleon interaction. The interaction time must be very short (~10-22 s). Incident particles interact on the surface of a target nucleus rather than in the volume of a target nucleus.
This is typical for:
-
Question 2 of 20
2. Question
A projectile and a target nucleus are within the range of nuclear forces for the time allowing for a large number of interactions between nucleons.
This is typical for:
-
Question 3 of 20
3. Question
Products of these reactions are distributed near isotropically in angle (the nucleus loses memory of how it was created – the Bohr’s hypothesis of independence). The mode of decay of the nucleus do not depend on the way the nucleus is formed.
This is typical for:
-
Question 4 of 20
4. Question
Typical direct nuclear reaction is:
-
Question 5 of 20
5. Question
Typical compound nucleus reaction is:
-
Question 6 of 20
6. Question
One the following statements is not typical for nuclear resonances?
Correct
See: Compound Nucleus Reactions
Explanation: The observation of resonances is by no means restricted to neutron-nuclear reactions.
Incorrect
See: Compound Nucleus Reactions
Explanation: The observation of resonances is by no means restricted to neutron-nuclear reactions.
-
Question 7 of 20
7. Question
Raising the temperature of PWR fuel causes the fuel nuclei to vibrate more rapidly within their lattice structures. This causes an increase in resonance absorption of neutrons.
Correct
See: Neutron Absorption
This phenomenon is known as the Doppler broadening of resonances, which is very important phenomenon, that improves reactor stability.
Incorrect
See: Neutron Absorption
This phenomenon is known as the Doppler broadening of resonances, which is very important phenomenon, that improves reactor stability.
-
Question 8 of 20
8. Question
The reaction 40Ca (α, α’)40mCa belongs to:
-
Question 9 of 20
9. Question
One of the following reactions is not a mechanism of neutron elastic scattering reactions:
Correct
See: Elastic Scattering Reactions
The neutron elastic scattering can occur by way of two interaction mechanisms:
- Potential scattering. In potential scattering, the neutron and the nucleus interact without neutron absorption and the formation of a compound nucleus. In fact, the incident neutron does not necessarily have to “touch” the nucleus and the neutron is scattered by the short range nuclear forces when it approaches close enough to the nucleus. Potential scattering occurs with incident neutrons that have an energy of up to about 1 MeV. It may be modeled as a billiard ball collision between a neutron and a nucleus.
- Compound-elastic scattering. In some cases, if the kinetic energy of an incident neutron just right to form a resonance, the neutron may be absorbed and a compound nucleus may be formed. This interaction is more unusual and is also known as resonance elastic scattering. Due to formation of the compound nucleus, initial and final neutron are not the same.
Incorrect
See: Elastic Scattering Reactions
The neutron elastic scattering can occur by way of two interaction mechanisms:
- Potential scattering. In potential scattering, the neutron and the nucleus interact without neutron absorption and the formation of a compound nucleus. In fact, the incident neutron does not necessarily have to “touch” the nucleus and the neutron is scattered by the short range nuclear forces when it approaches close enough to the nucleus. Potential scattering occurs with incident neutrons that have an energy of up to about 1 MeV. It may be modeled as a billiard ball collision between a neutron and a nucleus.
- Compound-elastic scattering. In some cases, if the kinetic energy of an incident neutron just right to form a resonance, the neutron may be absorbed and a compound nucleus may be formed. This interaction is more unusual and is also known as resonance elastic scattering. Due to formation of the compound nucleus, initial and final neutron are not the same.
-
Question 10 of 20
10. Question
Elastic scattering (cross-section) of slow neutrons by molecules is greater than by free nuclei.
Correct
See: Elastic Scattering Reactions
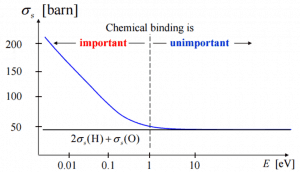
If the kinetic energy of an incident neutron is large compared with the chemical binding energy of the atoms in a molecule, the chemical bound can be ignored.
If the kinetic energy of an incident neutron is of the order or less than the chemical binding energy, the cross-section of the molecule is not equal to the sum of cross-sections of its individual nuclei.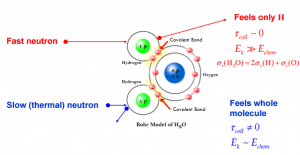
Scattering of slow neutrons by molecules is greater than by free nuclei. Incorrect
See: Elastic Scattering Reactions

If the kinetic energy of an incident neutron is large compared with the chemical binding energy of the atoms in a molecule, the chemical bound can be ignored.
If the kinetic energy of an incident neutron is of the order or less than the chemical binding energy, the cross-section of the molecule is not equal to the sum of cross-sections of its individual nuclei.
Scattering of slow neutrons by molecules is greater than by free nuclei. -
Question 11 of 20
11. Question
During an inelastic scattering the neutron can be absorbed and then re-emitted. This reaction belongs to direct reactions.
Incorrect
See: Inelastic Scattering Reactions
Explanation: The reaction occurs via compound nucleus.
-
Question 12 of 20
12. Question
One the following statements is not typical for neutron inelastic reactions?
Correct
See: Inelastic Scattering Reactions
Explanation: For hydrogen nucleus, inelastic scattering does not occur, because it does not have excited states.
Incorrect
See: Inelastic Scattering Reactions
Explanation: For hydrogen nucleus, inelastic scattering does not occur, because it does not have excited states.
-
Question 13 of 20
13. Question
The most important neutron absorption reactions are divided by the exit channel into two types of reactions. One the following reactions is not an absorption reaction.
-
Question 14 of 20
14. Question
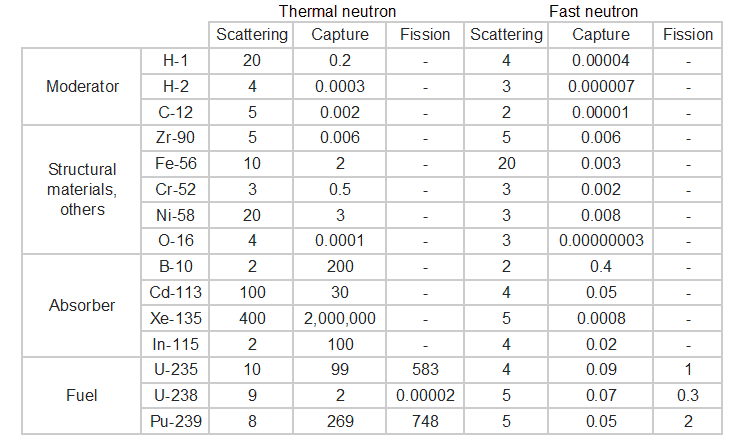
Sort the following isotopes according to the radiative capture cross-section for thermal neutrons from highest to lowest cross-section.
-
Xe-135
-
Gd-157
-
U-235
-
U-238
-
H-1 (Hydrogen)
-
H-2 (Deuterium)
-
-
Question 15 of 20
15. Question
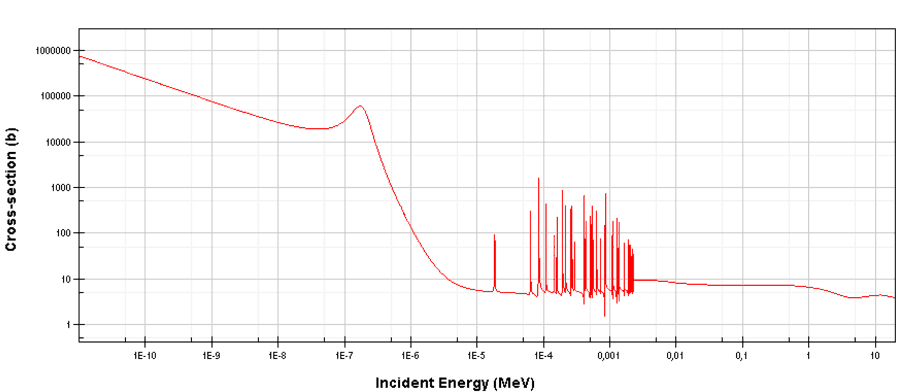
For which neutron absorber is the following chart (cross-sections) typical (see the cut-off energy ~0.5 eV)?
Correct
See: Cadmium
There is a cadmium cut-off energy (Cadmium edge) in the absorption cross-section. Only neutrons of kinetic energy below the cadmium cut-off energy (~0.5 eV) are strongly absorbed by 113Cd. Therefore cadmium is widely used to absorb thermal neutrons in a thermal neutron filters.
Incorrect
See: Cadmium
There is a cadmium cut-off energy (Cadmium edge) in the absorption cross-section. Only neutrons of kinetic energy below the cadmium cut-off energy (~0.5 eV) are strongly absorbed by 113Cd. Therefore cadmium is widely used to absorb thermal neutrons in a thermal neutron filters.
-
Question 16 of 20
16. Question
What is not typical for (γ, n) reactions (production of photoneutrons)?
Correct
See: Photoneutrons
Explanation: Photoneutrons are usually major source in a reactor and ensure sufficient neutron flux on source range detectors when reactor is subcritical in long term shutdown.
Incorrect
See: Photoneutrons
Explanation: Photoneutrons are usually major source in a reactor and ensure sufficient neutron flux on source range detectors when reactor is subcritical in long term shutdown.
-
Question 17 of 20
17. Question
The most important neutron nuclear reactions in nuclear reactor physics are reactions of thermal neutrons with boron nuclei (rather with 10B nuclei). In nuclear industry boron is commonly used as a neutron absorber and a neutron converter.
Which type of reaction is used in neutron absorbers based on boron (n + 10B)?
Correct
See: Boron 10

This equation describes neutron capture in the boron, which is diluted in the coolant. Boric acid is used in nuclear power plants as a long-term compensator of nuclear fuel reactivity. Incorrect
See: Boron 10

This equation describes neutron capture in the boron, which is diluted in the coolant. Boric acid is used in nuclear power plants as a long-term compensator of nuclear fuel reactivity. -
Question 18 of 20
18. Question
Which of the following charged particle reactions is very important in reactivity control?
Correct
See: Boron 10
See: Charged Particle Reactions
Explanation: Most of (n,alpha) reactions of thermal neutrons are 10B(n,alpha)7Li reactions accompanied by 0.48 MeV gamma emission. Therefore boron can be used as neutron absorber in case chemical shim, burnable absorbers or control rods. It can be also used as the neutron converter in neutron detectors, because its cross-section is very high and produces energetic alpha particles.
Incorrect
See: Boron 10
See: Charged Particle Reactions
Explanation: Most of (n,alpha) reactions of thermal neutrons are 10B(n,alpha)7Li reactions accompanied by 0.48 MeV gamma emission. Therefore boron can be used as neutron absorber in case chemical shim, burnable absorbers or control rods. It can be also used as the neutron converter in neutron detectors, because its cross-section is very high and produces energetic alpha particles.
-
Question 19 of 20
19. Question
Which of the following charged particle reactions is very important in radiation protection?
Correct
See: Charged Particle Reactions
Explanation: This nuclear reaction continually take place especially in the earth’s atmosphere, forming equilibrium amounts of the radionuclide 14C. In nuclear power plants, it is important especially from radiation protection point of view. The reaction is responsible for most of the radiation dose delivered to the human body by thermal neutrons. The nitrogen atoms are contained in proteins, therefore if the human body is exposed to thermal neutrons, then these thermal neutrons may be absorbed by 14N, causing a proton emission. Protons are directly ionizing particles and deposit their energy over a very short distance in the body tissue.
Incorrect
See: Charged Particle Reactions
Explanation: This nuclear reaction continually take place especially in the earth’s atmosphere, forming equilibrium amounts of the radionuclide 14C. In nuclear power plants, it is important especially from radiation protection point of view. The reaction is responsible for most of the radiation dose delivered to the human body by thermal neutrons. The nitrogen atoms are contained in proteins, therefore if the human body is exposed to thermal neutrons, then these thermal neutrons may be absorbed by 14N, causing a proton emission. Protons are directly ionizing particles and deposit their energy over a very short distance in the body tissue.
-
Question 20 of 20
20. Question
Q-value of the reaction is:
Correct
See: Q-value
The Q-value of the reaction is given by:
Q = [ma + mA – (mb + mB)]c2
which is the same as the excess kinetic energy of the final products:
Q = Tfinal – Tinitial
= Tb + TB – (Ta + TA)
For reactions in which there is an increase in the kinetic energy of the products Q is positive. The positive Q reactions are said to be exothermic (or exergic). There is a net release of energy, since the kinetic energy of the final state is greater than the kinetic energy of the initial state.
For reactions in which there is a decrease in the kinetic energy of the products Q is negative. The negative Q reactions are said to be endothermic (or endoergic) and they require a net energy input.
Incorrect
See: Q-value
The Q-value of the reaction is given by:
Q = [ma + mA – (mb + mB)]c2
which is the same as the excess kinetic energy of the final products:
Q = Tfinal – Tinitial
= Tb + TB – (Ta + TA)
For reactions in which there is an increase in the kinetic energy of the products Q is positive. The positive Q reactions are said to be exothermic (or exergic). There is a net release of energy, since the kinetic energy of the final state is greater than the kinetic energy of the initial state.
For reactions in which there is a decrease in the kinetic energy of the products Q is negative. The negative Q reactions are said to be endothermic (or endoergic) and they require a net energy input.
- J. R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).
- J. R. Lamarsh, A. J. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 0-201-82498-1.
- W. M. Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1.
- Glasstone, Sesonske. Nuclear Reactor Engineering: Reactor Systems Engineering, Springer; 4th edition, 1994, ISBN: 978-0412985317
- W.S.C. Williams. Nuclear and Particle Physics. Clarendon Press; 1 edition, 1991, ISBN: 978-0198520467
- G.R.Keepin. Physics of Nuclear Kinetics. Addison-Wesley Pub. Co; 1st edition, 1965
Advanced Reactor Physics:
- K. O. Ott, W. A. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 0-894-48033-2.
- K. O. Ott, R. J. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 0-894-48029-4.
- D. L. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 0-894-48453-2.
- E. E. Lewis, W. F. Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 0-894-48452-4.