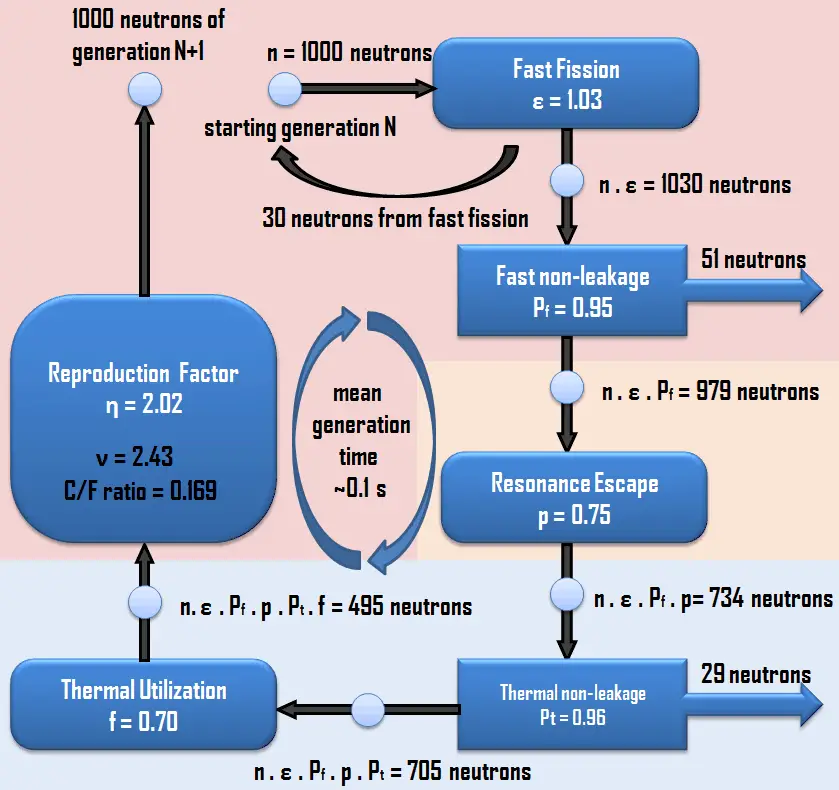
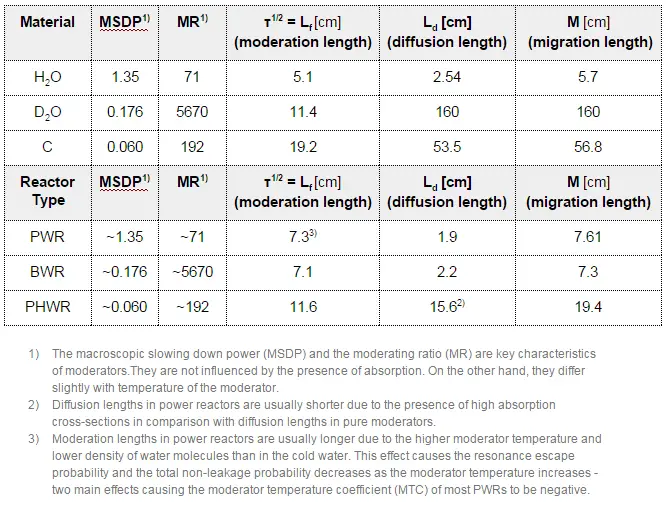
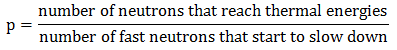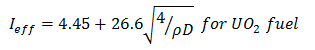The fast fission factor determined the number of fast neutrons produced by fissions at all energies. Still, in thermal reactors, the bulk of fissions occurs at thermal energies, and it is obvious these fission neutrons have to be thermalized. But during this thermalization, neutrons may collide not only with moderator nuclei but also with fuel nuclei. Unfortunately, especially 238U exhibits resonance behavior between the fast region and the thermal region. While the neutrons are slowing down through the resonance region of 238U, which extends from about 6 eV to 200 eV, there is a chance that some neutrons will be captured. The probability that a neutron will not be captured in the entire resonance region is called the resonance escape probability.
See also: Nuclear Resonance
979
↓
p ~ 0.75
↓
734
The resonance escape probability, symbolized by p, is the probability that a neutron will be slowed to thermal energy and escape resonance capture. This probability is defined as the ratio of the number of neutrons that reach thermal energies to the number of fast neutrons that slow down.
The compound nucleus is the intermediate state formed in a compound nucleus reaction. It is normally one of the excited states of the nucleus formed by the combination of the incident particle and target nucleus. Suppose a target nucleus X is bombarded with particles a. In that case, it is sometimes observed that the ensuing nuclear reaction occurs with appreciable probability only if the energy of particle a is in the neighborhood of certain definite energy values. These energy values are referred to as resonance energies. The compound nuclei of these certain energies are referred to as nuclear resonances. Resonances are usually found only at relatively low energies of the projectile. The widths of the resonances increase in general with increasing energies. At higher energies, the widths may reach the order of the distances between resonances, and then no resonances can be observed. The narrowest resonances are usually the compound states of heavy nuclei (such as fissionable nuclei) and thermal neutrons (usually in (n,γ) capture reactions). The observation of resonances is by no means restricted to neutron nuclear reactions.
See also: Compound Nucleus Reactions
From the definition, p is always less than 1.0 when there is any amount of 238U or 240Pu present in the core, which means that resonance capture by these isotopes always removes some of the neutrons from the neutron flux.
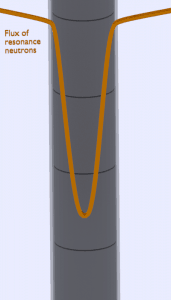
The resonance escape probability is strongly influenced by the arrangement and the geometry of the reactor core. There is a significantly higher probability that the resonance neutron collides with the fuel nucleus in a homogenous reactor core, where many moderator nuclei surround fuel nuclei. Therefore in homogenous reactor cores, the resonance escape probability is lower than in heterogeneous cores.
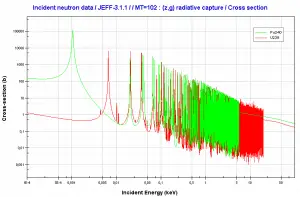
On the other hand, all fuel nuclei in a heterogeneous reactor core are in fuel pellets encapsulated within a fuel rod. This arrangement increases the probability that the fast neutron will escape the fuel matrix. The neutron slows down in the moderator where there are no atoms of 238U and 240Pu present. Therefore in heterogeneous reactor cores, the resonance escape probability is significantly higher than in homogenous cores.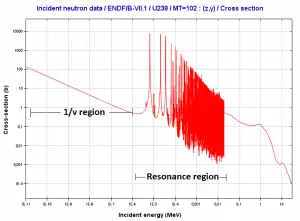
ξCARBON = 0.158N(2MeV → 1eV) = ln 2⋅106/ξ =14.5/0.158 = 92
Theory of Resonance Escape Probability
The resonance escape probability is for heterogeneous reactor cores about 0.75. But more than its value, how this value can be changed during reactor operation is crucial. The resonance capture is the main phenomenon, which contributes to the reactor stability and makes the reactor core inherently safe and resistant to prompt power changes, as in the case of reactivity-initiated accidents (RIA). This feature depends strongly on certain reactor designs and also on certain fuel loading patterns. Therefore it must be verified during the reload safety assessment.
The resonance escape probability for all resonances can be calculated according to the following equation:
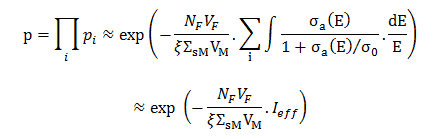
The integral in this expression is called the effective resonance integral Ιeff. In practical situations, this integral strongly depends on the geometry of the unit cell. The core’s geometry strongly influences the spatial and energy self-shielding primarily in heterogeneous reactor cores. This phenomenon causes a significant increase in the resonance escape probability (“p” from the four-factor formula) compared to homogeneous cores. Without the spatial self-shielding provided by the separation of fuel and moderator, values of keff = 1 are possible with natural uranium fuel only if heavy water is used as the moderator. In the literature, empirical relations have been developed for the effective resonance integral of the following form:
where the resonance integrals are in barns, rho is the material density in g/cm3, and D is the fuel rod diameter in cm. This equation is valid for isolated rods with a diameter higher than 0.2cm.
For tightly packed lattice in the fuel, assembly self-shielding increases somewhat through a Dancoff correction.
From the equation, it is obvious. The resonance escape probability is also dependent on the moderator-to-fuel ratio – NM / NF (the term before Ieff). The change of the moderator-to-fuel ratio also changes the neutron flux spectrum in the reactor core. Most light water reactors are designed as so-called under moderated, and the neutron flux spectrum is slightly harder (the moderation is slightly insufficient) than in an optimum case. But this design provides an important safety feature. This feature will be discussed in the following section.
Main operational changes that affect this factor:
- Change in the moderator temperature. It is known, the resonance escape probability is also dependent on the moderator-to-fuel ratio. As the moderator temperature increases, the ratio of the moderating atoms (molecules of water) decreases due to the thermal expansion of water. Its density simply decreases. This, in turn, causes hardening of neutron spectrum in the reactor core resulting in higher resonance absorption (lower p). The decreasing density of the moderator causes that neutrons stay at a higher energy for a longer period, which increases the probability of non-fission capture of these neutrons. This process is one of two processes, which determine the moderator temperature coefficient (MTC). The second process is connected with the leakage probability of the neutrons. The moderator temperature coefficient must be for most PWRs negative, which improves the reactor stability because reactor core heating causes a negative reactivity insertion.
- Change in the fuel temperature. The second operational change, which affects the resonance escape probability, is connected with the phenomenon usually known as the Doppler broadening. The effect of the Doppler broadening is generally considered to be even more important than a negative moderator temperature coefficient. Especially in the case of reactivity-initiated accidents (RIA), the Doppler coefficient of reactivity would be the first and most important effect in the compensation of the inserted positive reactivity. The time for heat to be transferred to the moderator is usually measured in seconds, while the Doppler coefficient is effective almost instantaneously. The Doppler broadening with the self-shielding process causes the Doppler coefficient (or the fuel temperature coefficient) for all power reactors to always be negative. Therefore an increase in the fuel temperature promptly causes an increase in the resonance integral (Ieff), which, in turn, causes a negative reactivity insertion. It is of the highest importance in reactor safety.
See also: Doppler Broadening
See also: Self-shielding
