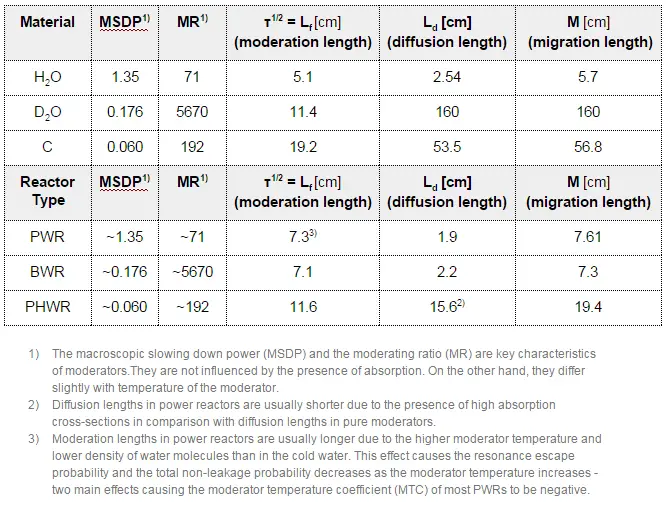
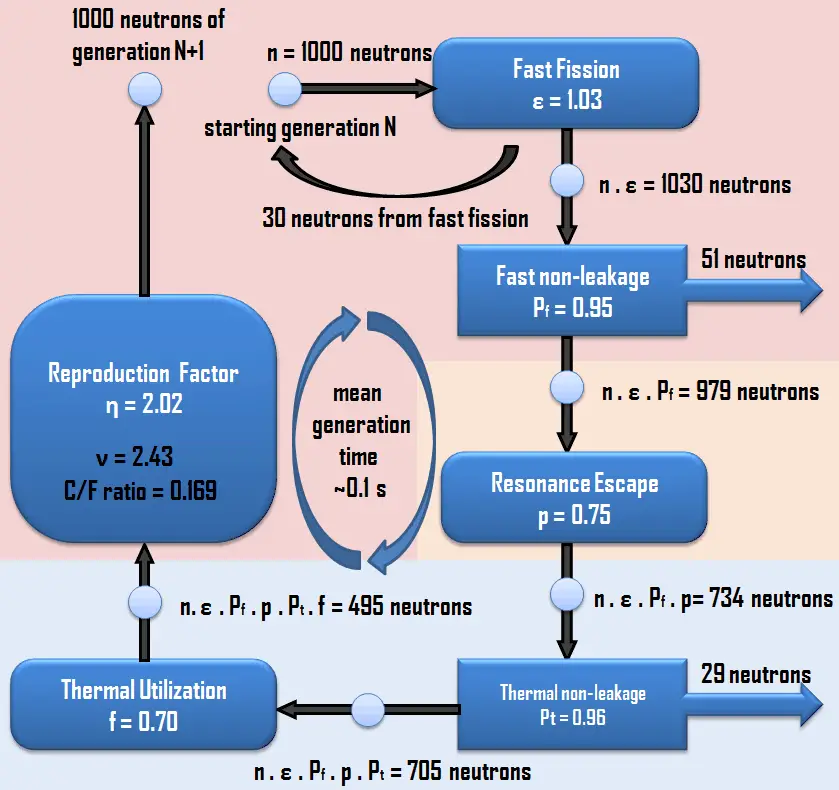
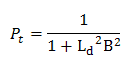In an infinite multiplication system, the neutron leakage of the system is logically neglected. But all multiplying systems, all realistic reactor cores are finite multiplying systems, and this process may not be neglected. During neutron diffusion, some of the neutrons leak out of the boundaries of the reactor core before they are absorbed. This process and its impact on the effective multiplying factor is characterized by the thermal non-leakage factor, Pt, defined as the ratio of the number of thermal neutrons that do not leak from the reactor core during the neutron diffusion process to the number of neutrons that reach thermal energies.
734
↓
Pt ~ 0.96
↓
705
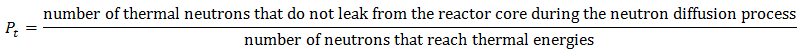
The thermal non-leakage probability is for large reactor cores about 0.95 – 0.98. This value is minimally affected (in comparison with the other factors) by operational changes except for changes in the moderator temperature. The only parameter that influences the thermal non-leakage probability is the moderator temperature. It can be derived from the neutron diffusion theory. The following equation also represents the probability that a thermal neutron will remain in the core:
Ld is the diffusion length, B is the geometrical buckling (in case of critical state Bg = Bm), which depends only on the shape and size of the core. The value of B for small cores is higher than the value for large cores. So that, it is obvious, the thermal neutrons leakage is higher for small cores and depends on the macroscopic slowing down power of neutron moderator (leakage is higher for poor moderators). The following equation gives the diffusion length:
Total Non-leakage Probability
The fast non-leakage probability (Pf) and the thermal non-leakage probability (Pt) may be combined into one term that gives the fraction of all neutrons that do not leak out of the reactor core. This term is called the total non-leakage probability and is given the symbol PNL, and may be expressed by the following equation: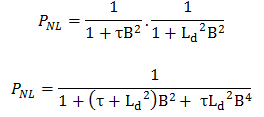
For large reactors, we can rewrite this equation without a substantial loss of accuracy simply by replacing the diffusion length Ld and τ with the migration length M in the one group equation. The term B4 is very small for large reactors, and therefore it can be neglected. We may then write.
where M is the migration area (m2). The migration length is defined as the square root of the migration area.
Main operational changes that affect this factor:
Since both (Pf and Pt) are affected by a change in moderator temperature in a heterogeneous water-moderated reactor and the directions of the feedbacks are the same, the resulting total non-leakage probability is also sensitive to the change in the moderator temperature. As a result, an increase in the moderator temperature causes that the probability of leakage to increase. This effect is one of two main effects causing the moderator temperature coefficient (MTC) of most PWRs to be negative.
The thermal neutron leakage is dependent on the core temperature (or moderator temperature). The moderator temperature influences macroscopic cross-sections for elastic scattering reaction, especially the atomic number density – NH2O(Σs=σs.NH2O) due to the thermal expansion of water. Also, the microscopic cross-section (σa) for neutron absorption changes with core temperature. Both processes have the same direction. As the temperature of the core increases, the diffusion coefficient (D = 1/3.Σtr) increases, and the absorption cross-section decreases, which causes the increase in the thermal neutron leakage. This physical process is a part of the moderator temperature coefficient (MTC).
In power reactors, the total non-leakage probability also significantly changes with fuel burnup. This dependency is not associated with any of the parameters like the diffusion coefficient or the geometrical buckling. In power reactors, the total non-leakage probability depends on the certain fuel loading pattern, and the reload strategy. Neutron leakage is one of the key parameters in the neutron and fuel economy.
To enhance the neutron and fuel economy, core designers design the low leakage loading patterns, in which fresh fuel assemblies are not situated in the peripheral positions of the reactor core. The peripheral positions are loaded with the fuel with the highest fuel burnup. Compared to the average assemblies, these “high” burnup assemblies have inherently lower relative power (due to the lower kinf and the fact they feel the presence of a non-multiplying environment). In short, this parameter is significantly dependent on a certain loading pattern. During fuel burnup, the neutron leakage usually increases, especially in low leakage loading patterns. This process is caused by reducing the differences in kinf between fresh fuel assemblies and peripheral high-burnup assemblies.