Atoms
What are atoms?
The atoms are defined as the smallest constituents of ordinary matter, which can be divided without releasing electrically charged particles. The atoms consist of two parts, an atomic nucleus, and an electron cloud.
Key Facts
- The physical world is composed of combinations of various subatomic or fundamental particles, and these are the smallest building blocks of matter.
- The atoms consist of two parts, an atomic nucleus, and an electron cloud.
- The number of electrons and their arrangement in the electron cloud is responsible for the chemical behavior of atoms.
- The nuclear properties (atomic mass, nuclear cross-sections) of the element are determined by the number of protons (atomic number) and the number of neutrons (neutron number).
- Nuclear stability is a concept that helps to identify the stability of an isotope. It is needed to find the ratio of neutrons to protons to identify the stability of an isotope. To determine the stability of an isotope, you can use the ratio neutron/proton (N/Z).
- There are only certain combinations of neutrons and protons which form stable nuclei.
- Unstable nuclei must undergo nuclear decay (radioactive decay) to stabilize themselves, and it is a random and natural process.
- The number of atoms in 1 mole (e.g., 12 grams of carbon) of a substance is equal to the Avogadro’s constant, equal to 6.022 x 1023.
The physical world is composed of combinations of various subatomic or fundamental particles. These are the smallest building blocks of matter. All matter except dark matter is made of molecules, which are themselves made of atoms. The atoms are the smallest constituents of ordinary matter, which can be divided without releasing electrically charged particles. The atoms consist of two parts. An atomic nucleus and an electron cloud. The electrons are spinning around the atomic nucleus. The nucleus itself is generally made of protons and neutrons, but even these are composite objects. Inside the protons and neutrons, we find the quarks.
 The science that deals with atoms are called atomic physics. Note that atomic and nuclear physics are not the same. The term atomic physics is often associated with nuclear power due to the synonymous use of atomic and nuclear in standard English. However, physicists distinguish between atomic and nuclear physics. Atomic physics deals with the atom as a system consisting of a nucleus and electrons. Nuclear physics deals with the nucleus as a system consisting of nucleons (protons and neutrons). The main difference is in the scale. While the term atomic deals with 1Å = 10-10m, where Å is an ångström (according to Anders Jonas Ångström), the term nuclear deals with 1femtometre = 1fermi = 10-15m.
The science that deals with atoms are called atomic physics. Note that atomic and nuclear physics are not the same. The term atomic physics is often associated with nuclear power due to the synonymous use of atomic and nuclear in standard English. However, physicists distinguish between atomic and nuclear physics. Atomic physics deals with the atom as a system consisting of a nucleus and electrons. Nuclear physics deals with the nucleus as a system consisting of nucleons (protons and neutrons). The main difference is in the scale. While the term atomic deals with 1Å = 10-10m, where Å is an ångström (according to Anders Jonas Ångström), the term nuclear deals with 1femtometre = 1fermi = 10-15m.
Atomic physics is the field of physics that studies atoms as an isolated system of electrons and an atomic nucleus. It is primarily concerned with the arrangement of electrons around the nucleus and the processes by which these arrangements change. This includes ions as well as neutral atoms, and unless otherwise stated, for the purposes of this discussion, it should be assumed that the term atom includes ions. Atomic physics also helps to understand the physics of molecules, but molecular physics also describes the physical properties of molecules.
See also: Atomic and Nuclear Physics
Properties of Atoms
Every solid, liquid, gas, and plasma is composed of neutral or ionized atoms. The chemical properties of the atom are determined by the number of protons, in fact, by the number and arrangement of electrons. The configuration of these electrons follows the principles of quantum mechanics. The number of electrons in each element’s electron shells, particularly the outermost valence shell, is the primary factor determining its chemical bonding behavior. In the periodic table, the elements are listed in order of increasing atomic number Z.
Chemical Properties of Atoms
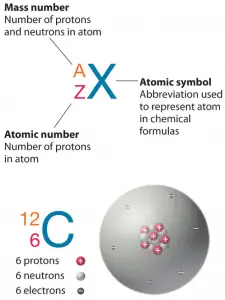 The total number of protons in the nucleus of an atom is called the atomic number (or the proton number) of the atom and is given the symbol Z. The number of electrons in an electrically-neutral atom is the same as the number of protons in the nucleus. Therefore, the total electrical charge of the nucleus is +Ze, where e (elementary charge) equals 1,602 x 10-19coulombs. Each electron is influenced by the electric fields produced by the positive nuclear charge and the other (Z – 1) negative electrons in the atom.
The total number of protons in the nucleus of an atom is called the atomic number (or the proton number) of the atom and is given the symbol Z. The number of electrons in an electrically-neutral atom is the same as the number of protons in the nucleus. Therefore, the total electrical charge of the nucleus is +Ze, where e (elementary charge) equals 1,602 x 10-19coulombs. Each electron is influenced by the electric fields produced by the positive nuclear charge and the other (Z – 1) negative electrons in the atom.
It is the Pauli exclusion principle that requires the electrons in an atom to occupy different energy levels instead of them all condensing in the ground state. The ordering of the electrons in the ground state of multielectron atoms starts with the lowest energy state (ground state). It moves progressively from there up the energy scale until each of the atom’s electrons has been assigned a unique set of quantum numbers. This fact has key implications for building up the periodic table of elements.
Nuclear Properties
Properties of atomic nuclei (atomic mass, nuclear cross-sections) are determined by the number of protons and the number of neutrons (neutron number). It must be noted especially nuclear cross-sections may vary by many orders from nuclide with the neutron number N to nuclide with the neutron number N+1. For example, actinides with odd neutron numbers are usually fissile (fissionable with slow neutrons), while actinides with even neutron numbers are not fissile (but fissionable with fast neutrons). Due to the Pauli exclusion principle, heavy nuclei with an even number of protons and an even number of neutrons are very stable thanks to the occurrence of ‘paired spin.’ On the other hand, nuclei with an odd number of protons and neutrons are mostly unstable.
Neutron and Atomic Numbers and Nuclear Stability
Nuclear stability is a concept that helps to identify the stability of an isotope. It is needed to find the ratio of neutrons to protons to identify the stability of an isotope. To determine the stability of an isotope, you can use the ratio neutron/proton (N/Z). Also, to help understand this concept, there is a chart of the nuclides, known as a Segre chart. This chart shows a plot of the known nuclides as a function of their atomic and neutron numbers. It can be observed from the chart that there are more neutrons than protons in nuclides with Z greater than about 20 (Calcium). These extra neutrons are necessary for the stability of the heavier nuclei. The excess neutrons act somewhat like nuclear glue. Only two stable nuclides have fewer neutrons than protons: hydrogen-1 and helium-3.

Atomic nuclei consist of protons and neutrons, which attract each other through the nuclear force, while protons repel each other via the electric force due to their positive charge. These two forces compete, leading to various stability of nuclei. There are only certain combinations of neutrons and protons which form stable nuclei.
Neutrons stabilize the nucleus because they attract each other and protons, which helps offset the electrical repulsion between protons. As a result, as the number of protons increases, an increasing ratio of neutrons to protons is needed to form a stable nucleus. If there are too many or too few neutrons for a given number of protons, the resulting nucleus is not stable and undergoes radioactive decay. Unstable isotopes decay through various radioactive decay pathways, most commonly alpha decay, beta decay, gamma decay, or electron capture. Many other rare types of decay, such as spontaneous fission or neutron emission, are known.
Mass of Atom
See also: Atomic Masses
Since electrons are almost massless (in comparison to the nucleons), the total number of protons and neutrons in the nucleus of an atom determines the atomic mass. The total number of protons and neutrons in the nucleus of an atom is called the atomic mass number (or the mass number) of the atom and is given the symbol A. The mass number is different for each different isotope of a chemical element. The mass number is written either after the element name or as a superscript to the left of an element’s symbol. For example, the most common isotope of carbon is carbon-12 or 12C.
The unit of measure for mass is the atomic mass unit (amu). One atomic mass unit is equal to 1.66 x 10-24 grams.
Besides the standard kilogram, it is a second mass standard. It is the carbon-12 atom, which, by international agreement, has been assigned a mass of 12 atomic mass units (u). The relation between the two units is
One atomic mass unit is equal:
1u = 1.66 x 10-24 grams.
One unified atomic mass unit is approximately the mass of one nucleon (either a single proton or neutron) and is numerically equivalent to 1 g/mol.
For 12C, the atomic mass is exactly 12u since the atomic mass unit is defined from it. The isotopic mass usually differs for other isotopes and is usually within 0.1 u of the mass number. For example, 63Cu (29 protons and 34 neutrons) has a mass number of 63, and an isotopic mass in its nuclear ground state is 62.91367 u.
There are two reasons for the difference between mass number and isotopic mass, known as the mass defect:
- The neutron is slightly heavier than the proton, increasing the mass of nuclei with more neutrons than protons relative to the atomic mass unit scale based on 12C with equal numbers of protons and neutrons.
- The nuclear binding energy varies between nuclei, and a nucleus with greater binding energy has lower total energy and a lower mass according to Einstein’s mass-energy equivalence relation E = mc2. For 63Cu, the atomic mass is less than 63, so this must be the dominant factor.
Ionization Energy of Atoms
Ionization energy, also called ionization potential, is the energy necessary to remove an electron from the neutral atom.
X + energy → X+ + e−
where X is any atom or molecule capable of ionizing, X+ is that atom or molecule with an electron removed (positive ion), and e− is the removed electron.
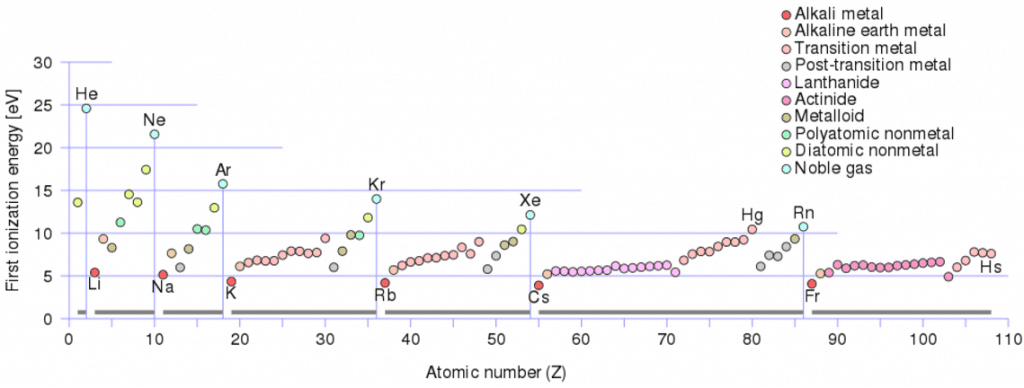
There is ionization energy for each successive electron removed. The electrons that circle the nucleus move in fairly well-defined orbits, and some of these electrons are more tightly bound in the atom than others. For example, only 7.38 eV is required to remove the outermost electron from a lead atom, while 88,000 eV is required to remove the innermost electron.
- Ionization energy is lowest for the alkali metals, which have a single electron outside a closed shell.
- Ionization energy increases across a row on the periodic maximum for the noble gases which have closed shells.
For example, sodium requires only 496 kJ/mol or 5.14 eV/atom to ionize it. On the other hand, neon, the noble gas immediately preceding it in the periodic table, requires 2081 kJ/mol or 21.56 eV/atom.
The ionization energy associated with removing the first electron is most commonly used. The nth ionization energy refers to the amount of energy required to remove an electron from the species with a charge of (n-1).
1st ionization energy
X → X+ + e−
2nd ionization energy
X+ → X2+ + e−
3rd ionization energy
X2+ → X3+ + e−
For example, only 7.38 eV is required to remove the outermost electron from a lead atom, while 88,000 eV is required to remove the innermost electron.
Atomic Radius of Chemical Elements
It must be noted that atoms lack a well-defined outer boundary. The atomic radius of a chemical element measures the distance out to which the electron cloud extends from the nucleus. However, this assumes the atom exhibits a spherical shape, which is only obeyed for atoms in a vacuum or free space. Therefore, there are various non-equivalent definitions of atomic radius.
- Van der Waals radius. In principle, Vana der Waals radius is half the minimum distance between the nuclei of two atoms of the element that are not bound to the same molecule.
- Ionic radius. An ionic radius is one-half the distance between the nuclei of two ions in an ionic bond.
- Covalent radius. A covalent radius is the nominal radius of the atoms of an element when covalently bound to other atoms.
- Metallic radius. A metallic radius is one-half the distance between the nuclei of two adjacent atoms in a crystalline structure when joined to other atoms by metallic bonds.
See also: Atomic Radii
Volume of Atom
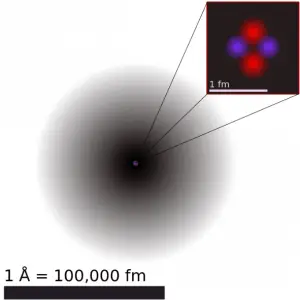
The volume of an atom is about 15 orders of magnitude larger than the volume of a nucleus. For uranium atom, the Van der Waals radius is about 186 pm = 1.86 ×10−10m. The Van der Waals radius, rw, of an atom is the radius of an imaginary hard-sphere representing the distance of the closest approach to another atom. Assuming a spherical shape, the uranium atom has a volume of about 26.9 ×10−30 m3. But this “huge” space is occupied primarily by electrons because the nucleus occupies only about 1721×10−45 m3 of space. Together, these electrons weigh only a fraction (let’s say 0.05%) of the entire atom.
It may seem that the space and the matter are empty, but it is not. Due to the quantum nature of electrons, the electrons are not pointing particles, and they are smeared out over the whole atom. The classical description cannot be used to describe things on the atomic scale. On the atomic scale, physicists have found that quantum mechanics describes things very well on that scale. Particle locations in quantum mechanics are not at an exact position, and they are described by a probability density function. Therefore the space in an atom (between electrons and an atomic nucleus) is not empty. Still, it is filled by a probability density function of electrons (usually known as “electron cloud “).
Antiatom
Antimatter refers to material that would be made up of “antiatoms” in which antiprotons and antineutrons would form the nucleus around which positrons (antielectrons) would move. The term is also used for antiparticles in general.
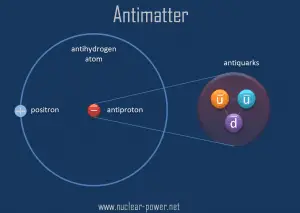 Antimatter particles bind with one another to form antimatter, just as ordinary particles bind to form normal matter. For example, a positron and an antiproton can form an antihydrogen atom. Physical principles indicate that complex antimatter atomic nuclei are possible and anti-atoms corresponding to the known chemical elements.
Antimatter particles bind with one another to form antimatter, just as ordinary particles bind to form normal matter. For example, a positron and an antiproton can form an antihydrogen atom. Physical principles indicate that complex antimatter atomic nuclei are possible and anti-atoms corresponding to the known chemical elements.
Our visible Universe is almost entirely composed of matter, and very little antimatter has existed since the Big Bang. This problem is known as the baryon asymmetry. This asymmetry of matter and antimatter in the visible Universe is one of the great unsolved problems in physics.
In the special theory of relativity, certain types of matter may be created or destroyed. Still, the mass and energy associated with such matter remain unchanged in quantity in all of these processes. As a result of the conservation of lepton and baryon numbers, antimatter (antiparticles) can be created out of energy, but only if a particle counterpart for every antiparticle is created as well.