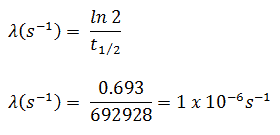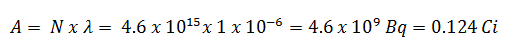Article Summary & FAQs
What is radioactive decay law?
The radioactive decay law states that the probability per unit time that a nucleus will decay is constant, independent of time. This constant is called the decay constant and is denoted by λ, “lambda”. This constant probability may vary greatly between different types of nuclei, leading to the many different observed decay rates. The radioactive decay of a certain number of atoms (mass) is exponential in time.
Key Facts
- There are only certain combinations of neutrons and protons, which form stable nuclei. As the number of protons increases, an increasing ratio of neutrons to protons is needed to form a stable nucleus. If there are too many (neutrons also obey the Pauli exclusion principle) or too few neutrons for a given number of protons, the resulting nucleus is not stable, and it undergoes radioactive decay.
- Radioactive decay is a random process at the level of single atoms, in that, according to quantum theory, it is impossible to predict when a particular atom will decay. In other words, a nucleus of a radionuclide has no “memory”.
- The radioactive decay law is valid for all modes of decay. There are three basic modes of radioactive decay:
Additional important decay modes:
- A measure of radioactivity (activity) is based on the counting of disintegrations per second. The SI unit of activity is the becquerel (Bq), equal to one reciprocal second.
- The radioactive decay of a certain number of atoms (mass) is exponential in time. Radioactive decay law: N = N.e-λt The rate of nuclear decay is also measured in terms of half-lives. Radioactive material with a short half-life is much more radioactive (at the time of production) but will obviously lose its radioactivity rapidly.
- The half-life is the amount of time it takes for a given isotope to lose half of its radioactivity. If a radioisotope has a half-life of 14 days, half of its atoms will have decayed within 14 days. In 14 more days, half of that remaining half will decay, and so on.
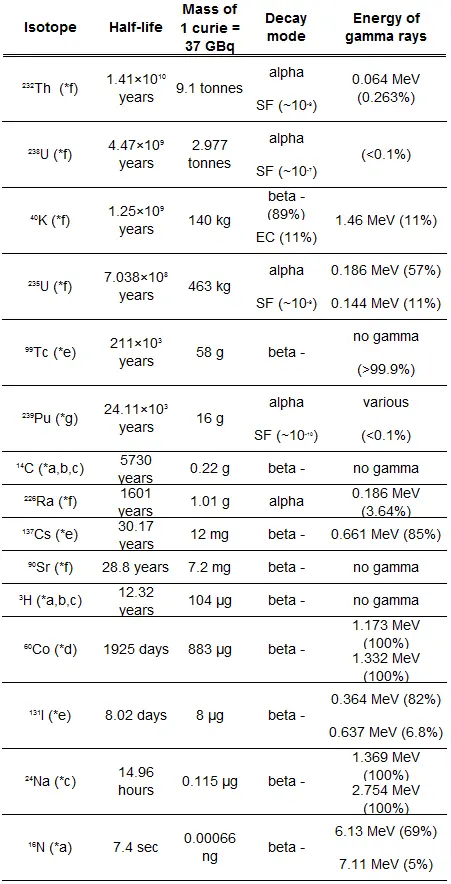
- Half-lives range from millionths of a second for highly radioactive fission products to billions of years for long-lived materials (such as naturally occurring uranium).
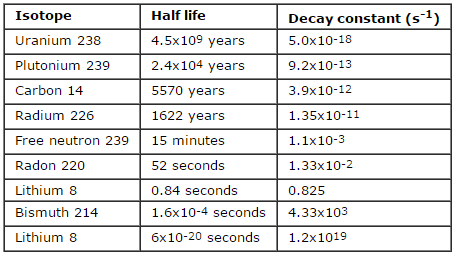
- In radioactivity calculations, one of two parameters (decay constant or half-life), which characterize the rate of decay, must be known. There is a relation between the half-life (t1/2) and the decay constant λ.
Half-lives range from millionths of a second for highly radioactive fission products to billions of years for long-lived materials (such as naturally occurring uranium).
The radioactive decay law is an universal law that describes the statistical behaviour of a large number of nuclides.
As was written, radioactive decay is a random process at the level of single atoms. According to quantum theory, it is impossible to predict when a particular atom will decay. In other words, a nucleus of a radionuclide has no “memory”. A nucleus does not “age” over time. Thus, the probability of its breaking down does not increase with time but stays constant no matter how long the nucleus has existed. During its unpredictable decay, this unstable nucleus spontaneously and randomly decomposes to form a different nucleus (or a different energy state – gamma decay), giving off radiation in the form of atomic particles or high energy rays.
Calculations of the decay of radioactive nuclei are relatively straightforward because there is only one fundamental law governing all decay processes.
The radioactive decay law states that the probability per unit time that a nucleus will decay is constant, independent of time. This constant is called the decay constant and is denoted by λ, “lambda”. This constant probability may vary greatly between different types of nuclei, leading to the many different observed decay rates. The radioactive decay of a certain number of atoms (mass) is exponential in time.
Radioactive decay law: N = N.e-λt
The rate of nuclear decay is also measured in terms of half-lives. The half-life is the amount of time it takes for a given isotope to lose half of its radioactivity. If a radioisotope has a half-life of 14 days, half of its atoms will have decayed within 14 days. In 14 more days, half of that remaining half will decay, and so on. Half-lives range from millionths of a second for highly radioactive fission products to billions of years for long-lived materials (such as naturally occurring uranium). Notice that short half-lives go with large decay constants. Radioactive material with a short half-life is much more radioactive (at the time of production) but will obviously lose its radioactivity rapidly. No matter how long or short, the half-life is after seven half-lives have passed, less than 1 percent of the initial activity remaining.
The radioactive decay law can also be derived for activity calculations or mass of radioactive material calculations:
(Number of nuclei) N = N.e-λt (Activity) A = A.e-λt (Mass) m = m.e-λt
Where N (number of particles) is the total number of particles in the sample, A (total activity) is the number of decays per unit time of a radioactive sample, m is the mass of remaining radioactive material.
Activity – Specific Activity
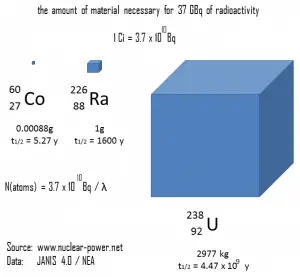A measure of radioactivity (activity) is based on the counting of disintegrations per second. The SI unit of activity is the becquerel (Bq), equal to one reciprocal second. The activity depends only on the number of decays per second, not on the type of decay, the energy of the decay products, or the biological effects of the radiation. It can be used to characterize the rate of emission of ionizing radiation. Specific activity is the activity per quantity of a radionuclide. Thus specific activity is defined as the activity per quantity of atoms of a particular radionuclide. It is usually given in units of Bq/g, but another commonly used unit of activity is the curie (Ci), allowing the definition of specific activity in Ci/g.
Units of activity (the curie and the becquerel) can also be used to characterize an overall quantity of controlled or accidental releases of radioactive atoms.
Units of Activity
- Becquerel. The becquerel is the SI unit of radioactivity defined in 1974. It is named in honor of Henri Becquerel, a French physicist who discovered radioactivity in 1896. One becquerel (1Bq) is equal to 1 disintegration per second.
- Curie. The Curie is a non-SI unit of radioactivity defined in 1910. It was originally defined as equivalent to the number of disintegrations that one gram of radium-226 will undergo in one second. Currently, a curie is defined as 1Ci = 3.7 x 1010 disintegrations per second.
- Rutherford. The Rutherford (symbol Rd) is also a non-SI unit defined as the activity of a quantity of radioactive material in which one million nuclei decay per second.
Decay Constant and Half-Life
In radioactivity calculations, one of two parameters (decay constant or half-life), which characterize the rate of decay, must be known. There is a relation between the half-life (t1/2) and the decay constant λ. The relationship can be derived from decay law by setting N = ½ No. This gives:
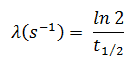where ln 2 (the natural log of 2) equals 0.693. If the decay constant (λ) is given, it is easy to calculate the half-life, and vice-versa.
Bateman Equations
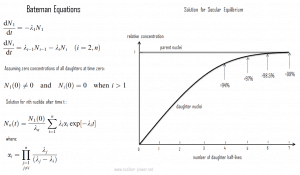In physics, the Bateman equations are a set of first-order differential equations, which describe the time evolution of nuclide concentrations undergoing serial or linear decay chains. Ernest Rutherford formulated the model in 1905, and the analytical solution for the case of radioactive decay in a linear chain was provided by Harry Bateman in 1910. This model can also be used in nuclear depletion codes to solve nuclear transmutation and decay problems.
For example, ORIGEN is a computer code system for calculating the buildup, decay, and processing of radioactive materials. ORIGEN uses a matrix exponential method to solve a large system of coupled, linear, first-order ordinary differential equations (similar to the Bateman equations) with constant coefficients.
The Bateman equations for radioactive decay case of n – nuclide series in linear chain describing nuclide concentrations are as follows shown in the figure.
Example – Radioactive Decay Law
A sample of material contains 1 microgram of iodine-131. Note that iodine-131 plays a major role as a radioactive isotope present in nuclear fission products and contributes to health hazards when released into the atmosphere during an accident. Iodine-131 has a half-life of 8.02 days.
Calculate:
- The number of iodine-131 atoms is initially present.
- The activity of the iodine-131 in curies.
- The number of iodine-131 atoms will remain in 50 days.
- The time it will take for the activity to reach 0.1 mCi.
Solution:
- The number of atoms of iodine-131 can be determined using isotopic mass as below.
NI-131 = mI-131 . NA / MI-131
NI-131 = (1 μg) x (6.02×1023 nuclei/mol) / (130.91 g/mol)
NI-131 = 4.6 x 1015 nuclei
- The activity of the iodine-131 in curies can be determined using its decay constant:
The iodine-131 has a half-life of 8.02 days (692928 sec), and therefore its decay constant is:
Using this value for the decay constant, we can determine the activity of the sample:
3) and 4) The number of iodine-131 atoms that will remain in 50 days (N50d) and the time it will take for the activity to reach 0.1 mCi can be calculated using the decay law:
As can be seen, after 50 days, the number of iodine-131 atoms and thus the activity will be about 75 times lower. After 82 days, the activity will be approximately 1200 times lower. Therefore, the time of ten half-lives (factor 210 = 1024) is widely used to define residual activity.
